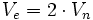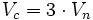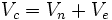Plantilla:Áreas y volúmenes en el espacio
De Wikipedia
| Revisión de 11:00 15 ene 2009 Coordinador (Discusión | contribuciones) ← Ir a diferencia anterior |
Revisión de 06:56 18 may 2010 Coordinador (Discusión | contribuciones) Ir a siguiente diferencia → |
||
| Línea 25: | Línea 25: | ||
| <center><iframe> | <center><iframe> | ||
| - | url=http://maralboran.ath.cx/web_ma/descartes/1y2_eso/Areas_2eso_d3/areascg_3_2.html | + | url=http://maralboran.org/web_ma/descartes/1y2_eso/Areas_2eso_d3/areascg_3_2.html |
| width=500 | width=500 | ||
| height=420 | height=420 | ||
| name=myframe | name=myframe | ||
| </iframe></center> | </iframe></center> | ||
| - | <center>[http://maralboran.ath.cx/web_ma/descartes/1y2_eso/Areas_2eso_d3/areascg_3_2.html '''Shift-Click''' aquí si no se ve bien la escena]</center> | + | <center>[http://maralboran.org/web_ma/descartes/1y2_eso/Areas_2eso_d3/areascg_3_2.html '''Shift-Click''' aquí si no se ve bien la escena]</center> |
| }} | }} | ||
| {{ai_cuerpo | {{ai_cuerpo | ||
| Línea 39: | Línea 39: | ||
| <center><iframe> | <center><iframe> | ||
| - | url=http://maralboran.ath.cx/web_ma/descartes/1y2_eso/Volumenes_d3/VOLUMENES_2_2.html | + | url=http://maralboran.org/web_ma/descartes/1y2_eso/Volumenes_d3/VOLUMENES_2_2.html |
| width=530 | width=530 | ||
| height=420 | height=420 | ||
| name=myframe | name=myframe | ||
| </iframe></center> | </iframe></center> | ||
| - | <center>[http://maralboran.ath.cx/web_ma/descartes/1y2_eso/Volumenes_d3/VOLUMENES_2_2.html '''Shift-Click''' aquí si no se ve bien la escena]</center> | + | <center>[http://maralboran.org/web_ma/descartes/1y2_eso/Volumenes_d3/VOLUMENES_2_2.html '''Shift-Click''' aquí si no se ve bien la escena]</center> |
| }} | }} | ||
| Línea 90: | Línea 90: | ||
| <center><iframe> | <center><iframe> | ||
| - | url=http://maralboran.ath.cx/web_ma/descartes/1y2_eso/Areas_2eso_d3/areascg_2_1.html | + | url=http://maralboran.org/web_ma/descartes/1y2_eso/Areas_2eso_d3/areascg_2_1.html |
| width=500 | width=500 | ||
| height=700 | height=700 | ||
| name=myframe | name=myframe | ||
| </iframe></center> | </iframe></center> | ||
| - | <center>[http://maralboran.ath.cx/web_ma/descartes/1y2_eso/Areas_2eso_d3/areascg_2_1.html '''Shift-Click''' aquí si no se ve bien la escena]</center> | + | <center>[http://maralboran.org/web_ma/descartes/1y2_eso/Areas_2eso_d3/areascg_2_1.html '''Shift-Click''' aquí si no se ve bien la escena]</center> |
| }} | }} | ||
| {{ai_cuerpo | {{ai_cuerpo | ||
| Línea 104: | Línea 104: | ||
| <center><iframe> | <center><iframe> | ||
| - | url=http://maralboran.ath.cx/web_ma/descartes/1y2_eso/Volumenes_d3/VOLUMENES_1_1.html | + | url=http://maralboran.org/web_ma/descartes/1y2_eso/Volumenes_d3/VOLUMENES_1_1.html |
| width=530 | width=530 | ||
| height=330 | height=330 | ||
| name=myframe | name=myframe | ||
| </iframe></center> | </iframe></center> | ||
| - | <center>[http://maralboran.ath.cx/web_ma/descartes/1y2_eso/Volumenes_d3/VOLUMENES_1_1.html '''Shift-Click''' aquí si no se ve bien la escena]</center> | + | <center>[http://maralboran.org/web_ma/descartes/1y2_eso/Volumenes_d3/VOLUMENES_1_1.html '''Shift-Click''' aquí si no se ve bien la escena]</center> |
| }} | }} | ||
| {{ai_cuerpo | {{ai_cuerpo | ||
| Línea 118: | Línea 118: | ||
| <center><iframe> | <center><iframe> | ||
| - | url=http://maralboran.ath.cx/web_ma/descartes/1y2_eso/Volumenes_d3/VOLUMENES_2_1.html | + | url=http://maralboran.org/web_ma/descartes/1y2_eso/Volumenes_d3/VOLUMENES_2_1.html |
| width=530 | width=530 | ||
| height=390 | height=390 | ||
| name=myframe | name=myframe | ||
| </iframe></center> | </iframe></center> | ||
| - | <center>[http://maralboran.ath.cx/web_ma/descartes/1y2_eso/Volumenes_d3/VOLUMENES_2_1.html '''Shift-Click''' aquí si no se ve bien la escena]</center> | + | <center>[http://maralboran.org/web_ma/descartes/1y2_eso/Volumenes_d3/VOLUMENES_2_1.html '''Shift-Click''' aquí si no se ve bien la escena]</center> |
| Línea 157: | Línea 157: | ||
| <center><iframe> | <center><iframe> | ||
| - | url=http://maralboran.ath.cx/web_ma/descartes/1y2_eso/Volumenes_d3/VOLUMENES_4_1.html | + | url=http://maralboran.org/web_ma/descartes/1y2_eso/Volumenes_d3/VOLUMENES_4_1.html |
| width=530 | width=530 | ||
| height=390 | height=390 | ||
| name=myframe | name=myframe | ||
| </iframe></center> | </iframe></center> | ||
| - | <center>[http://maralboran.ath.cx/web_ma/descartes/1y2_eso/Volumenes_d3/VOLUMENES_4_1.html '''Shift-Click''' aquí si no se ve bien la escena]</center> | + | <center>[http://maralboran.org/web_ma/descartes/1y2_eso/Volumenes_d3/VOLUMENES_4_1.html '''Shift-Click''' aquí si no se ve bien la escena]</center> |
| Ahora mueve el deslizador de la parte superior y explica lo que ocurre y el porqué. | Ahora mueve el deslizador de la parte superior y explica lo que ocurre y el porqué. | ||
| Línea 196: | Línea 196: | ||
| <center><iframe> | <center><iframe> | ||
| - | url=http://maralboran.ath.cx/web_ma/descartes/1y2_eso/Volumenes_d3/VOLUMENES_9_1.html | + | url=http://maralboran.org/web_ma/descartes/1y2_eso/Volumenes_d3/VOLUMENES_9_1.html |
| width=840 | width=840 | ||
| height=490 | height=490 | ||
| name=myframe | name=myframe | ||
| </iframe></center> | </iframe></center> | ||
| - | <center>[http://maralboran.ath.cx/web_ma/descartes/1y2_eso/Volumenes_d3/VOLUMENES_9_1.html '''Shift-Click''' aquí si no se ve bien la escena]</center> | + | <center>[http://maralboran.org/web_ma/descartes/1y2_eso/Volumenes_d3/VOLUMENES_9_1.html '''Shift-Click''' aquí si no se ve bien la escena]</center> |
| }} | }} | ||
| }} | }} | ||
| Línea 233: | Línea 233: | ||
| <center><iframe> | <center><iframe> | ||
| - | url=http://maralboran.ath.cx/web_ma/descartes/1y2_eso/Volumenes_d3/VOLUMENES_3_1.html | + | url=http://maralboran.org/web_ma/descartes/1y2_eso/Volumenes_d3/VOLUMENES_3_1.html |
| width=530 | width=530 | ||
| height=390 | height=390 | ||
| name=myframe | name=myframe | ||
| </iframe></center> | </iframe></center> | ||
| - | <center>[http://maralboran.ath.cx/web_ma/descartes/1y2_eso/Volumenes_d3/VOLUMENES_3_1.html '''Shift-Click''' aquí si no se ve bien la escena]</center> | + | <center>[http://maralboran.org/web_ma/descartes/1y2_eso/Volumenes_d3/VOLUMENES_3_1.html '''Shift-Click''' aquí si no se ve bien la escena]</center> |
| }} | }} | ||
| }} | }} | ||
| Línea 272: | Línea 272: | ||
| <center><iframe> | <center><iframe> | ||
| - | url=http://maralboran.ath.cx/web_ma/descartes/1y2_eso/Volumenes_d3/VOLUMENES_4_2.html | + | url=http://maralboran.org/web_ma/descartes/1y2_eso/Volumenes_d3/VOLUMENES_4_2.html |
| width=530 | width=530 | ||
| height=390 | height=390 | ||
| name=myframe | name=myframe | ||
| </iframe></center> | </iframe></center> | ||
| - | <center>[http://maralboran.ath.cx/web_ma/descartes/1y2_eso/Volumenes_d3/VOLUMENES_4_2.html '''Shift-Click''' aquí si no se ve bien la escena]</center> | + | <center>[http://maralboran.org/web_ma/descartes/1y2_eso/Volumenes_d3/VOLUMENES_4_2.html '''Shift-Click''' aquí si no se ve bien la escena]</center> |
| Ahora mueve el deslizador de la parte superior y explica lo que ocurre y el porqué. | Ahora mueve el deslizador de la parte superior y explica lo que ocurre y el porqué. | ||
| Línea 309: | Línea 309: | ||
| <center><iframe> | <center><iframe> | ||
| - | url=http://maralboran.ath.cx/web_ma/descartes/1y2_eso/Volumenes_d3/VOLUMENES_9_1.html | + | url=http://maralboran.org/web_ma/descartes/1y2_eso/Volumenes_d3/VOLUMENES_9_1.html |
| width=840 | width=840 | ||
| height=490 | height=490 | ||
| name=myframe | name=myframe | ||
| </iframe></center> | </iframe></center> | ||
| - | <center>[http://maralboran.ath.cx/web_ma/descartes/1y2_eso/Volumenes_d3/VOLUMENES_9_1.html '''Shift-Click''' aquí si no se ve bien la escena]</center> | + | <center>[http://maralboran.org/web_ma/descartes/1y2_eso/Volumenes_d3/VOLUMENES_9_1.html '''Shift-Click''' aquí si no se ve bien la escena]</center> |
| }} | }} | ||
| }} | }} | ||
| Línea 341: | Línea 341: | ||
| <center><iframe> | <center><iframe> | ||
| - | url=http://maralboran.ath.cx/web_ma/descartes/1y2_eso/Volumenes_d3/VOLUMENES_6_1.html | + | url=http://maralboran.org/web_ma/descartes/1y2_eso/Volumenes_d3/VOLUMENES_6_1.html |
| width=530 | width=530 | ||
| height=390 | height=390 | ||
| name=myframe | name=myframe | ||
| </iframe></center> | </iframe></center> | ||
| - | <center>[http://maralboran.ath.cx/web_ma/descartes/1y2_eso/Volumenes_d3/VOLUMENES_6_1.html '''Shift-Click''' aquí si no se ve bien la escena]</center> | + | <center>[http://maralboran.org/web_ma/descartes/1y2_eso/Volumenes_d3/VOLUMENES_6_1.html '''Shift-Click''' aquí si no se ve bien la escena]</center> |
| }} | }} | ||
| {{ai_cuerpo | {{ai_cuerpo | ||
| Línea 355: | Línea 355: | ||
| <center><iframe> | <center><iframe> | ||
| - | url=http://maralboran.ath.cx/web_ma/descartes/1y2_eso/Volumenes_d3/VOLUMENES_6_2.html | + | url=http://maralboran.org/web_ma/descartes/1y2_eso/Volumenes_d3/VOLUMENES_6_2.html |
| width=530 | width=530 | ||
| height=390 | height=390 | ||
| name=myframe | name=myframe | ||
| </iframe></center> | </iframe></center> | ||
| - | <center>[http://maralboran.ath.cx/web_ma/descartes/1y2_eso/Volumenes_d3/VOLUMENES_6_2.html '''Shift-Click''' aquí si no se ve bien la escena]</center> | + | <center>[http://maralboran.org/web_ma/descartes/1y2_eso/Volumenes_d3/VOLUMENES_6_2.html '''Shift-Click''' aquí si no se ve bien la escena]</center> |
| En consecuencia,si llamamos: | En consecuencia,si llamamos: | ||
| Línea 390: | Línea 390: | ||
| <center><iframe> | <center><iframe> | ||
| - | url=http://maralboran.ath.cx/web_ma/descartes/1y2_eso/Volumenes_d3/VOLUMENES_6_3.html | + | url=http://maralboran.org/web_ma/descartes/1y2_eso/Volumenes_d3/VOLUMENES_6_3.html |
| width=530 | width=530 | ||
| height=350 | height=350 | ||
| name=myframe | name=myframe | ||
| </iframe></center> | </iframe></center> | ||
| - | <center>[http://maralboran.ath.cx/web_ma/descartes/1y2_eso/Volumenes_d3/VOLUMENES_6_3.html '''Shift-Click''' aquí si no se ve bien la escena]</center> | + | <center>[http://maralboran.org/web_ma/descartes/1y2_eso/Volumenes_d3/VOLUMENES_6_3.html '''Shift-Click''' aquí si no se ve bien la escena]</center> |
| }} | }} | ||
| Línea 409: | Línea 409: | ||
| {{p}} | {{p}} | ||
| <center><iframe> | <center><iframe> | ||
| - | url=http://maralboran.ath.cx/web_ma/descartes/1y2_eso/Volumenes_d3/VOLUMENES_8_1.html | + | url=http://maralboran.org/web_ma/descartes/1y2_eso/Volumenes_d3/VOLUMENES_8_1.html |
| width=500 | width=500 | ||
| height=390 | height=390 | ||
| name=myframe | name=myframe | ||
| </iframe></center> | </iframe></center> | ||
| - | <center>[http://maralboran.ath.cx/web_ma/descartes/1y2_eso/Volumenes_d3/VOLUMENES_8_1.html '''Shift-Click''' aquí si no se ve bien la escena]</center> | + | <center>[http://maralboran.org/web_ma/descartes/1y2_eso/Volumenes_d3/VOLUMENES_8_1.html '''Shift-Click''' aquí si no se ve bien la escena]</center> |
| }} | }} | ||
| {{ai_cuerpo | {{ai_cuerpo | ||
| Línea 422: | Línea 422: | ||
| {{p}} | {{p}} | ||
| <center><iframe> | <center><iframe> | ||
| - | url=http://maralboran.ath.cx/web_ma/descartes/1y2_eso/Volumenes_d3/VOLUMENES_8_2.html | + | url=http://maralboran.org/web_ma/descartes/1y2_eso/Volumenes_d3/VOLUMENES_8_2.html |
| width=500 | width=500 | ||
| height=390 | height=390 | ||
| name=myframe | name=myframe | ||
| </iframe></center> | </iframe></center> | ||
| - | <center>[http://maralboran.ath.cx/web_ma/descartes/1y2_eso/Volumenes_d3/VOLUMENES_8_2.html '''Shift-Click''' aquí si no se ve bien la escena]</center> | + | <center>[http://maralboran.org/web_ma/descartes/1y2_eso/Volumenes_d3/VOLUMENES_8_2.html '''Shift-Click''' aquí si no se ve bien la escena]</center> |
| }} | }} | ||
| }} | }} | ||
Revisión de 06:56 18 may 2010
Tabla de contenidos |
Prisma
|
|
|
Actividad Interactiva: Area y volumen del prisma
Actividad 1: Halla el área lateral y total de un prisma recto de base un triángulo equilátero, de 1 cm de lado y 2 cm de altura.
Actividad: Comprueba los resultados en la siguiente escena:
Actividad 2: Halla el volumen de un prisma recto de base cuadrada, de lado 1,5 cm y altura 2,2 cm...
Actividad: Comprueba los resultados en la siguiente escena: |
Ortoedro
Como sabemos, un ortoedro es un prisma recto de base rectangular o cuadrada.
|
|
Cubo
Un caso particular de ortoedro es el cubo cuyas caras son todas cuadradas.
|
|
|
Actividad Interactiva: Area y volumen del ortoedro y cubo
Actividad 1: Halla el área lateral y total de un ortoedro de aristas 2, 3 y 1,5 cm.
Actividad: Comprueba los resultados en la siguiente escena:
Actividad 2: Halla el volumen de un cubo de 3 cm de arista.
Actividad: Comprueba los resultados en la siguiente escena:
Actividad 3: Halla el volumen de un ortoedro de 3 cm de largo, 2 cm de ancho y 2,5 cm de alto.
Actividad: Comprueba los resultados en la siguiente escena: |
Pirámide
|
|
|
Actividad Interactiva: Volumen de la pirámide
Actividad 1: Halla el volumen de una pirámide cuya base es un triángulo equilátero, con 3 cm de arista básica y 5 cm de altura.
Actividad: Comprueba los resultados en la siguiente escena: Ahora mueve el deslizador de la parte superior y explica lo que ocurre y el porqué. |
Pirámide truncada
|
|
|
Actividad Interactiva: Tronco de pirámide
Actividad 1: Halla el volumen de un tronco de pirámide cuadrada que tiene una arista básica mayor de 3 cm, una arista básica menor de 2 cm y una altura de 1,7 cm. Halla también su superficie.
Actividad: Comprueba los resultados en la siguiente escena: |
Cilindro
|
|
|
Actividad Interactiva: Volumen del cilindro
Actividad 1: Halla el volumen de un cilindro de 3 cm de radio y 2 cm de altura.
Actividad: Comprueba los resultados en la siguiente escena: |
Cono
|
|
|
Actividad Interactiva: Volumen del cono
Actividad 1: Halla el volumen de un cono de 2 cm de radio y 3 cm de altura.
Actividad: Comprueba los resultados en la siguiente escena: Ahora mueve el deslizador de la parte superior y explica lo que ocurre y el porqué. |
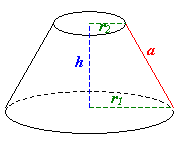
Cono truncado
|
|
|
Actividad Interactiva: Tronco de cono
Actividad 1: Halla el volumen de un tronco de cono que tiene un radio básico mayor de 4,2 cm, un radio básico menor de 2 cm y una altura de 2 cm. Halla también su superficie.
Actividad: Comprueba los resultados en la siguiente escena: |
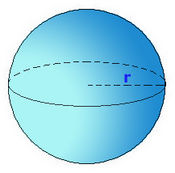
Esfera
|
|
|
Actividades Interactivas: Volumen de la esfera
Actividad 1: Halla el volumen de una esfera de 2,1 cm de radio.
Actividad: Comprueba los resultados en la siguiente escena:
Actividad 2: ¿Qué relación existe entre el volumen de la esfera y los volúmenes del cilindro y del cono?
Actividad: En la siguiente escena modifica los valores del radio y observa los resultados: En consecuencia,si llamamos:
entonces:
Actividad 3: Halla el volumen y la superficie de un balón de futbol.
Actividad: Balón Fútbol Competición, MASTER T-5: Balón técnico para competición. Cubierta de cuero PU Cordley de 32 paneles cosidos. Fabricado bajo norma ISO9002 de acuerdo a las especificaciones FIFA. Sus cuatro capas de poliéster garantizan estabilidad y evitan la deformación del balón. Cámara de látex de doble laminado y válvula de Butyl automática. Datos técnicos:
|
Ejercicios
|
Actividad Interactiva: Volúmenes de cuerpos irregulares
Actividad 1. Halla el volumen de las siguientes figuras.
Actividad: Halla el volumen de las figuras que aparecen en la siguiente escena. Pulsa las flechas para cambiar la figura.
Actividad 2. Halla el volumen de las siguientes figuras.
Actividad: Halla el volumen de las figuras que aparecen en la siguiente escena. Pulsa las flechas para cambiar la figura. |
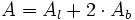
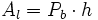
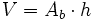
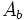 : Área de la base.
: Área de la base.
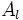 : Área lateral.
: Área lateral.
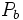 : Perímetro de la base.
: Perímetro de la base.
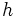 : altura.
: altura.
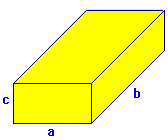

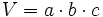
 : aristas.
: aristas.
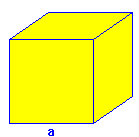
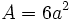
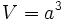
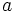 : arista.
: arista.

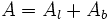
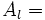 Suma áreas triángulos
Suma áreas triángulos
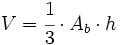
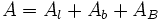
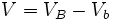
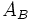 : Área de la base inferior.
: Área de la base inferior.
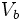 : Volumen de la pirámide pequeña de base b.
: Volumen de la pirámide pequeña de base b.
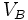 : Volumen de la pirámide completa de base B.
: Volumen de la pirámide completa de base B.

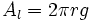

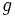 : generatriz.
: generatriz.
 : radio.
: radio.

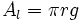
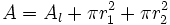
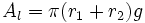
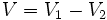
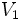 : Volumen del cono completo.
: Volumen del cono completo.
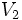 : Volumen del cono pequeño eliminado.
: Volumen del cono pequeño eliminado.
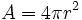
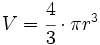
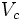 = Volumen del cilindro de radio R y altura 2R
= Volumen del cilindro de radio R y altura 2R
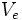 = Volumen de la esfera de radio R.
= Volumen de la esfera de radio R.
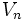 = Volumen del cono de radio R y altura 2R.
= Volumen del cono de radio R y altura 2R.