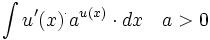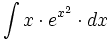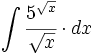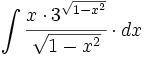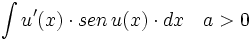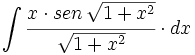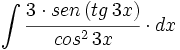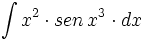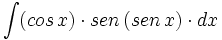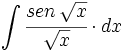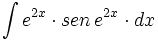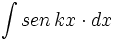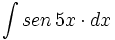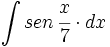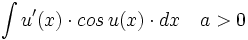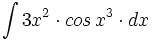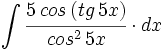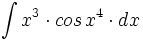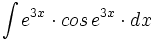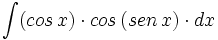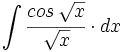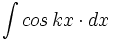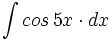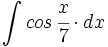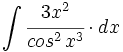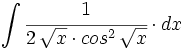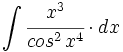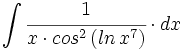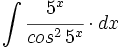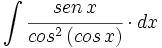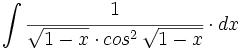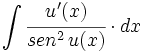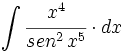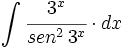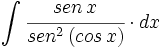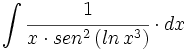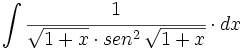Cálculo de primitivas inmediatas (2ºBach)
De Wikipedia
(Diferencia entre revisiones)
| Revisión de 05:28 26 jun 2017 Coordinador (Discusión | contribuciones) ← Ir a diferencia anterior |
Revisión de 05:37 26 jun 2017 Coordinador (Discusión | contribuciones) Ir a siguiente diferencia → |
||
| Línea 20: | Línea 20: | ||
| |titulo1=Ejercicio 1 | |titulo1=Ejercicio 1 | ||
| |duracion=7'01" | |duracion=7'01" | ||
| - | |sinopsis=Primitivas del tipo <math>\int [u(x)]^m \cdot u'(x) \cdot dx \quad (m \ne -1)</math>: | + | |sinopsis= |
| - | + | ||
| #<math>\int 4x^3(1+x^4)^{20} \cdot dx</math> | #<math>\int 4x^3(1+x^4)^{20} \cdot dx</math> | ||
| #<math>\int 3(3x+5)^7 \cdot dx</math> | #<math>\int 3(3x+5)^7 \cdot dx</math> | ||
| Línea 57: | Línea 56: | ||
| |titulo1=Ejercicio 4 | |titulo1=Ejercicio 4 | ||
| |duracion=6'27" | |duracion=6'27" | ||
| - | |sinopsis=Primitivas del tipo <math>\int [u(x)]^m \cdot u'(x) \cdot dx \quad (m \ne -1)</math>: | + | |sinopsis= |
| - | + | ||
| #<math>\int e^x(1+e^x)^3 \cdot dx</math> | #<math>\int e^x(1+e^x)^3 \cdot dx</math> | ||
| #<math>\int 6x^5(1+x^6)^{10} \cdot dx</math> | #<math>\int 6x^5(1+x^6)^{10} \cdot dx</math> | ||
| Línea 70: | Línea 68: | ||
| |titulo1=Ejercicio 5 | |titulo1=Ejercicio 5 | ||
| |duracion=13'31" | |duracion=13'31" | ||
| - | |sinopsis=Primitivas del tipo <math>\int [u(x)]^m \cdot u'(x) \cdot dx \quad (m \ne -1)</math>, pero que no son del todo inmediatas: | + | |sinopsis= |
| - | + | ||
| #<math>\int x(7+x^2)^5 \cdot dx</math> | #<math>\int x(7+x^2)^5 \cdot dx</math> | ||
| #<math>\int e^{3x}(2+e^{3x})^4 \cdot dx</math> | #<math>\int e^{3x}(2+e^{3x})^4 \cdot dx</math> | ||
| Línea 81: | Línea 78: | ||
| |titulo1=Ejercicio 6 | |titulo1=Ejercicio 6 | ||
| |duracion=13'31" | |duracion=13'31" | ||
| - | |sinopsis=Primitivas del tipo <math>\int [u(x)]^m \cdot u'(x) \cdot dx \quad (m \ne -1)</math>, pero que no son del todo inmediatas: | + | |sinopsis= |
| - | + | ||
| #<math>\int \cfrac{1}{x\sqrt[4]{1-ln \, x}} \cdot dx</math> | #<math>\int \cfrac{1}{x\sqrt[4]{1-ln \, x}} \cdot dx</math> | ||
| #<math>\int \cfrac{sen \, 3x}{\sqrt[5]{1-cos \, 3x}} \cdot dx</math> | #<math>\int \cfrac{sen \, 3x}{\sqrt[5]{1-cos \, 3x}} \cdot dx</math> | ||
| Línea 96: | Línea 92: | ||
| |titulo1=Ejercicio 7 | |titulo1=Ejercicio 7 | ||
| |duracion=5'47" | |duracion=5'47" | ||
| - | |sinopsis=Primitivas del tipo <math>\int [u(x)]^m \cdot u'(x) \cdot dx \quad (m \ne -1)</math>, pero que no son del todo inmediatas: | + | |sinopsis= |
| - | + | ||
| #<math>\int 4^x \sqrt{1+4^x} \cdot dx</math> | #<math>\int 4^x \sqrt{1+4^x} \cdot dx</math> | ||
| #<math>\int \cfrac{\sqrt{1+tg \, 2x}}{cos^2 \, 2x} \cdot dx</math> | #<math>\int \cfrac{\sqrt{1+tg \, 2x}}{cos^2 \, 2x} \cdot dx</math> | ||
| Línea 114: | Línea 109: | ||
| |titulo1=Ejercicio 1 | |titulo1=Ejercicio 1 | ||
| |duracion=4'26" | |duracion=4'26" | ||
| - | |sinopsis=Primitivas del tipo <math>\int \cfrac{u'(x)}{u(x)} \cdot dx</math>: | + | |sinopsis= |
| - | + | ||
| #<math>\int \cfrac{3}{(5+tg \,3x)\cdot cos^2 \,3x} \cdot dx</math> | #<math>\int \cfrac{3}{(5+tg \,3x)\cdot cos^2 \,3x} \cdot dx</math> | ||
| #<math>\int \cfrac{cos \, 3x}{1-sen \, 3x} \cdot dx</math> | #<math>\int \cfrac{cos \, 3x}{1-sen \, 3x} \cdot dx</math> | ||
| Línea 126: | Línea 120: | ||
| |titulo1=Ejercicio 2 | |titulo1=Ejercicio 2 | ||
| |duracion=2'36" | |duracion=2'36" | ||
| - | |sinopsis=Primitivas del tipo <math>\int \cfrac{u'(x)}{u(x)} \cdot dx</math>: | + | |sinopsis= |
| - | + | ||
| #<math>\int \cfrac{1}{\sqrt{x} \cdot (1+\sqrt{x})} \cdot dx</math> | #<math>\int \cfrac{1}{\sqrt{x} \cdot (1+\sqrt{x})} \cdot dx</math> | ||
| #<math>\int \cfrac{1}{\sqrt[3]{x} \cdot (1+\sqrt[3]{x^2})} \cdot dx</math> | #<math>\int \cfrac{1}{\sqrt[3]{x} \cdot (1+\sqrt[3]{x^2})} \cdot dx</math> | ||
| Línea 143: | Línea 136: | ||
| |titulo1=Ejercicio 1 | |titulo1=Ejercicio 1 | ||
| |duracion=10'25" | |duracion=10'25" | ||
| - | |sinopsis=Primitivas del tipo <math>\int u'(x)^\cdot a^{u(x)} \cdot dx \quad a>0</math>: | + | |sinopsis= |
| - | + | ||
| #<math>\int 5x^4 \cdot 7^{x^5} \cdot dx</math> | #<math>\int 5x^4 \cdot 7^{x^5} \cdot dx</math> | ||
| #<math>\int 4x^3 e^{x^4} \cdot dx</math> | #<math>\int 4x^3 e^{x^4} \cdot dx</math> | ||
| Línea 168: | Línea 160: | ||
| |titulo1=Ejercicio 1 | |titulo1=Ejercicio 1 | ||
| |duracion=6'33" | |duracion=6'33" | ||
| - | |sinopsis=Primitivas del tipo <math>\int u'(x) \cdot sen \, u(x) \cdot dx \quad a>0</math>: | + | |sinopsis= |
| - | + | ||
| #<math>\int \cfrac{sen \, (ln \, x)}{x} \cdot dx</math> | #<math>\int \cfrac{sen \, (ln \, x)}{x} \cdot dx</math> | ||
| #<math>\int \cfrac{x \cdot sen \, \sqrt{1+x^2}}{\sqrt{1+x^2}} \cdot dx</math> | #<math>\int \cfrac{x \cdot sen \, \sqrt{1+x^2}}{\sqrt{1+x^2}} \cdot dx</math> | ||
| Línea 191: | Línea 182: | ||
| |titulo1=Ejercicio 1 | |titulo1=Ejercicio 1 | ||
| |duracion=7'28" | |duracion=7'28" | ||
| - | |sinopsis=Primitivas del tipo <math>\int u'(x) \cdot cos \, u(x) \cdot dx \quad a>0</math>: | + | |sinopsis= |
| - | + | ||
| #<math>\int 3x^2 \cdot cos \, x^3 \cdot dx</math> | #<math>\int 3x^2 \cdot cos \, x^3 \cdot dx</math> | ||
| #<math>\int \cfrac{3x^2 \cdot cos \, \sqrt{1+x^3}}{2 \, \sqrt{1+x^3}} \cdot dx</math> | #<math>\int \cfrac{3x^2 \cdot cos \, \sqrt{1+x^3}}{2 \, \sqrt{1+x^3}} \cdot dx</math> | ||
| Línea 215: | Línea 205: | ||
| |titulo1=Ejercicio 1 | |titulo1=Ejercicio 1 | ||
| |duracion=6'39" | |duracion=6'39" | ||
| - | |sinopsis=Primitivas del tipo <math>\int \cfrac{u'(x)}{cos^2 \, u(x)} \cdot dx</math>: | + | |sinopsis= |
| - | + | ||
| #<math>\int \cfrac{3x^2}{cos^2 \, x^3} \cdot dx</math> | #<math>\int \cfrac{3x^2}{cos^2 \, x^3} \cdot dx</math> | ||
| #<math>\int \cfrac{3x^2}{2 \, \sqrt{1+x^3} \cdot cos^2 \, \sqrt{1+x^3}} \cdot dx</math> | #<math>\int \cfrac{3x^2}{2 \, \sqrt{1+x^3} \cdot cos^2 \, \sqrt{1+x^3}} \cdot dx</math> | ||
| Línea 232: | Línea 221: | ||
| |titulo=Ejercicios resueltos: ''Primitivas inmediatas'' | |titulo=Ejercicios resueltos: ''Primitivas inmediatas'' | ||
| |enunciado=Primitivas del tipo <math>\int \cfrac{u'(x)}{sen^2 \, u(x)} \cdot dx</math> | |enunciado=Primitivas del tipo <math>\int \cfrac{u'(x)}{sen^2 \, u(x)} \cdot dx</math> | ||
| - | {{Videotutoriales|titulo=Ejercicios|enunciado= | + | {{Video_enlace_fonemato |
| - | {{Video_enlace2 | + | |titulo1=Ejercicio 1 |
| - | |titulo1=1. Ejemplo | + | |duracion=5'24" |
| - | |duracion=3'01" | + | |
| - | |sinopsis= | + | |
| - | |url1=http://www.matematicasbachiller.com/videos/cintegral/01/in01_05g1_media/in01_05g1.wmv | + | |
| - | }} | + | |
| - | {{Video_enlace2 | + | |
| - | |titulo1=2. Ejemplo | + | |
| - | |duracion=4'12" | + | |
| |sinopsis= | |sinopsis= | ||
| - | |url1=http://www.matematicasbachiller.com/videos/cintegral/01/in01_05g2_media/in01_05g2.wmv | + | #<math>\int \cfrac{1}{2 \, \sqrt{x} \cdot sen^2 \, \sqrt{x}} \cdot dx</math> |
| + | #<math>\int \cfrac{x^4}{sen^2 \, x^5} \cdot dx</math> | ||
| + | #<math>\int \cfrac{3^x}{sen^2 \, 3^x} \cdot dx</math> | ||
| + | #<math>\int \cfrac{sen \, x}{sen^2 \, (cos \, x)} \cdot dx</math> | ||
| + | #<math>\int \cfrac{1}{x \cdot sen^2 \, (ln \, x^3)} \cdot dx</math> | ||
| + | #<math>\int \cfrac{1}{\sqrt{1+x} \cdot sen^2 \, \sqrt{1+x}} \cdot dx</math> | ||
| + | |url1=https://www.youtube.com/watch?v=05mXNQAehUI&index=20&list=PLECA0C7A8B59E5534 | ||
| }} | }} | ||
| }} | }} | ||
Revisión de 05:37 26 jun 2017
Menú:
| Enlaces internos | Para repasar o ampliar | Enlaces externos |
| Indice Descartes Manual Casio | WIRIS Calculadora |
Ejercicios resueltos: Primitivas inmediatas
Primitivas del tipo ![\int [u(x)]^m \cdot u'(x) \cdot dx \quad (m \ne -1)](/wikipedia/images/math/d/0/b/d0b6088fa26b6d625de05c0a40b70602.png)
- Primitivas del tipo
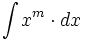
 . Ejemplos
. Ejemplos
- Ejercicios:
- Primitivas del tipo
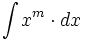
 en las que hay que aplicar el binomio de Newton.
en las que hay que aplicar el binomio de Newton.
- Ejercicios:
Ejercicios resueltos: Primitivas inmediatas
}}
Ejercicios resueltos: Primitivas inmediatas
Primitivas del tipo ![\int \cfrac{u'(x)}{a^2+[u(x)]^2} \cdot dx](/wikipedia/images/math/1/2/1/1216aad7e7c8189456878755ca0c846c.png)
Ejercicios resueltos: Primitivas inmediatas
Primitivas del tipo ![\int \cfrac{u'(x)}{\sqrt{a^2-[u(x)]^2}} \cdot dx](/wikipedia/images/math/7/0/e/70e26d7ca7ea8762b35e39fea301f6a4.png)
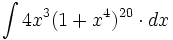
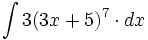
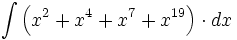

![\int \left( \sqrt{x} + \sqrt[3]{x} + \sqrt[6]{x} + \sqrt[8]{x}\right) \cdot dx](/wikipedia/images/math/a/d/7/ad7b72d3dc41c911a4cf21a1642eeead.png)
![\int \left( \cfrac{1}{\sqrt[5]{x}} + \cfrac{1}{\sqrt[7]{x}} + \cfrac{1}{\sqrt[9]{x}}\right) \cdot dx](/wikipedia/images/math/6/4/7/6472b81d1d67dc15b37e43230a2dd291.png)
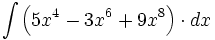
![\int \left( 5 -3\sqrt{x}+9\sqrt[5]{x} \right) \cdot dx](/wikipedia/images/math/8/a/9/8a9fe9ee2f33c419cf96dc0ee568039f.png)
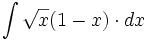
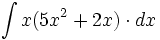

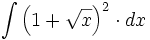
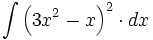
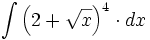
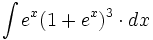
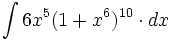
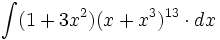
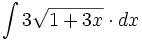
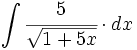
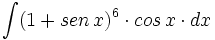
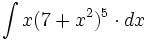
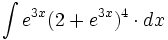
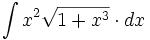

![\int \cfrac{1}{x\sqrt[4]{1-ln \, x}} \cdot dx](/wikipedia/images/math/c/f/c/cfc07daf2baefd842e5848f0f7e03f42.png)
![\int \cfrac{sen \, 3x}{\sqrt[5]{1-cos \, 3x}} \cdot dx](/wikipedia/images/math/f/e/d/fedd75fa6e1b4fc832a1b3244d31d706.png)
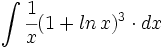
![\int \cfrac{7}{\sqrt[3]{1+7x}} \cdot dx](/wikipedia/images/math/3/e/c/3ec4553d16d4a020caac805f5621e032.png)
![\int 2\sqrt[3]{1+2x} \cdot dx](/wikipedia/images/math/9/1/9/919dbc3b549d0ed508a6e32d4f1eb63f.png)

![\int \cfrac{cos \, x}{\sqrt[3]{1+sen \, x}} \cdot dx](/wikipedia/images/math/7/1/d/71d9e7ee213b836b85f2bed8530bad51.png)
![\int \cfrac{1}{x\sqrt[4]{1+ln \, x}} \cdot dx](/wikipedia/images/math/3/6/e/36e712c3e51f2f00db9567790cce7502.png)
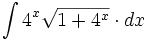
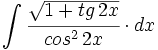

![\int \cfrac{\sqrt[4]{1+cotg \, 3x}}{sen^2 \, 3x} \cdot dx](/wikipedia/images/math/8/f/2/8f234b8b662cc63a891d726b7da2ec0c.png)
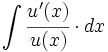
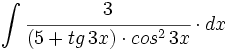
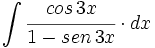
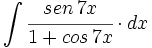
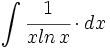
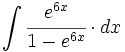
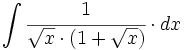
![\int \cfrac{1}{\sqrt[3]{x} \cdot (1+\sqrt[3]{x^2})} \cdot dx](/wikipedia/images/math/e/0/0/e00ec3c06bca7273fafc77dfb13d2365.png)