Plantilla:Videos ejemplos propiedades potencias racionales

De Wikipedia

(Diferencia entre revisiones)

Revisión de 19:13 12 ago 2017

Propiedades de las potencias de números racionales

Tutorial 1 (27'46") Sinopsis:

Tutorial muy completo que explica las propiedades básicas de las potencias con ejemplos resueltos sencillos y alguno más complejo.

Tutorial 2 (11'54") Sinopsis:

Potencias de exponente entero de números racionales.
Propiedades.
Ejemplos

Producto de potencias de la misma base (3'00") Sinopsis:

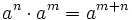$a^n \cdot a^m=a^{m+n}\;$ . Ejemplos.

Cociente de potencias de la misma base (2'32") Sinopsis:

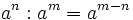$a^n : a^m=a^{m-n}\;$ . Ejemplos.

Potencia de exponente negativo (2'52") Sinopsis:

$a^{-n} = \cfrac{1}{a^n}\;$ . Ejemplos.

Potencia de exponente cero (1'35") Sinopsis:

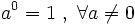$a^0 = 1 \ , \ \forall a \ne 0\;$ . Ejemplos.

Potencia de otra potencia (1'58") Sinopsis:

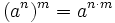$(a^n)^m = a^{n \cdot m}\;$ . Ejemplos.

Potencia de un producto (2'48") Sinopsis:

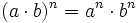$(a \cdot b)^n = a^n \cdot b^n\;$ . Ejemplos.

Potencia de un cociente (2'48") Sinopsis:

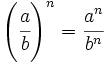$\left( \cfrac{a}{b} \right)^n = \cfrac{a^n}{b^n}\;$ . Ejemplos.

Ejemplos de potencias de fracciones (10'06") Sinopsis:

Tutorial que explica la potencia de exponente entero (positivo y negativo) con fracciones y operaciones combinadas con multiplicación, división y potencias, trabajando la simplificación previa.

Ejercicio 1 (2'09") Sinopsis:

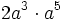
Simplifica: $2 a^3 \cdot a^5$

Ejercicio 2 (3'37") Sinopsis:

Simplifica: $\left( \cfrac{5 \cdot 4 \cdot 3}{6 \cdot 2} \right)^2$

Ejercicio 3 (3'42") Sinopsis: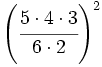

Simplifica: $\left[ \cfrac{(8^2 \cdot 8) \cdot (6^7 \cdot 6)^2}{(8^3)^3 \cdot (6^3)^0 \cdot 6^3} \right]^3$

Ejercicio 4 (3'40") Sinopsis:

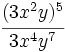
Simplifica: $\cfrac{(3x^2y)^5}{3x^4y^7}$

Ejercicio 5 (2'47") Sinopsis:

Simplifica: $\cfrac{2^{16}}{2^{13}}+\cfrac{5^3 \cdot 3^8}{5^2 \cdot 3^6}$

Ejercicio 6 (17'31") Sinopsis:

Simplifica y expresa la solución como una única potencia:

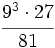
a) $\cfrac{9^3 \cdot 27}{81}$

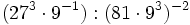
b) $(27^3 \cdot 9^{-1}) : (81 \cdot 9^3)^{-2}$

c) $\cfrac{16 \cdot 8^6 \cdot 2^{-3}}{(2^{-1})^{-3} \cdot 4}$

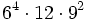
d) $6^4 \cdot 12 \cdot 9^2$

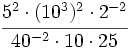
e) a) $\cfrac{5^2 \cdot (10^3)^2 \cdot 2^{-2}}{40^{-2} \cdot 10 \cdot 25}$

Ejercicio 7 (14'41") Sinopsis:

Simplifica y expresa la solución como una única potencia:

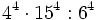
a) $4^4 \cdot 15^4 :6^4$

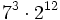
b) $7^3 \cdot 2^{12}$

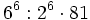
c) $6^6 : 2^6 \cdot 81$

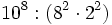
d) $10^8 : (8^2 \cdot 2^2)$

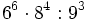
e) $6^6 \cdot 8^4 : 9^3$

f) No se pudo entender (función desconocida\cdfrac): \cdfrac{4^6 \cdot 9^3}{12^5}

Ejercicio 8 (11'39") Sinopsis:

Simplifica:

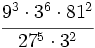
a) $\cfrac{9^3 \cdot 3^6 \cdot 81^2}{27^5 \cdot 3^2}$

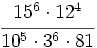
b) $\cfrac{15^6 \cdot 12^4}{10^5 \cdot 3^6 \cdot 81}$

Ejercicio 9 (9'43") Sinopsis:

Simplifica:

a) $\left[\left(\cfrac{3}{5} \right)^{-1} \cdot \left(\cfrac{9}{25} \right)^2 \right]^3$

b) $\left[\cfrac{16}{9} \cdot \left(\cfrac{56}{27} \right)^{-1} \right] \cdot \left(\cfrac{14}{9} \right)^3 \cdot \left(\cfrac{7}{12} \right)^{-2}$

Ejercicio 10 (4'05") Sinopsis:

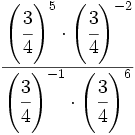
Simplifica $\cfrac{ \left(\cfrac{3}{4} \right)^5 \cdot \left(\cfrac{3}{4} \right)^{-2}}{ \left(\cfrac{3}{4} \right)^{-1} \cdot \left(\cfrac{3}{4} \right)^6}$

Ejercicio 11 (13'13") Sinopsis:

Simplifica:

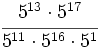
a) $\cfrac{5^{13} \cdot 5^{17}}{5^{11} \cdot 5^{16} \cdot 5^1}$

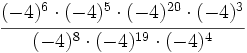
b) $\cfrac{(-4)^6 \cdot (-4)^5 \cdot (-4)^{20} \cdot (-4)^3}{(-4)^8 \cdot (-4)^{19} \cdot (-4)^4}$

c) $\left[ \cfrac{( 2^3 \cdot 2^6)^{-2} \cdot (3^4)^3 \cdot 3 }{( 2^6 \cdot 2^{10})^{-1}\cdot (3^6 \cdot 3^2 \cdot 3^5)}\right]^{10}$

Ejercicio 12 (2'08") Sinopsis:

Simplifica: $\left[ \left( \cfrac{1}{3} \right)^{10} : \left( \cfrac{1}{3} \right)^7 \right]^2$

Obtenido de "http://maralboran.org/wikipedia/index.php/Plantilla:Videos_ejemplos_propiedades_potencias_racionales"

 |sinopsis=Simplifica: {{Sube|porcentaje=20%|contenido=<math>\cfrac{2^{16}}{2^{13}}+\cfrac{5^3 \cdot 3^8}{5^2 \cdot 3^6}</math>}}
 |url1=https://www.youtube.com/watch?v=e9LKPUkjuaw&list=PLTDunuLIi6ogbD4_joialQfNyKekQcbnG&index=20
+}}
+{{Video_enlace_clasematicas
+|titulo1=Ejercicio 6
+|duracion=17'31"
+|sinopsis=Simplifica y expresa la solución como una única potencia:
+a) <math>\cfrac{9^3 \cdot 27}{81}</math>
+b) <math>(27^3 \cdot 9^{-1}) : (81 \cdot 9^3)^{-2} </math>
+c) <math>\cfrac{16 \cdot 8^6 \cdot 2^{-3}}{(2^{-1})^{-3} \cdot 4}</math>
+d) <math>6^4 \cdot 12 \cdot 9^2</math>
+e) a) <math>\cfrac{5^2 \cdot (10^3)^2 \cdot 2^{-2}}{40^{-2} \cdot 10 \cdot 25}</math>
+|url1=https://www.youtube.com/watch?v=Htf-4aBTvSQ&list=PLZNmE9BEzVIlrsOMVfmJS3vaKVqScVudR&index=2
+}}
+{{Video_enlace_clasematicas
+|titulo1=Ejercicio 7
+|duracion=14'41"
+|sinopsis=Simplifica y expresa la solución como una única potencia:
+a) <math>4^4 \cdot 15^4 :6^4</math>
+b) <math>7^3 \cdot 2^{12}</math>
+c) <math>6^6 : 2^6 \cdot 81</math>
+d) <math>10^8 : (8^2 \cdot 2^2)</math>
+e) <math>6^6 \cdot 8^4 : 9^3</math>
+f) <math>\cdfrac{4^6 \cdot 9^3}{12^5}</math>
+|url1=https://www.youtube.com/watch?v=If6mSe0qgkY&list=PLZNmE9BEzVIlrsOMVfmJS3vaKVqScVudR&index=3
 }}
 {{Video_enlace_abel
-|titulo1=Ejercicio 6
+|titulo1=Ejercicio 8
 |duracion=11'39"
 |sinopsis=Simplifica:
 }}
 {{Video_enlace_unicoos
-|titulo1=Ejercicio 7
+|titulo1=Ejercicio 9
 |duracion=9'43"
 |sinopsis=Simplifica:
 }}
 {{Video_enlace_julioprofe
-|titulo1=Ejercicio 8
+|titulo1=Ejercicio 10
 |duracion=4'05"
 |sinopsis=Simplifica <math>\cfrac{ \left(\cfrac{3}{4} \right)^5 \cdot \left(\cfrac{3}{4} \right)^{-2}}{ \left(\cfrac{3}{4} \right)^{-1} \cdot \left(\cfrac{3}{4} \right)^6}</math>
 }}
 {{Video_enlace_julioprofe
-|titulo1=Ejercicio 9
+|titulo1=Ejercicio 11
 |duracion=13'13"
 |sinopsis=Simplifica:
 }}
 {{Video_enlace_julioprofe
-|titulo1=Ejercicio 10
+|titulo1=Ejercicio 12
 |duracion=2'08"
 |sinopsis=Simplifica: <math>\left[ \left( \cfrac{1}{3} \right)^{10} : \left( \cfrac{1}{3} \right)^7 \right]^2 </math>
 |url1=https://www.youtube.com/watch?v=yw1lx9htI2I
 }}
-{{Video_enlace_clasematicas
-|titulo1=Ejercicio 11
-|duracion=17'31"
-|sinopsis=Simplifica y expresa la solución como una única potencia:
-a) <math>\cfrac{9^3 \cdot 27}{81}</math>
-b) <math>(27^3 \cdot 9^{-1}) : (81 \cdot 9^3)^{-2} </math>
-c) <math>\cfrac{16 \cdot 8^6 \cdot 2^{-3}}{(2^{-1})^{-3} \cdot 4}</math>
-d) <math>6^4 \cdot 12 \cdot 9^2</math>
-e) a) <math>\cfrac{5^2 \cdot (10^3)^2 \cdot 2^{-2}}{40^{-2} \cdot 10 \cdot 25}</math>
-|url1=https://www.youtube.com/watch?v=Htf-4aBTvSQ&list=PLZNmE9BEzVIlrsOMVfmJS3vaKVqScVudR&index=2
-}}
-{{Video_enlace_clasematicas
-|titulo1=Ejercicio 12
-|duracion=14'41"
-|sinopsis=Simplifica y expresa la solución como una única potencia:
-a) <math>4^4 \cdot 15^4 :6^4</math>
-b) <math>7^3 \cdot 2^{12}</math>
-c) <math>6^6 : 2^6 \cdot 81</math>
-d) <math>10^8 : (8^2 \cdot 2^2)</math>
-e) <math>6^6 \cdot 8^4 : 9^3</math>
-f) <math>\cdfrac{4^6 \cdot 9^3}{12^5}</math>
-|url1=https://www.youtube.com/watch?v=If6mSe0qgkY&list=PLZNmE9BEzVIlrsOMVfmJS3vaKVqScVudR&index=3
-}}
-{{Video_enlace_clasematicas
-|titulo1=Ejercicio 13
-|duracion=24'23"
-|sinopsis=Cuatro ejercicios de potencias en el que se utilizan también operaciones con fracciones,productos, divisiones y potencias.
-|url1=https://www.youtube.com/watch?v=wisBNVlGm_s&list=PLZNmE9BEzVIlrsOMVfmJS3vaKVqScVudR&index=4
-}}
 }}
 {{p}}

Plantilla:Videos ejemplos propiedades potencias racionales

De Wikipedia

Revisión de 19:13 12 ago 2017

Vistas

Herramientas personales

Menú

Buscar

Herramientas