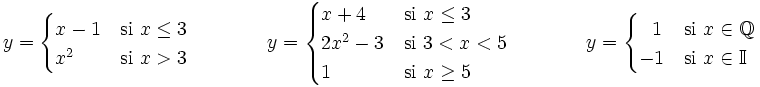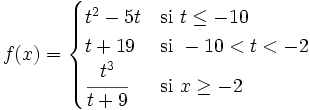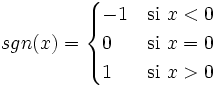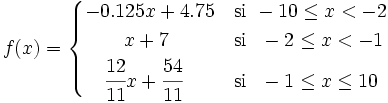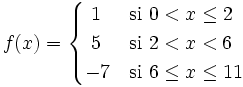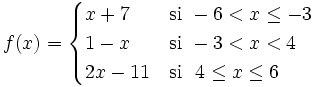Plantilla:Funciones definidas a trozos

De Wikipedia

(Diferencia entre revisiones)

 {{Caja_Amarilla|texto=
-Una '''función definida a trozos''' es aquella que utiliza varias expresiones para su definición, utilizando cada una de ellas en un determinado tramo del dominio de definición de la función principal.
+Una '''función definida a trozos''' es aquella que utiliza varias funciones para su definición, cada una de ellas definida en un determinado subconjunto del dominio de definición de la función principal.
 }}
 {{p}}
-{{Desplegable|titulo=Ejemplos:|contenido=Son funciones definidas a trozos:
+{{Ejemplo_simple|titulo=Ejemplos:|contenido=Son funciones definidas a trozos:
 :<math>y = \begin{cases} x-1 & \mbox{si }x \le 3 \\  x^2 & \mbox{si }x>3 \end{cases} \qquad \qquad y = \begin{cases} x+4 & \mbox{si }x \le 3 \\ 2x^2-3 & \mbox{si }3<x<5 \\ 1 & \mbox{si }x \ge 5 \end{cases} \qquad \qquad y = \begin{cases}  ~~1 & \mbox{si }x \in  \mathbb{Q} \\ -1 & \mbox{si }x\in  \mathbb{I} \end{cases}</math>
 }}
 {{p}}
-{{AI2|titulo=Actividad Interactiva: ''Funciones definidas a trozos''|cuerpo=
+{{Geogebra_enlace
-{{ai_cuerpo
+|descripcion=En esta escena podrás representar funciones definidas en hasta 4 trozos.
-|enunciado='''Actividad 1:''' Ejemplo de función definida a trozos.
+|enlace=[http://ggbm.at/JCV99Kf8 Graficador de funciones definidas a trozos]
-|actividad=
+}}
-En la siguiente escena puedes ver la representación de la función
+{{p}}
+{{Ejemplo|titulo=Ejemplos: ''Función definida a trozos''
+|enunciado=Representa la siguiente función:
-<center><math>y = \begin{cases} x+4 & \mbox{si }x \le 3 \\ 7-2(x-3) & \mbox{si }3<x<6 \\ 1 & \mbox{si }x \ge 6 \end{cases}</math></center>
+<center><math>y = \begin{cases} x^2 & \mbox{si }x \le 1 \\  2x+1 & \mbox{si }x>1 \end{cases}</math></center>
-Mueve el punto P para ver que expresión corresponde a cada tramo.
+|sol=
+Para su representación, tenemos que dibujar cada una de las funciones en sus respectivos tramos, prestando especial atención a los puntos de "empalme" (extremos de los intervalos de definición).
-<center><iframe>
+[[Imagen:f_a_trozos_1.jpg|300px|center]]
-url=http://maralboran.org/web_ma/descartes/Analisis/funcion_a_trozos/funcion_a_trozos2_1.html
-width=480
-height=390
-name=myframe
-</iframe></center>
-<center>[http://maralboran.org/web_ma/descartes/Analisis/funcion_a_trozos/funcion_a_trozos2_1.html '''Click''' aquí si no se ve bien la escena]</center>
-}}
-{{ai_cuerpo
-|enunciado='''Actividad 2:''' El entrenamiento y otros ejemplos de funciones a trozos.
-|actividad=
-<center><iframe>
-url=http://contenidos.santillanaenred.com/jukebox/servlet/GetPlayer?p3v=true&xref=200412010939_PRE_0_726754081&mode=1&rtc=1001&locale=es_ES&cache=false',600,400,'snrPop',0);
-width=100%
-height=600
-name=myframe
-</iframe></center>
-<center>[http://contenidos.santillanaenred.com/jukebox/servlet/GetPlayer?p3v=true&xref=200412010939_PRE_0_726754081&mode=1&rtc=1001&locale=es_ES&cache=false',600,400,'snrPop',0); '''Click''' aquí si no se ve bien la escena]</center>
-}}
-{{ai_cuerpo
-|enunciado='''Actividad 3:''' Une cada función con su gráfica.
-|actividad=
-<center><iframe>
-url=http://contenidos.santillanaenred.com/jukebox/servlet/GetPlayer?p3v=true&xref=200412010940_AC_0_751125357&mode=1&rtc=1001&locale=es_ES&cache=false',750,540,'snrPop',0);
-width=100%
-height=600
-name=myframe
-</iframe></center>
-<center>[http://contenidos.santillanaenred.com/jukebox/servlet/GetPlayer?p3v=true&xref=200412010940_AC_0_751125357&mode=1&rtc=1001&locale=es_ES&cache=false',750,540,'snrPop',0); '''Click''' aquí si no se ve bien la escena]</center>
-}}
 }}
 {{p}}
-{{Ejemplo|titulo=Ejemplo: ''Función definida a trozos''
+{{Ejemplo|titulo=Ejemplos: ''Función definida a trozos''
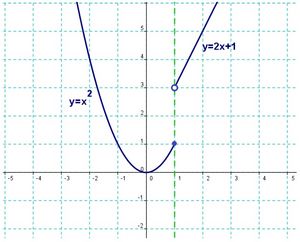
-|enunciado=Representa la siguiente función:
+|enunciado=Encuentra la expresión analítica de la función cuya gráfica es la siguiente:
+[[Imagen:funcion_trozos.png|250px|center]]
+|sol=
+Tendremos que hallar las ecuaciones de tres rectas. Para ello localizaremos dos puntos por los que pase cada recta y a partir de ellos obtendremos su pendiente y luego su ecuación por medio de la ecuación punto-pendiente.
+{{p}}
 <center><math>y = \begin{cases} x-3 & \mbox{si }x \le 0 \\ ~~2 & \mbox{si }0<x<3 \\ -x & \mbox{si }x \ge 3 \end{cases}</math></center>
-|sol=Para su representación, tenemos que dibujar cada una de las tres funciones en sus respectivos tramos, prestando especial atención a los puntos de "empalme" (extremos de los intervalos de definición).
-<center>[[Imagen:funcion_trozos.png|250px]]</center>
 }}
 {{p}}
-{{AI2|titulo=Actividad Interactiva: ''Funciones definidas a trozos''|cuerpo=
+{{Videotutoriales|titulo=Funciones definidas a trozos|enunciado=
-{{ai_cuerpo
+{{Video_enlace_clasematicas
-|enunciado='''Actividad 1:''' Funciones lineales definidas en dos trozos.
+|titulo1=Tutorial 1a
-|actividad=
+|duracion=21'12"
-En esta escena representaremos funciones a trozos del tipo:
+|url1=https://www.youtube.com/watch?v=jCDBKO8ghtg&list=PLZNmE9BEzVIkfJ32AmaQoob2npxScGpo3&index=5
+|sinopsis=Tutorial en el que se trabaja con las funciones definidas por partes en fórmulas, cálculo de imágenes y preimágenes de valores.
+}}
+{{Video_enlace_clasematicas
+|titulo1=Tutorial 1b
+|duracion=17'02"
+|url1=https://www.youtube.com/watch?v=KOXcDbcfj44&index=6&list=PLZNmE9BEzVIk3VQdqC9kJ8pcR0iJJMh87
+|sinopsis=Tutorial en el que se representa gráficamente funciones definidas por partes en fórmulas.
+}}
+----
+{{Video_enlace_khan
+|titulo1=Ejercicio 1
+|duracion=5'39"
+|sinopsis=Halla el valor de f(-10) en la siguiente función a trozos:
-<center><math>y = \begin{cases} ax+b & \mbox{si }x <k \\ cx+d & \mbox{si }x>k \end{cases}</math></center>
+:<math>f(x) = \begin{cases} t^2-5t & \mbox{si } t \le -10 \\  t+19 & \mbox{si }  -10<t<-2 \\  \cfrac{t^3}{t+9} & \mbox{si } x \ge -2 \end{cases}</math>
-Modifica con los controles los valores de a, b, c, d y k. Podrás obtener así distintas funciones a trozos.
+|url1=https://youtu.be/TSuuXnT7QMc
+}}
+{{Video_enlace_khan
+|titulo1=Ejercicio 2
+|duracion=7'32"
+|sinopsis=Obtén la expresión analítica de la función a trozos a partir de su gráfica dada en el video.
+|url1=https://youtu.be/6X_7SU2fy00
+}}
+{{Video_enlace_julioprofe
+|titulo1=Ejercicio 3
+|duracion=13'38"
+|sinopsis=Obtén la gráfica, el dominio y el rango de la siguiente función a trozos:
+:<math>f(x) = \begin{cases} x^2+2x & \mbox{si }x \le 1 \\  ~~x & \mbox{si } -1 < x \le 1 \\  -1 & \mbox{si } x>1 \end{cases}</math>
-<center><iframe>
+|url1=https://www.youtube.com/watch?v=AU1GVkYD78w
-url=http://maralboran.org/web_ma/descartes/Analisis/funcion_a_trozos/funcion_a_trozos3_1.html
-width=450
-height=380
-name=myframe
-</iframe></center>
-<center>[http://maralboran.org/web_ma/descartes/Analisis/funcion_a_trozos/funcion_a_trozos3_1.html '''Click''' aquí si no se ve bien la escena]</center>
 }}
-{{ai_cuerpo
+{{Video_enlace_unicoos
-|enunciado='''Actividad 2.'''  Representación gráfica de distintas funciones definidas a trozos (dos trozos).
+|titulo1=Ejercicio 4
-|actividad=
+|duracion=4'51"
-<center><iframe>
+|sinopsis=La '''función signo''' viene dada por la siguiente expresión:
-url=http://maralboran.org/web_ma/geogebra/figuras/ftrozos__1.html
-widfth=780
-height=460
-name=myframe
-</iframe></center>
-<center>[http://maralboran.org/web_ma/geogebra/figuras/f_trozos_1.html '''Click''' aquí si no se ve bien la escena]</center>
+:<math>sgn(x) = \begin{cases} -1 & \mbox{si } x < 0 \\  0 & \mbox{si }  x=0 \\  1 & \mbox{si } x>0 \end{cases}</math>
+Teniendo en cuenta esto representa: <math>y=sgn(4x-2)\;</math>.
+|url1=http://www.unicoos.com/video/matematicas/4-eso/funciones-elementales/funcion-signo/funcion-signo-01
 }}
+{{Video_enlace_khan
+|titulo1=Ejercicio 5
+|duracion=6'26"
+|sinopsis=Representa gráficamente la función a trozos:
+:<math>f(x) = \begin{cases} -0.125x+4.75 & \mbox{si } -10 \le x < -2 \\  \qquad x+7 & \mbox{si }  ~-2 \le x < -1 \\  \quad \cfrac{12}{11}x+\cfrac{54}{11} & \mbox{si } ~-1 \le x \le 10 \end{cases}</math>
+|url1=https://youtu.be/Gf1r578-E10
 }}
+{{Video_enlace_khan
+|titulo1=Ejercicio 6
+|duracion=3'22"
+|sinopsis=Halla el dominio y el rango de la siguiente función a trozos:
+:<math>f(x) = \begin{cases} ~1 & \mbox{si } 0 < x \le 2 \\  ~5 & \mbox{si }  2 < x < 6 \\  -7 & \mbox{si } 6 \le x \le 11 \end{cases}</math>
+|url1=https://youtu.be/mvsTzLxMGdc
+}}
+{{Video_enlace_khan
+|titulo1=Ejercicio 7
+|duracion=9'41"
+|sinopsis=Halla el dominio y el rango de la siguiente función a trozos:
+:<math>f(x) = \begin{cases} x+7 & \mbox{si } -6 < x \le -3 \\  1-x & \mbox{si }  -3 < x < 4 \\  2x-11 & \mbox{si } ~4 \le x \le 6 \end{cases}</math>
+|url1=https://youtu.be/3i7ZQ13ocI4
+}}
+}}
+{{Actividades|titulo=Funciones definidas a trozos|enunciado=
+{{AI_Khan
+|titulo1=Autoevaluación 1a
+|descripcion=Evaluar funciones definidas por partes
+|url1=http://es.khanacademy.org/math/algebra/absolute-value-equations-functions/piecewise-functions/e/evaluating-piecewise-functions
+}}
+{{AI_Khan
+|titulo1=Autoevaluación 1b
+|descripcion=Evaluar funciones definidas por partes
+|url1=http://es.khanacademy.org/math/algebra/absolute-value-equations-functions/piecewise-functions/e/evaluate-step-functions-from-their-graph
+}}
+{{AI_Khan
+|titulo1=Autoevaluación 2
+|descripcion=Representa gráficamente funciones definidas por partes
+|url1=http://es.khanacademy.org/math/algebra/absolute-value-equations-functions/piecewise-functions/e/piecewise-graphs-linear
+}}
+}}
 {{p}}

Revisión actual

Una función definida a trozos es aquella que utiliza varias funciones para su definición, cada una de ellas definida en un determinado subconjunto del dominio de definición de la función principal.

Ejemplos: [Mostrar]

Son funciones definidas a trozos:

$y = \begin{cases} x-1 & \mbox{si }x \le 3 \\ x^2 & \mbox{si }x>3 \end{cases} \qquad \qquad y = \begin{cases} x+4 & \mbox{si }x \le 3 \\ 2x^2-3 & \mbox{si }3<x<5 \\ 1 & \mbox{si }x \ge 5 \end{cases} \qquad \qquad y = \begin{cases} ~~1 & \mbox{si }x \in \mathbb{Q} \\ -1 & \mbox{si }x\in \mathbb{I} \end{cases}$

Graficador de funciones definidas a trozos Descripción: [Mostrar]

En esta escena podrás representar funciones definidas en hasta 4 trozos.

Ejemplos: Función definida a trozos

Representa la siguiente función:

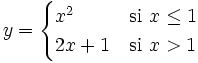
$y = \begin{cases} x^2 & \mbox{si }x \le 1 \\ 2x+1 & \mbox{si }x>1 \end{cases}$

Solución:[Mostrar]

Para su representación, tenemos que dibujar cada una de las funciones en sus respectivos tramos, prestando especial atención a los puntos de "empalme" (extremos de los intervalos de definición).

Ejemplos: Función definida a trozos

Encuentra la expresión analítica de la función cuya gráfica es la siguiente:

Solución:[Mostrar]

Tendremos que hallar las ecuaciones de tres rectas. Para ello localizaremos dos puntos por los que pase cada recta y a partir de ellos obtendremos su pendiente y luego su ecuación por medio de la ecuación punto-pendiente.

$y = \begin{cases} x-3 & \mbox{si }x \le 0 \\ ~~2 & \mbox{si }0<x<3 \\ -x & \mbox{si }x \ge 3 \end{cases}$

Funciones definidas a trozos [Mostrar]

Tutorial 1a (21'12") Sinopsis:[Mostrar]

Tutorial en el que se trabaja con las funciones definidas por partes en fórmulas, cálculo de imágenes y preimágenes de valores.

Tutorial 1b (17'02") Sinopsis:[Mostrar]

Tutorial en el que se representa gráficamente funciones definidas por partes en fórmulas.

Ejercicio 1 (5'39") Sinopsis: [Mostrar]

Halla el valor de f(-10) en la siguiente función a trozos:

$f(x) = \begin{cases} t^2-5t & \mbox{si } t \le -10 \\ t+19 & \mbox{si } -10<t<-2 \\ \cfrac{t^3}{t+9} & \mbox{si } x \ge -2 \end{cases}$

Ejercicio 2 (7'32") Sinopsis: [Mostrar]

Obtén la expresión analítica de la función a trozos a partir de su gráfica dada en el video.

Ejercicio 3 (13'38") Sinopsis: [Mostrar]

Obtén la gráfica, el dominio y el rango de la siguiente función a trozos:

$f(x) = \begin{cases} x^2+2x & \mbox{si }x \le 1 \\ ~~x & \mbox{si } -1 < x \le 1 \\ -1 & \mbox{si } x>1 \end{cases}$

Ejercicio 4 (4'51") Sinopsis: [Mostrar]

La función signo viene dada por la siguiente expresión:

$sgn(x) = \begin{cases} -1 & \mbox{si } x < 0 \\ 0 & \mbox{si } x=0 \\ 1 & \mbox{si } x>0 \end{cases}$

Teniendo en cuenta esto representa: 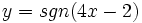$y=sgn(4x-2)\;$ .

Ejercicio 5 (6'26") Sinopsis: [Mostrar]

Representa gráficamente la función a trozos:

$f(x) = \begin{cases} -0.125x+4.75 & \mbox{si } -10 \le x < -2 \\ \qquad x+7 & \mbox{si } ~-2 \le x < -1 \\ \quad \cfrac{12}{11}x+\cfrac{54}{11} & \mbox{si } ~-1 \le x \le 10 \end{cases}$