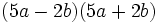Plantilla:Videos: identidades notables
De Wikipedia
(Diferencia entre revisiones)
| Revisión de 11:32 3 nov 2017 Coordinador (Discusión | contribuciones) ← Ir a diferencia anterior |
Revisión de 11:36 12 ene 2018 Coordinador (Discusión | contribuciones) Ir a siguiente diferencia → |
||
| Línea 36: | Línea 36: | ||
| ---- | ---- | ||
| {{Video_enlace_abel | {{Video_enlace_abel | ||
| - | |titulo1=Ejercicio 1 | + | |titulo1=Ejercicio 1a |
| |duracion=8'39" | |duracion=8'39" | ||
| |sinopsis=Desarrolla: | |sinopsis=Desarrolla: | ||
| Línea 47: | Línea 47: | ||
| }} | }} | ||
| {{Video_enlace_abel | {{Video_enlace_abel | ||
| - | |titulo1=Ejercicio 2 | + | |titulo1=Ejercicio 1b |
| |duracion=9'11" | |duracion=9'11" | ||
| |sinopsis=Desarrolla: | |sinopsis=Desarrolla: | ||
| Línea 57: | Línea 57: | ||
| }} | }} | ||
| {{Video_enlace_julioprofe | {{Video_enlace_julioprofe | ||
| - | |titulo1=Ejercicio 3 | + | |titulo1=Ejercicio 2a |
| |duracion=2'17" | |duracion=2'17" | ||
| |sinopsis=Desarrolla: <math>(3x+5y)^2\,</math> | |sinopsis=Desarrolla: <math>(3x+5y)^2\,</math> | ||
| Línea 63: | Línea 63: | ||
| }} | }} | ||
| {{Video_enlace_julioprofe | {{Video_enlace_julioprofe | ||
| - | |titulo1=Ejercicio 4 | + | |titulo1=Ejercicio 2b |
| |duracion=4'23" | |duracion=4'23" | ||
| |sinopsis=Desarrolla: <math>(2x^3+9y^4)^2\,</math> | |sinopsis=Desarrolla: <math>(2x^3+9y^4)^2\,</math> | ||
| Línea 69: | Línea 69: | ||
| }} | }} | ||
| {{Video_enlace_julioprofe | {{Video_enlace_julioprofe | ||
| - | |titulo1=Ejercicio 5 | + | |titulo1=Ejercicio 2c |
| |duracion=1'33" | |duracion=1'33" | ||
| |sinopsis=Desarrolla: <math>(7m-3c)^2\,</math> | |sinopsis=Desarrolla: <math>(7m-3c)^2\,</math> | ||
| Línea 75: | Línea 75: | ||
| }} | }} | ||
| {{Video_enlace_julioprofe | {{Video_enlace_julioprofe | ||
| - | |titulo1=Ejercicio 6 | + | |titulo1=Ejercicio 2d |
| |duracion=5'44" | |duracion=5'44" | ||
| |sinopsis=Desarrolla: <math>(4K^2L-5K^6L^3)^2\,</math> | |sinopsis=Desarrolla: <math>(4K^2L-5K^6L^3)^2\,</math> | ||
| Línea 81: | Línea 81: | ||
| }} | }} | ||
| {{Video_enlace_julioprofe | {{Video_enlace_julioprofe | ||
| - | |titulo1=Ejercicio 7 | + | |titulo1=Ejercicio 2e |
| |duracion=2'01" | |duracion=2'01" | ||
| |sinopsis=Desarrolla: <math>(5m^3+3n^4)\cdot(5m^3-3n^4)\,</math> | |sinopsis=Desarrolla: <math>(5m^3+3n^4)\cdot(5m^3-3n^4)\,</math> | ||
| |url1=https://www.youtube.com/watch?v=nFWyn0j8Flw&index=23&list=PL9B9AC3136D2D4C45}} | |url1=https://www.youtube.com/watch?v=nFWyn0j8Flw&index=23&list=PL9B9AC3136D2D4C45}} | ||
| {{Video_enlace_unicoos | {{Video_enlace_unicoos | ||
| - | |titulo1=Ejercicio 8 | + | |titulo1=Ejercicio 3 |
| |duracion=5'54" | |duracion=5'54" | ||
| |sinopsis=Desarrolla: | |sinopsis=Desarrolla: | ||
| Línea 103: | Línea 103: | ||
| }} | }} | ||
| {{Video_enlace_escuela | {{Video_enlace_escuela | ||
| - | |titulo1=Ejercicio 9 | + | |titulo1=Ejercicio 4a |
| |duracion=8'05" | |duracion=8'05" | ||
| |sinopsis=Desarrolla los siguientes productos notables: | |sinopsis=Desarrolla los siguientes productos notables: | ||
| Línea 116: | Línea 116: | ||
| }} | }} | ||
| {{Video_enlace_escuela | {{Video_enlace_escuela | ||
| - | |titulo1=Ejercicio 10 | + | |titulo1=Ejercicio 4b |
| |duracion=4'33" | |duracion=4'33" | ||
| |sinopsis=Desarrolla los siguientes productos notables: | |sinopsis=Desarrolla los siguientes productos notables: | ||
| Línea 128: | Línea 128: | ||
| }} | }} | ||
| {{Video_enlace_escuela | {{Video_enlace_escuela | ||
| - | |titulo1=Ejercicio 11 | + | |titulo1=Ejercicio 4c |
| |duracion=10'51" | |duracion=10'51" | ||
| |sinopsis=Desarrolla los siguientes productos notables: | |sinopsis=Desarrolla los siguientes productos notables: | ||
| Línea 146: | Línea 146: | ||
| |url1=https://www.youtube.com/watch?v=Cv3xDNwrh0I&list=PLw7Z_p6_h3oxSaTeel6R_pQaMSalig8UR&index=9 | |url1=https://www.youtube.com/watch?v=Cv3xDNwrh0I&list=PLw7Z_p6_h3oxSaTeel6R_pQaMSalig8UR&index=9 | ||
| + | }} | ||
| + | {{Video_enlace_khan | ||
| + | |titulo1=Ejercicio 5a | ||
| + | |duracion=5'56" | ||
| + | |sinopsis=Calcula: <math>(x+3)(x-3)\;</math> | ||
| + | |||
| + | |url1=https://youtu.be/Lb4v-gZ_KZ4 | ||
| + | }} | ||
| + | {{Video_enlace_khan | ||
| + | |titulo1=Ejercicio 5b | ||
| + | |duracion=7'19" | ||
| + | |sinopsis=Calcula: <math>(x+7)^2\;</math> | ||
| + | |||
| + | |url1=https://youtu.be/b0m1ZAuWX2M | ||
| + | }} | ||
| + | {{Video_enlace_khan | ||
| + | |titulo1=Ejercicio 5c | ||
| + | |duracion=2'30" | ||
| + | |sinopsis=Calcula: <math>(2x+8)(2x-8)\;</math> | ||
| + | |||
| + | |url1=https://youtu.be/sBhNnSoP10w | ||
| + | }} | ||
| + | {{Video_enlace_khan | ||
| + | |titulo1=Ejercicio 5d | ||
| + | |duracion=3'59" | ||
| + | |sinopsis=Calcula: <math>(7x+10)^2\;</math> | ||
| + | |||
| + | |url1=https://youtu.be/4y4EzvTgSiY | ||
| + | }} | ||
| + | {{Video_enlace_khan | ||
| + | |titulo1=Ejercicio 5e | ||
| + | |duracion=8'27" | ||
| + | |sinopsis=Halla el área de un cuadrado cuyo lado mide <math>(6x-5y)\;</math>. | ||
| + | |||
| + | |url1=https://youtu.be/mXFr19ToYkQ | ||
| + | }} | ||
| + | {{Video_enlace_khan | ||
| + | |titulo1=Ejercicio 5f | ||
| + | |duracion=10'35" | ||
| + | |sinopsis=Calcula: | ||
| + | |||
| + | :a) <math>(x+9)^2\;</math> | ||
| + | |||
| + | :b) <math>(x+b)^2\;</math> | ||
| + | |||
| + | :c) <math>(3x-7)^2\;</math> | ||
| + | |||
| + | :d) <math>(4x^2+y^2)^2\;</math> | ||
| + | |||
| + | :e) <math>(2x-1)(2x+1)\;</math> | ||
| + | |||
| + | :f) <math>(5a-2b)(5a+2b)\;</math> | ||
| + | |||
| + | |url1=https://youtu.be/D6wANZkEEOI | ||
| }} | }} | ||
| }} | }} | ||
Revisión de 11:36 12 ene 2018
Productos notables. Ejemplos.
- Fórmulas del cuadrado de una suma y de una diferencia.
- Ejemplos::
- a)

- b)

- Fórmula de la suma por diferencia.
- Ejemplos:
- a)
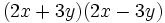
- b)
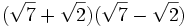
- c)
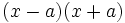
Como se desarrollan un binomio al cuadrado y una suma por diferencia.
Desarrolla:
- a)
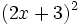
- b)
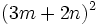
- c)
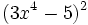
- d)
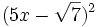
Desarrolla:
- a)
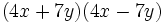
- b)
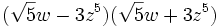
- c)

Desarrolla: 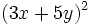
Desarrolla: 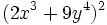
Desarrolla: 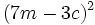
Desarrolla: 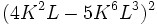
Desarrolla: 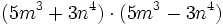
Desarrolla:
a) 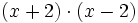
b) 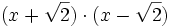
c) 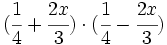
d) 
e) 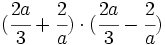
Desarrolla los siguientes productos notables:
- 2a)
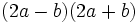
- 2b)
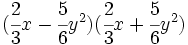
- 2c)
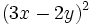
- 2d)
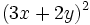
- 2e)

Desarrolla los siguientes productos notables:
- 4a)
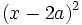
- 4b)
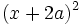
- 4c)

- 4d)
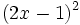
Desarrolla los siguientes productos notables:
- 5a)
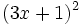
- 5b)

- 5c)
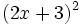
- 5d)
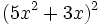
- 5e)
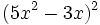
- 5f)
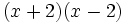
- 5g)
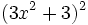
- 5h)

- 5i)
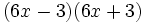
- 5j)

- 5k)
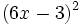
- 5l)

Calcula: 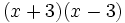
Calcula: 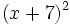
Calcula: 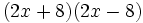
Calcula: 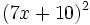
Halla el área de un cuadrado cuyo lado mide  .
.
Calcula:
- a)
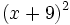
- b)
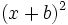
- c)
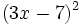
- d)
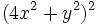
- e)

- f)