Plantilla:Divisibilidad de polinomios
De Wikipedia
| Revisión de 16:28 9 sep 2016 Coordinador (Discusión | contribuciones) (→Polinomios múltiplos y divisores) ← Ir a diferencia anterior |
Revisión actual Coordinador (Discusión | contribuciones) |
||
| Línea 1: | Línea 1: | ||
| ===Polinomios múltiplos y divisores=== | ===Polinomios múltiplos y divisores=== | ||
| - | {{Caja_Amarilla|texto=Un polinomio <math>Q(x)\,</math> es '''divisor''' de otro, <math>P(x)\,</math> y lo representaremos por <math>Q(x)|P(x)\;</math>, si la división <math>P(x):\,Q(x)\,</math> es exacta, es decir, cuando existe otro polinomio <math>C(x)\;</math> tal que: | + | {{Caja_Amarilla|texto=*Un polinomio <math>Q(x)\,</math> es '''divisor''' de otro, <math>P(x)\,</math> y lo representaremos por <math>Q(x)|P(x)\;</math>, si la división <math>P(x):\,Q(x)\,</math> es exacta, es decir, cuando existe otro polinomio <math>C(x)\;</math> tal que <math>P(x)=\,Q(x)\cdot C(x)\,</math>. |
| - | {{p}} | + | |
| - | {{Caja|contenido=<math>P(x)=\,Q(x)\cdot C(x)\,</math>}} | + | *En tal caso, diremos que <math>P(x)\,</math> es '''divisible''' por <math>Q(x)\,</math> y que <math>P(x)\,</math> es un '''múltiplo''' de <math>Q(x)\,</math>. |
| - | {{p}} | + | *También diremos que <math>Q(x)\,</math> y <math>C(x)\,</math> son factores del polnomio <math>P(x)\,</math>. |
| - | También diremos que <math>P(x)\,</math> es '''divisible''' por <math>Q(x)\,</math> o que <math>P(x)\,</math> es un '''múltiplo''' de <math>Q(x)\,</math>. | + | |
| }} | }} | ||
| {{p}} | {{p}} | ||
| - | {{Desplegable|titulo=Ejemplos:{{b}}|contenido= | + | {{Ejemplo_simple|titulo=Ejemplo|contenido= |
| - | + | Dados los polinomios: | |
| - | Sean <math> P(x)=(3x^3-14x^2+4x+3) \, , \quad Q(x)=(3x+1) \, , \quad C(x)=x^2-5x+3</math>: | + | |
| {{p}} | {{p}} | ||
| - | <math>Q(x)|P(x)\;</math>, porque <math>P(x)=\,Q(x)\cdot C(x)\,</math>: | + | <center><math> P(x)=(3x^3-14x^2+4x+3) \, , \quad Q(x)=(3x+1) \, , \quad C(x)=x^2-5x+3</math>:</center> |
| - | + | {{p}} | |
| - | <math> (3x^3-14x^2+4x+3):(3x+1)=x^2-5x+3\;</math>, porque <math>(3x+1) \cdot (x^2-5x+3) =3x^3-14x^2+4x+3</math> | + | Se cumple que |
| + | {{p}} | ||
| + | <center><math>Q(x)|P(x)\;</math>, porque <math>P(x)=\,Q(x)\cdot C(x)\,</math>.</center> | ||
| + | {{p}} | ||
| + | Es decir, la siguiente división es exacta: | ||
| + | {{p}} | ||
| + | <center><math> (3x^3-14x^2+4x+3):(3x+1)=x^2-5x+3\;</math></center> | ||
| + | {{p}} | ||
| + | porque: | ||
| + | {{p}} | ||
| + | <center><math>(3x+1) \cdot (x^2-5x+3) =3x^3-14x^2+4x+3</math></center> | ||
| }} | }} | ||
| {{p}} | {{p}} | ||
| Línea 22: | Línea 30: | ||
| ===Polinomios irreducibles=== | ===Polinomios irreducibles=== | ||
| - | {{Caja_Amarilla|texto=Un polinomio <math>P(x)\,</math> es '''irreducible''' cuando ningún polinomio de grado inferior es divisor suyo.}} | + | {{Caja_Amarilla|texto=Un polinomio <math>P(x)\,</math> es '''irreducible''' cuando ningún polinomio de grado inferior (distinto de grado cero) es divisor suyo.}} |
| {{p}} | {{p}} | ||
| - | {{Desplegable|titulo=Ejemplos:{{b}}|contenido= | + | {{Ejemplo_simple|titulo=Ejemplos|contenido= |
| Son polinomios irreducibles, entre otros: | Son polinomios irreducibles, entre otros: | ||
| + | |||
| * Los de primer grado: <math>3x,\ x-3,\ 5x-3\ \;</math> | * Los de primer grado: <math>3x,\ x-3,\ 5x-3\ \;</math> | ||
| * Los de segundo grado sin raíces: <math>x^2+1,\ 2x^2-3x+5 \;</math> | * Los de segundo grado sin raíces: <math>x^2+1,\ 2x^2-3x+5 \;</math> | ||
| + | }} | ||
| + | {{p}} | ||
| + | ===Máximo común divisor y mínimo común múltiplo=== | ||
| + | {{Caja_Amarilla|texto=Se dice que el polinomio <math>D(x)\;</math> es el '''máximo común divisor''' de los polinomios <math>P(x)\;</math> y <math>Q(x)\;</math>, y lo expresaremos: | ||
| + | |||
| + | <center><math>m.c.d \,[P(x), Q(x)]=D(x)\;</math></center> | ||
| + | |||
| + | si <math>D(x)\;</math> es divisor de ambos y no existe otro polinomio que divida a ambos que tenga mayor grado que él. | ||
| + | }} | ||
| + | {{p}} | ||
| + | {{Ejemplo_simple|titulo=Ejemplos|contenido= | ||
| + | Dados los polinomios: | ||
| + | |||
| + | :<math>P(x)=(x-1)^2 (x-3)\;</math> | ||
| + | :<math>Q(x)=(x-1)^3 (x-3)^2 (x+1)\;</math>: | ||
| + | |||
| + | Su máximo común divisor es: | ||
| + | |||
| + | :<math>m.c.d \,[P(x), Q(x)]=(x-1)^2(x-3)\;</math> | ||
| + | }} | ||
| + | {{Caja_Amarilla|texto=Se dice que el polinomio <math>M(x)\;</math> es el '''mínimo común múltiplo''' de los polinomios <math>P(x)\;</math> y <math>Q(x)\;</math>, y lo expresaremos: | ||
| + | |||
| + | <center><math>m.c.m \,[P(x), Q(x)]=M(x)\;</math></center> | ||
| + | |||
| + | si <math>D(x)\;</math> es múltiplo de ambos y no existe otro polinomio que sea múltiplo de ambos que tenga menor grado que él. | ||
| + | }} | ||
| + | {{p}} | ||
| + | {{Ejemplo_simple|titulo=Ejemplos|contenido= | ||
| + | Dados los polinomios: | ||
| + | |||
| + | :<math>P(x)=(x-1)^2 (x-3)\;</math> | ||
| + | :<math>Q(x)=(x-1)^3 (x-3)^2 (x+1)\;</math>: | ||
| + | |||
| + | |||
| + | Su mínimo común múltiplo es: | ||
| + | |||
| + | :<math>m.c.m \,[P(x), Q(x)]=(x-1)^3 (x-3)^2 (x+1)\;</math> | ||
| }} | }} | ||
Revisión actual
Polinomios múltiplos y divisores
- Un polinomio
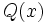 es divisor de otro,
es divisor de otro,  y lo representaremos por
y lo representaremos por 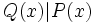 , si la división
, si la división 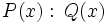 es exacta, es decir, cuando existe otro polinomio
es exacta, es decir, cuando existe otro polinomio 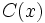 tal que
tal que 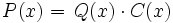 .
.
- En tal caso, diremos que
 es divisible por
es divisible por 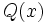 y que
y que  es un múltiplo de
es un múltiplo de 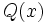 .
.
- También diremos que
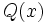 y
y 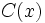 son factores del polnomio
son factores del polnomio  .
.
Dados los polinomios:
 :
:Se cumple que
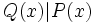 , porque
, porque 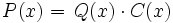 .
.Es decir, la siguiente división es exacta:
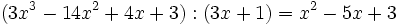
porque:
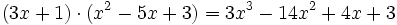
La divisibilidad de polinomios es semejante a la divisibilidad con números enteros. Asimismo, la factorización de polinomios equivale a la descomposición de un número en factores primos, y los conceptos de máximo común divisor, mínimo común múltiplo e irreducibilidad son similares a los correspondientes conceptos numéricos.
Polinomios irreducibles
Un polinomio  es irreducible cuando ningún polinomio de grado inferior (distinto de grado cero) es divisor suyo.
es irreducible cuando ningún polinomio de grado inferior (distinto de grado cero) es divisor suyo.
Son polinomios irreducibles, entre otros:
- Los de primer grado:

- Los de segundo grado sin raíces:
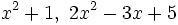
Máximo común divisor y mínimo común múltiplo
Se dice que el polinomio 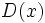 es el máximo común divisor de los polinomios
es el máximo común divisor de los polinomios 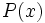 y
y 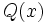 , y lo expresaremos:
, y lo expresaremos:
![m.c.d \,[P(x), Q(x)]=D(x)\;](/wikipedia/images/math/c/6/6/c66f3168e53561a04ed46aade0f24ca0.png)
si 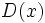 es divisor de ambos y no existe otro polinomio que divida a ambos que tenga mayor grado que él.
es divisor de ambos y no existe otro polinomio que divida a ambos que tenga mayor grado que él.
Se dice que el polinomio 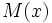 es el mínimo común múltiplo de los polinomios
es el mínimo común múltiplo de los polinomios 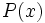 y
y 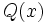 , y lo expresaremos:
, y lo expresaremos:
![m.c.m \,[P(x), Q(x)]=M(x)\;](/wikipedia/images/math/2/2/1/221e514439439421dc1201091dd735b3.png)
si 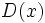 es múltiplo de ambos y no existe otro polinomio que sea múltiplo de ambos que tenga menor grado que él.
es múltiplo de ambos y no existe otro polinomio que sea múltiplo de ambos que tenga menor grado que él.

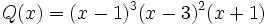 :
:
![m.c.d \,[P(x), Q(x)]=(x-1)^2(x-3)\;](/wikipedia/images/math/9/6/c/96c02ce39375f7c1585c25c27c0c0fcb.png)
![m.c.m \,[P(x), Q(x)]=(x-1)^3 (x-3)^2 (x+1)\;](/wikipedia/images/math/8/d/5/8d598eb8a1613c40ffc2eebba001fa1f.png)

