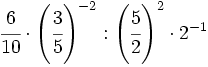Plantilla:Videos ejemplos propiedades potencias racionales
De Wikipedia
| Revisión de 09:24 11 nov 2017 Coordinador (Discusión | contribuciones) ← Ir a diferencia anterior |
Revisión actual Coordinador (Discusión | contribuciones) |
||
| Línea 69: | Línea 69: | ||
| |sinopsis=Potencia de un cociente: <math>\left( \cfrac{a}{b} \right)^n = \cfrac{a^n}{b^n}\;</math>. Ejemplos. | |sinopsis=Potencia de un cociente: <math>\left( \cfrac{a}{b} \right)^n = \cfrac{a^n}{b^n}\;</math>. Ejemplos. | ||
| |url1=https://www.youtube.com/watch?v=oAcdsF4fO94&list=PLo7_lpX1yruM4Eki_2jP4vJRepj5j2QS3&index=7 | |url1=https://www.youtube.com/watch?v=oAcdsF4fO94&list=PLo7_lpX1yruM4Eki_2jP4vJRepj5j2QS3&index=7 | ||
| + | }} | ||
| + | {{Video_enlace_pildoras | ||
| + | |titulo1=Tutorial 5a | ||
| + | |duracion=8'12" | ||
| + | |sinopsis=*Potencias de exponente 1 y 0. | ||
| + | *Producto y cociente de potencias de la misma base. | ||
| + | *Potencia de un producto y de un cociente. | ||
| + | *Potencia de otra potencia. | ||
| + | *Ejemplos. | ||
| + | |url1=http://youtu.be/yc4-A5edFK0?list=PLwCiNw1sXMSCPdAlIxXUq7vq8c4wzj8To | ||
| + | }} | ||
| + | {{Video_enlace_pildoras | ||
| + | |titulo1=Tutorial 5b | ||
| + | |duracion=6'26" | ||
| + | |sinopsis=*Potencias de base negativa. | ||
| + | *Potencias de exponente negativo. | ||
| + | *Ejemplos. | ||
| + | |url1=http://youtu.be/NFPibwNulnI?list=PLwCiNw1sXMSCPdAlIxXUq7vq8c4wzj8To | ||
| }} | }} | ||
| ---- | ---- | ||
| - | {{Video_enlace_tuprofesorvirtual | + | {{Video_enlace_pildoras |
| + | |titulo1=Ejemplos 1 | ||
| + | |duracion=5'40" | ||
| + | |sinopsis=Cálculos con potencias de exponente positivo. | ||
| + | |url1=https://youtu.be/Ex1geRx9_Eg?list=PLwCiNw1sXMSCPdAlIxXUq7vq8c4wzj8To | ||
| + | }} | ||
| + | {{Video_enlace_pildoras | ||
| + | |titulo1=Ejemplos 2 | ||
| + | |duracion=3'35" | ||
| + | |sinopsis=Cálculos con potencias de exponente negativo. | ||
| + | |url1=https://youtu.be/CaOr3UK_xtg?list=PLwCiNw1sXMSCPdAlIxXUq7vq8c4wzj8To | ||
| + | }} | ||
| + | {{Video_enlace_pildoras | ||
| + | |titulo1=Ejemplos 3 | ||
| + | |duracion=6'55" | ||
| + | |sinopsis=Simplificaciones de operaciones con potencias. | ||
| + | |url1=https://youtu.be/kQFv3cEKtM8?list=PLwCiNw1sXMSCPdAlIxXUq7vq8c4wzj8To | ||
| + | }} | ||
| + | ---- | ||
| + | '''Cálculos con potencias de fracciones:''' | ||
| + | |||
| + | {{Video_enlace_escuela | ||
| |titulo1=Ejercicio 1 | |titulo1=Ejercicio 1 | ||
| + | |duracion=10'49" | ||
| + | |sinopsis=Calcula: | ||
| + | |||
| + | 20) <math>\left( \cfrac{-2~}{3} \right)^4 \cdot \left( \cfrac{-2~}{3} \right)^9</math> ; {{b4}}{{b4}} 21) <math>\left( \cfrac{-3~}{2} \right)^4 \cdot \left( \cfrac{-3~}{2} \right)^3</math> ; {{b4}}{{b4}} 22) <math>\left( \cfrac{3}{5} \right)^6 \cdot \left( \cfrac{3}{5} \right)^{-2}</math> | ||
| + | |||
| + | 23) <math>\left( \cfrac{1}{4} \right)^{-2} \cdot \left( \cfrac{1}{4} \right)^{-3}</math> ; {{b4}}{{b4}} 24) <math>\left( \cfrac{1}{4} \right)^5 \cdot \left( \cfrac{1}{4} \right)^{-3}</math> ; {{b4}}{{b4}} 25) <math>\left( \cfrac{2}{3} \right)^7 \cdot \left( \cfrac{2}{3} \right)^{-3}</math> | ||
| + | |||
| + | 26) <math>\left( \cfrac{-2~}{3} \right)^{-4} \cdot \left( \cfrac{-2~}{3} \right)^8</math> ; {{b4}}{{b4}} 27) <math>\left( \cfrac{2}{13} \right)^{-7} \cdot \left( \cfrac{2}{13} \right)^9</math> ; {{b4}}{{b4}} 28) <math>\left( \cfrac{1}{9} \right)^7 \cdot \left( \cfrac{1}{9} \right)^{-6}</math> | ||
| + | |||
| + | |url1=https://www.youtube.com/watch?v=6aC_gfAZE58&index=11&list=PLw7Z_p6_h3oxskBUdR5nmGa6YMySFNSY- | ||
| + | }} | ||
| + | {{Video_enlace_escuela | ||
| + | |titulo1=Ejercicio 2 | ||
| + | |duracion=8'36" | ||
| + | |sinopsis=Calcula: | ||
| + | |||
| + | 29) <math>\left( \cfrac{3}{4} \right)^3 : \left( \cfrac{3}{4} \right)^2</math> ; {{b4}}{{b4}} 30) <math>\left( \cfrac{4}{3} \right)^{-2} : \left( \cfrac{4}{3} \right)^4</math> ; {{b4}}{{b4}} 31) <math>\left( \cfrac{3}{7} \right)^6 \cdot \left( \cfrac{3}{7} \right)^4</math> | ||
| + | |||
| + | 32) <math>\left( \cfrac{2}{5} \right)^{-2} : \left( \cfrac{2}{5} \right)^6</math> ; {{b4}}{{b4}} 33) <math>\left( \cfrac{-2~}{3} \right)^{-5} : \left( \cfrac{-2~}{3} \right)^{-3}</math> ; {{b4}}{{b4}} 34) <math>\left( \cfrac{-1~}{7} \right)^4 \cdot \left( \cfrac{-1~}{7} \right)^{-3}</math> | ||
| + | |||
| + | |url1=https://www.youtube.com/watch?v=PQWjTEzvjG4&index=12&list=PLw7Z_p6_h3oxskBUdR5nmGa6YMySFNSY- | ||
| + | }} | ||
| + | {{Video_enlace_escuela | ||
| + | |titulo1=Ejercicio 3 | ||
| + | |duracion=10'26" | ||
| + | |sinopsis=Calcula: | ||
| + | |||
| + | :35) <math>\left[ \left( \cfrac{-3~}{7} \right)^2 \right]^3</math> ; {{b4}}{{b4}} 36) <math>\left[ \left( \cfrac{2}{3} \right)^3 \right]^5</math> ; {{b4}}{{b4}} 37) <math>\left[ \left( \cfrac{3}{4} \right)^2 \right]^4</math> | ||
| + | |||
| + | :38) <math>\left[ \left( \cfrac{2}{5} \right)^3 \right]^2</math> ; {{b4}}{{b4}} 39) <math>\left[ \left( \cfrac{2}{3} \right)^{-2} \right]^{-3}</math>; {{b4}}{{b4}} 40) <math>\left[ \left( \cfrac{3}{5} \right)^{-2} \right]^{-7}</math> | ||
| + | |||
| + | :41) <math>\left[ \left( \cfrac{-3~}{4} \right)^{-2} \right]^{-5}</math> ; {{b4}}{{b4}} 42) <math>\left[ \left( \cfrac{-2~}{7} \right)^3 \right]^{-2}</math> ; {{b4}}{{b4}} 43) <math>\left[ \left( \cfrac{-2~}{7} \right)^{-1} \right]^3</math> | ||
| + | |||
| + | :44) <math>\left[ \left( \cfrac{2}{5} \right)^{-3} \right]^{-2}</math> ; {{b4}}{{b4}} 45) <math>\left[ \left( \cfrac{-2~}{5} \right)^{-3} \right]^{-2}</math> ; {{b4}}{{b4}} 46) <math>\left[ \left( \cfrac{-1~}{8} \right)^{-2} \right]^{-3}</math> | ||
| + | |||
| + | |url1=https://www.youtube.com/watch?v=49qBtEWXgYU&list=PLw7Z_p6_h3oxskBUdR5nmGa6YMySFNSY-&index=13 | ||
| + | }} | ||
| + | {{Video_enlace_escuela | ||
| + | |titulo1=Ejercicio 4 | ||
| + | |duracion=5'28" | ||
| + | |sinopsis=Escribe como varias potencias: | ||
| + | |||
| + | :47) <math>\left( \cfrac{2}{3} \cdot \cfrac{1}{4} \cdot \cfrac{6}{5}\right)^2</math> | ||
| + | |||
| + | :48) <math>\left( \cfrac{1}{4} \cdot \cfrac{6}{25} \cdot \cfrac{5}{9}\right)^3</math> | ||
| + | |||
| + | :49) <math>\left( \cfrac{4}{5} \cdot \cfrac{5}{7} \cdot \cfrac{8}{6}\right)^3</math> | ||
| + | |||
| + | :50) <math>\left[ \cfrac{1}{3} \cdot \left( \cfrac{-2~}{5} \right) \cdot \left( \cfrac{-2~}{2} \right) \right]^2</math> | ||
| + | |||
| + | :51) <math>\left[ \cfrac{2}{3} \cdot \left( \cfrac{-5~}{4} \right) \cdot \cfrac{8}{5} \cdot \left( \cfrac{-5~}{2} \right) \right]^{-3}</math> | ||
| + | |url1=https://www.youtube.com/watch?v=Be6PSqNjvds&list=PLw7Z_p6_h3oxskBUdR5nmGa6YMySFNSY-&index=14 | ||
| + | }} | ||
| + | {{Video_enlace_escuela | ||
| + | |titulo1=Ejercicio 5 | ||
| + | |duracion=13'54" | ||
| + | |sinopsis=Escribe como una sola potencia: | ||
| + | |||
| + | :52) <math>\left( \cfrac{2}{5} \right)^2 \cdot \left( \cfrac{3}{7} \right)^2 \cdot \left( \cfrac{9}{11} \right)^2</math> | ||
| + | |||
| + | :53) <math>\left( \cfrac{1}{3} \right)^3 \cdot \left( \cfrac{1}{4} \right)^3 \cdot \left( \cfrac{5}{6} \right)^3</math> | ||
| + | |||
| + | :55) <math>\left( \cfrac{-2~}{3} \right)^2 \cdot \left( \cfrac{5}{4} \right)^2 \cdot \left( \cfrac{-3~}{5} \right)^2</math> | ||
| + | |||
| + | :56) <math>\left( \cfrac{-2~}{5} \right)^{-3} \cdot \left( \cfrac{1}{3} \right)^{-3} \cdot \left( \cfrac{5}{4} \right)^{-3}</math> | ||
| + | |||
| + | :57) <math>\left( \cfrac{-2~}{3} \right)^{-2} \cdot \left( \cfrac{1}{5} \right)^{-2} \cdot \left( \cfrac{3}{4} \right)^{-2}</math> | ||
| + | |||
| + | :58) <math>\left( \cfrac{2}{4} \right)^{-1} \cdot \left( \cfrac{-3~}{5} \right)^{-1} \cdot \left( \cfrac{5}{7} \right)^{-1}</math> | ||
| + | |||
| + | |||
| + | |||
| + | |url1=https://www.youtube.com/watch?v=06M-dlh6tSw&list=PLw7Z_p6_h3oxskBUdR5nmGa6YMySFNSY-&index=15 | ||
| + | }} | ||
| + | |||
| + | {{Video_enlace_unicoos | ||
| + | |titulo1=Ejercicio 6 | ||
| + | |duracion=9'43" | ||
| + | |sinopsis=Simplifica: | ||
| + | :a) <math>\left[\left(\cfrac{3}{5} \right)^{-1} \cdot \left(\cfrac{9}{25} \right)^2 \right]^3</math> | ||
| + | |||
| + | :b) <math>\left[\cfrac{16}{9} \cdot \left(\cfrac{56}{27} \right)^{-1} \right] \cdot \left(\cfrac{14}{9} \right)^3 \cdot \left(\cfrac{7}{12} \right)^{-2} </math> | ||
| + | |url1=https://www.youtube.com/watch?v=KdkMb7wSV4U | ||
| + | }} | ||
| + | {{Video_enlace_julioprofe | ||
| + | |titulo1=Ejercicio 7 | ||
| + | |duracion=4'05" | ||
| + | |sinopsis=Simplifica <math>\cfrac{ \left(\cfrac{3}{4} \right)^5 \cdot \left(\cfrac{3}{4} \right)^{-2}}{ \left(\cfrac{3}{4} \right)^{-1} \cdot \left(\cfrac{3}{4} \right)^6}</math> | ||
| + | |url1=https://www.youtube.com/watch?v=mQiYuVeXZxM | ||
| + | }} | ||
| + | {{Video_enlace_julioprofe | ||
| + | |titulo1=Ejercicio 8 | ||
| + | |duracion=2'08" | ||
| + | |sinopsis=Simplifica: <math>\left[ \left( \cfrac{1}{3} \right)^{10} : \left( \cfrac{1}{3} \right)^7 \right]^2 </math> | ||
| + | |url1=https://www.youtube.com/watch?v=yw1lx9htI2I | ||
| + | }} | ||
| + | ---- | ||
| + | '''Cálculos con potencias dentro de fracciones:''' | ||
| + | |||
| + | {{Video_enlace_escuela | ||
| + | |titulo1=Ejercicio 1 | ||
| + | |duracion=10'23" | ||
| + | |sinopsis=Calcula: | ||
| + | |||
| + | :59) <math>\cfrac{3^2 \cdot 3^3 \cdot 3}{3^4}\;</math> | ||
| + | |||
| + | :60) <math>\cfrac{4^{-1} \cdot 4^{-3}}{4^{-2} \cdot 4^{-5}}\;</math> | ||
| + | |||
| + | :61) <math>\cfrac{2 \cdot 2^{-3}}{2^{-2}}\;</math> | ||
| + | |||
| + | :62) <math>\cfrac{(-2)^{-3} \cdot (-2)^5 \cdot (-2)}{(-2)^{-1} \cdot (-2)^3}\;</math> | ||
| + | |||
| + | |url1=https://www.youtube.com/watch?v=dhd1kiF-oPg&list=PLw7Z_p6_h3oxskBUdR5nmGa6YMySFNSY-&index=16 | ||
| + | }} | ||
| + | {{Video_enlace_escuela | ||
| + | |titulo1=Ejercicio 2 | ||
| + | |duracion=7'23" | ||
| + | |sinopsis=Calcula: | ||
| + | |||
| + | :63) <math>\cfrac{2^2 \cdot 2^{-3} \cdot 2^5}{2^4 \cdot 2^{-1}}\;</math> | ||
| + | |||
| + | :64) <math>\cfrac{6^{-1} \cdot (6^5)^2}{6^2 \cdot 6^3}\;</math> | ||
| + | |||
| + | :65) <math>\cfrac{4^{-1} \cdot 4^{-3}}{4^{-2} \cdot 4^{-4}}\;</math> | ||
| + | |||
| + | :66) <math>\cfrac{(-3)^{-2} \cdot (-3)^{-3}}{\left[ (-3)^2 \right]^{-2}}\;</math> | ||
| + | |||
| + | |url1=https://www.youtube.com/watch?v=Tojm9kQ1FjU&list=PLw7Z_p6_h3oxskBUdR5nmGa6YMySFNSY-&index=17 | ||
| + | }} | ||
| + | {{Video_enlace_tuprofesorvirtual | ||
| + | |titulo1=Ejercicio 3 | ||
| |duracion=3'37" | |duracion=3'37" | ||
| |sinopsis=Simplifica: {{Sube|porcentaje=20%|contenido=<math>\left( \cfrac{5 \cdot 4 \cdot 3}{6 \cdot 2} \right)^2</math>}} | |sinopsis=Simplifica: {{Sube|porcentaje=20%|contenido=<math>\left( \cfrac{5 \cdot 4 \cdot 3}{6 \cdot 2} \right)^2</math>}} | ||
| Línea 78: | Línea 248: | ||
| }} | }} | ||
| {{Video_enlace_tuprofesorvirtual | {{Video_enlace_tuprofesorvirtual | ||
| - | |titulo1=Ejercicio 2 | + | |titulo1=Ejercicio 4 |
| |duracion=3'42" | |duracion=3'42" | ||
| |sinopsis=Simplifica: {{Sube|porcentaje=20%|contenido=<math>\left[ \cfrac{(8^2 \cdot 8) \cdot (6^7 \cdot 6)^2}{(8^3)^3 \cdot (6^3)^0 \cdot 6^3} \right]^3</math>}} | |sinopsis=Simplifica: {{Sube|porcentaje=20%|contenido=<math>\left[ \cfrac{(8^2 \cdot 8) \cdot (6^7 \cdot 6)^2}{(8^3)^3 \cdot (6^3)^0 \cdot 6^3} \right]^3</math>}} | ||
| Línea 84: | Línea 254: | ||
| }} | }} | ||
| {{Video_enlace_tuprofesorvirtual | {{Video_enlace_tuprofesorvirtual | ||
| - | |titulo1=Ejercicio 3 | + | |titulo1=Ejercicio 5 |
| |duracion=3'40" | |duracion=3'40" | ||
| |sinopsis=Simplifica: {{Sube|porcentaje=20%|contenido=<math>\cfrac{(3x^2y)^5}{3x^4y^7}</math>}} | |sinopsis=Simplifica: {{Sube|porcentaje=20%|contenido=<math>\cfrac{(3x^2y)^5}{3x^4y^7}</math>}} | ||
| Línea 90: | Línea 260: | ||
| }} | }} | ||
| {{Video_enlace_tuprofesorvirtual | {{Video_enlace_tuprofesorvirtual | ||
| - | |titulo1=Ejercicio 4 | + | |titulo1=Ejercicio 6 |
| |duracion=2'47" | |duracion=2'47" | ||
| |sinopsis=Simplifica: {{Sube|porcentaje=20%|contenido=<math>\cfrac{2^{16}}{2^{13}}+\cfrac{5^3 \cdot 3^8}{5^2 \cdot 3^6}</math>}} | |sinopsis=Simplifica: {{Sube|porcentaje=20%|contenido=<math>\cfrac{2^{16}}{2^{13}}+\cfrac{5^3 \cdot 3^8}{5^2 \cdot 3^6}</math>}} | ||
| Línea 96: | Línea 266: | ||
| }} | }} | ||
| {{Video_enlace_clasematicas | {{Video_enlace_clasematicas | ||
| - | |titulo1=Ejercicio 5 | + | |titulo1=Ejercicio 7 |
| |duracion=17'31" | |duracion=17'31" | ||
| |sinopsis=Simplifica y expresa la solución como una única potencia: | |sinopsis=Simplifica y expresa la solución como una única potencia: | ||
| Línea 112: | Línea 282: | ||
| }} | }} | ||
| {{Video_enlace_clasematicas | {{Video_enlace_clasematicas | ||
| - | |titulo1=Ejercicio 6 | + | |titulo1=Ejercicio 8 |
| |duracion=14'41" | |duracion=14'41" | ||
| |sinopsis=Simplifica y expresa la solución como una única potencia: | |sinopsis=Simplifica y expresa la solución como una única potencia: | ||
| Línea 130: | Línea 300: | ||
| }} | }} | ||
| {{Video_enlace_abel | {{Video_enlace_abel | ||
| - | |titulo1=Ejercicio 7 | + | |titulo1=Ejercicio 9 |
| |duracion=11'39" | |duracion=11'39" | ||
| |sinopsis=Simplifica: | |sinopsis=Simplifica: | ||
| Línea 138: | Línea 308: | ||
| }} | }} | ||
| {{Video_enlace_julioprofe | {{Video_enlace_julioprofe | ||
| - | |titulo1=Ejercicio 8 | + | |titulo1=Ejercicio 10 |
| |duracion=13'13" | |duracion=13'13" | ||
| |sinopsis=Simplifica: | |sinopsis=Simplifica: | ||
| Línea 149: | Línea 319: | ||
| }} | }} | ||
| ---- | ---- | ||
| - | {{Video_enlace_unicoos | + | '''Cálculos de diversos tipos:''' |
| - | |titulo1=Ejercicio 1 | + | |
| - | |duracion=9'43" | + | |
| - | |sinopsis=Simplifica: | + | |
| - | :a) <math>\left[\left(\cfrac{3}{5} \right)^{-1} \cdot \left(\cfrac{9}{25} \right)^2 \right]^3</math> | + | |
| - | :b) <math>\left[\cfrac{16}{9} \cdot \left(\cfrac{56}{27} \right)^{-1} \right] \cdot \left(\cfrac{14}{9} \right)^3 \cdot \left(\cfrac{7}{12} \right)^{-2} </math> | ||
| - | |url1=https://www.youtube.com/watch?v=KdkMb7wSV4U | ||
| - | }} | ||
| - | {{Video_enlace_julioprofe | ||
| - | |titulo1=Ejercicio 2 | ||
| - | |duracion=4'05" | ||
| - | |sinopsis=Simplifica <math>\cfrac{ \left(\cfrac{3}{4} \right)^5 \cdot \left(\cfrac{3}{4} \right)^{-2}}{ \left(\cfrac{3}{4} \right)^{-1} \cdot \left(\cfrac{3}{4} \right)^6}</math> | ||
| - | |url1=https://www.youtube.com/watch?v=mQiYuVeXZxM | ||
| - | }} | ||
| - | {{Video_enlace_julioprofe | ||
| - | |titulo1=Ejercicio 3 | ||
| - | |duracion=2'08" | ||
| - | |sinopsis=Simplifica: <math>\left[ \left( \cfrac{1}{3} \right)^{10} : \left( \cfrac{1}{3} \right)^7 \right]^2 </math> | ||
| - | |url1=https://www.youtube.com/watch?v=yw1lx9htI2I | ||
| - | }} | ||
| {{Video_enlace_clasematicas | {{Video_enlace_clasematicas | ||
| - | |titulo1=Ejercicios 4 | + | |titulo1=Ejercicio 1 |
| |duracion=10'06" | |duracion=10'06" | ||
| |sinopsis=Tutorial que explica la potencia de exponente entero (positivo y negativo) con fracciones y operaciones combinadas con multiplicación, división y potencias, trabajando la simplificación previa. | |sinopsis=Tutorial que explica la potencia de exponente entero (positivo y negativo) con fracciones y operaciones combinadas con multiplicación, división y potencias, trabajando la simplificación previa. | ||
Revisión actual
Tutorial muy completo que explica las propiedades básicas de las potencias con ejemplos resueltos sencillos y alguno más complejo.
- Potencias de exponente entero de números racionales.
- Propiedades.
- Ejemplos
Producto de potencias de fracciones con la misma base. Ejemplos.
Cociente de potencias de fracciones con la misma base. Ejemplos.
Potencia de otra potencia de una fracción. Ejemplos.
Potencia de un producto de fracciones. Ejemplos.
Producto de potencias de la misma base: 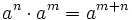 . Ejemplos.
. Ejemplos.
Cociente de potencias de la misma base: 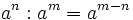 . Ejemplos.
. Ejemplos.
Potencia de otra potencia: 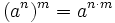 . Ejemplos.
. Ejemplos.
Potencia de un producto: 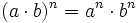 . Ejemplos.
. Ejemplos.
Potencia de un cociente: 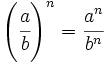 . Ejemplos.
. Ejemplos.
- Potencias de exponente 1 y 0.
- Producto y cociente de potencias de la misma base.
- Potencia de un producto y de un cociente.
- Potencia de otra potencia.
- Ejemplos.
- Potencias de base negativa.
- Potencias de exponente negativo.
- Ejemplos.
Cálculos con potencias de exponente positivo.
Cálculos con potencias de exponente negativo.
Simplificaciones de operaciones con potencias.
Cálculos con potencias de fracciones:
Calcula:
20) 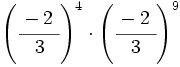 ; 21)
; 21) 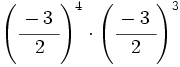 ; 22)
; 22) 
23) 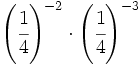 ; 24)
; 24) 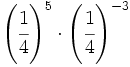 ; 25)
; 25) 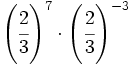
26) 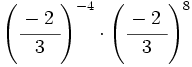 ; 27)
; 27) 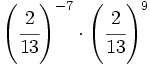 ; 28)
; 28) 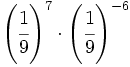
Calcula:
29) 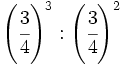 ; 30)
; 30)  ; 31)
; 31) 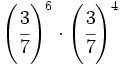
32)  ; 33)
; 33) 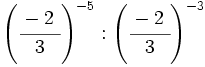 ; 34)
; 34) 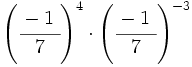
Calcula:
- 35)
![\left[ \left( \cfrac{-3~}{7} \right)^2 \right]^3](/wikipedia/images/math/0/d/5/0d5ecdfc87205ac8f368d6a70a5e1225.png) ; 36)
; 36) ![\left[ \left( \cfrac{2}{3} \right)^3 \right]^5](/wikipedia/images/math/2/9/c/29cf02b990eee91e9f5267cd7ae27ab8.png) ; 37)
; 37) ![\left[ \left( \cfrac{3}{4} \right)^2 \right]^4](/wikipedia/images/math/8/5/9/85905a10045ecdadf0d87cb496b46fd4.png)
- 38)
![\left[ \left( \cfrac{2}{5} \right)^3 \right]^2](/wikipedia/images/math/7/7/c/77ccd9469ab61459b42b5c42c31b6a03.png) ; 39)
; 39) ![\left[ \left( \cfrac{2}{3} \right)^{-2} \right]^{-3}](/wikipedia/images/math/7/1/7/717d66168670db7c17604eed1ecc5697.png) ; 40)
; 40) ![\left[ \left( \cfrac{3}{5} \right)^{-2} \right]^{-7}](/wikipedia/images/math/7/d/5/7d5523f771792c7a7444cc796d7a29e6.png)
- 41)
![\left[ \left( \cfrac{-3~}{4} \right)^{-2} \right]^{-5}](/wikipedia/images/math/d/e/2/de20c03afc7ad82625777cdcf282c409.png) ; 42)
; 42) ![\left[ \left( \cfrac{-2~}{7} \right)^3 \right]^{-2}](/wikipedia/images/math/d/8/f/d8f17a4b8bd520d1104f16e3bbe69742.png) ; 43)
; 43) ![\left[ \left( \cfrac{-2~}{7} \right)^{-1} \right]^3](/wikipedia/images/math/d/9/9/d996341203e6a65e266433c712564961.png)
- 44)
![\left[ \left( \cfrac{2}{5} \right)^{-3} \right]^{-2}](/wikipedia/images/math/e/2/b/e2b4f1793a567fb108f0a0c11c815bcb.png) ; 45)
; 45) ![\left[ \left( \cfrac{-2~}{5} \right)^{-3} \right]^{-2}](/wikipedia/images/math/1/5/4/154452bc7b4e3830e7474ee197167695.png) ; 46)
; 46) ![\left[ \left( \cfrac{-1~}{8} \right)^{-2} \right]^{-3}](/wikipedia/images/math/d/f/7/df755b98eccd3553b490053829676d47.png)
Escribe como varias potencias:
- 47)
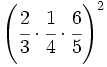
- 48)

- 49)

- 50)
![\left[ \cfrac{1}{3} \cdot \left( \cfrac{-2~}{5} \right) \cdot \left( \cfrac{-2~}{2} \right) \right]^2](/wikipedia/images/math/5/b/1/5b1a30cdfd3990559ce215699a00c2e3.png)
- 51)
![\left[ \cfrac{2}{3} \cdot \left( \cfrac{-5~}{4} \right) \cdot \cfrac{8}{5} \cdot \left( \cfrac{-5~}{2} \right) \right]^{-3}](/wikipedia/images/math/4/0/6/4066e6d3414c3e8d17a4b0e6837cad6a.png)
Escribe como una sola potencia:
- 52)
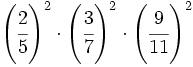
- 53)
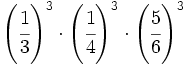
- 55)
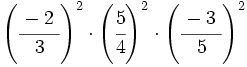
- 56)
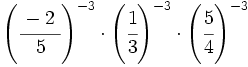
- 57)

- 58)
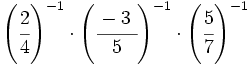
Simplifica:
- a)
![\left[\left(\cfrac{3}{5} \right)^{-1} \cdot \left(\cfrac{9}{25} \right)^2 \right]^3](/wikipedia/images/math/f/e/c/fec21991837955f9b9d945d4600cba18.png)
- b)
![\left[\cfrac{16}{9} \cdot \left(\cfrac{56}{27} \right)^{-1} \right] \cdot \left(\cfrac{14}{9} \right)^3 \cdot \left(\cfrac{7}{12} \right)^{-2}](/wikipedia/images/math/e/5/1/e5178ed20376c4ef7f07ca88d5d43975.png)
Simplifica 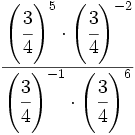
Simplifica: ![\left[ \left( \cfrac{1}{3} \right)^{10} : \left( \cfrac{1}{3} \right)^7 \right]^2](/wikipedia/images/math/6/9/1/6911b07dbbc54e802f87f7498ab77b09.png)
Cálculos con potencias dentro de fracciones:
Calcula:
- 59)

- 60)
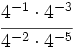
- 61)
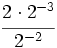
- 62)
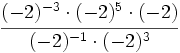
Calcula:
- 63)
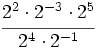
- 64)
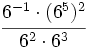
- 65)
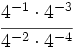
- 66)
![\cfrac{(-3)^{-2} \cdot (-3)^{-3}}{\left[ (-3)^2 \right]^{-2}}\;](/wikipedia/images/math/5/e/b/5eb0dbb75ab3ac5de8238f112e4d893f.png)
Simplifica: 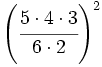
Simplifica: ![\left[ \cfrac{(8^2 \cdot 8) \cdot (6^7 \cdot 6)^2}{(8^3)^3 \cdot (6^3)^0 \cdot 6^3} \right]^3](/wikipedia/images/math/7/1/b/71b5b62cb54ed835ba335fdbca4de909.png)
Simplifica: 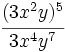
Simplifica: 
Simplifica y expresa la solución como una única potencia:
a) 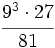
b) 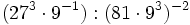
c) 
d) 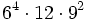
e) a) 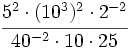
Simplifica y expresa la solución como una única potencia:
a) 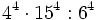
b) 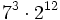
c) 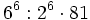
d) 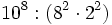
e) 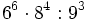
f) 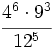
Simplifica:
- a)
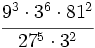
- b)
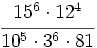
Simplifica:
- a)
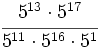
- b)
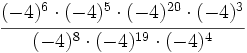
- c)
![\left[ \cfrac{( 2^3 \cdot 2^6)^{-2} \cdot (3^4)^3 \cdot 3 }{( 2^6 \cdot 2^{10})^{-1}\cdot (3^6 \cdot 3^2 \cdot 3^5)}\right]^{10}](/wikipedia/images/math/c/8/5/c85c926802bdbb7a51ef34eb65c6dda7.png)
Cálculos de diversos tipos:
Tutorial que explica la potencia de exponente entero (positivo y negativo) con fracciones y operaciones combinadas con multiplicación, división y potencias, trabajando la simplificación previa.
Exponente positivo:
- a)
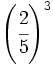 ; b)
; b)  ; c)
; c)  ; d)
; d) 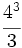 ; e)
; e) 
Exponente negativo:
- f)
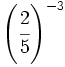 ; g)
; g) 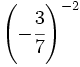 ; h)
; h)  ; i)
; i) 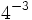 ; j)
; j) 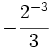
Operaciones combinadas:
- k)
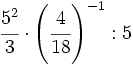 ; l)
; l)