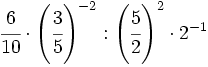Plantilla:Videos ejemplos propiedades potencias racionales
De Wikipedia
| Revisión de 12:23 11 nov 2017 Coordinador (Discusión | contribuciones) ← Ir a diferencia anterior |
Revisión actual Coordinador (Discusión | contribuciones) |
||
| Línea 69: | Línea 69: | ||
| |sinopsis=Potencia de un cociente: <math>\left( \cfrac{a}{b} \right)^n = \cfrac{a^n}{b^n}\;</math>. Ejemplos. | |sinopsis=Potencia de un cociente: <math>\left( \cfrac{a}{b} \right)^n = \cfrac{a^n}{b^n}\;</math>. Ejemplos. | ||
| |url1=https://www.youtube.com/watch?v=oAcdsF4fO94&list=PLo7_lpX1yruM4Eki_2jP4vJRepj5j2QS3&index=7 | |url1=https://www.youtube.com/watch?v=oAcdsF4fO94&list=PLo7_lpX1yruM4Eki_2jP4vJRepj5j2QS3&index=7 | ||
| + | }} | ||
| + | {{Video_enlace_pildoras | ||
| + | |titulo1=Tutorial 5a | ||
| + | |duracion=8'12" | ||
| + | |sinopsis=*Potencias de exponente 1 y 0. | ||
| + | *Producto y cociente de potencias de la misma base. | ||
| + | *Potencia de un producto y de un cociente. | ||
| + | *Potencia de otra potencia. | ||
| + | *Ejemplos. | ||
| + | |url1=http://youtu.be/yc4-A5edFK0?list=PLwCiNw1sXMSCPdAlIxXUq7vq8c4wzj8To | ||
| + | }} | ||
| + | {{Video_enlace_pildoras | ||
| + | |titulo1=Tutorial 5b | ||
| + | |duracion=6'26" | ||
| + | |sinopsis=*Potencias de base negativa. | ||
| + | *Potencias de exponente negativo. | ||
| + | *Ejemplos. | ||
| + | |url1=http://youtu.be/NFPibwNulnI?list=PLwCiNw1sXMSCPdAlIxXUq7vq8c4wzj8To | ||
| + | }} | ||
| + | ---- | ||
| + | {{Video_enlace_pildoras | ||
| + | |titulo1=Ejemplos 1 | ||
| + | |duracion=5'40" | ||
| + | |sinopsis=Cálculos con potencias de exponente positivo. | ||
| + | |url1=https://youtu.be/Ex1geRx9_Eg?list=PLwCiNw1sXMSCPdAlIxXUq7vq8c4wzj8To | ||
| + | }} | ||
| + | {{Video_enlace_pildoras | ||
| + | |titulo1=Ejemplos 2 | ||
| + | |duracion=3'35" | ||
| + | |sinopsis=Cálculos con potencias de exponente negativo. | ||
| + | |url1=https://youtu.be/CaOr3UK_xtg?list=PLwCiNw1sXMSCPdAlIxXUq7vq8c4wzj8To | ||
| + | }} | ||
| + | {{Video_enlace_pildoras | ||
| + | |titulo1=Ejemplos 3 | ||
| + | |duracion=6'55" | ||
| + | |sinopsis=Simplificaciones de operaciones con potencias. | ||
| + | |url1=https://youtu.be/kQFv3cEKtM8?list=PLwCiNw1sXMSCPdAlIxXUq7vq8c4wzj8To | ||
| }} | }} | ||
| ---- | ---- | ||
| Línea 89: | Línea 126: | ||
| |titulo1=Ejercicio 2 | |titulo1=Ejercicio 2 | ||
| |duracion=8'36" | |duracion=8'36" | ||
| - | |sinopsis=Ejercicios 29 al 34: Cociente de potencias de fracciones. | + | |sinopsis=Calcula: |
| + | |||
| + | 29) <math>\left( \cfrac{3}{4} \right)^3 : \left( \cfrac{3}{4} \right)^2</math> ; {{b4}}{{b4}} 30) <math>\left( \cfrac{4}{3} \right)^{-2} : \left( \cfrac{4}{3} \right)^4</math> ; {{b4}}{{b4}} 31) <math>\left( \cfrac{3}{7} \right)^6 \cdot \left( \cfrac{3}{7} \right)^4</math> | ||
| + | |||
| + | 32) <math>\left( \cfrac{2}{5} \right)^{-2} : \left( \cfrac{2}{5} \right)^6</math> ; {{b4}}{{b4}} 33) <math>\left( \cfrac{-2~}{3} \right)^{-5} : \left( \cfrac{-2~}{3} \right)^{-3}</math> ; {{b4}}{{b4}} 34) <math>\left( \cfrac{-1~}{7} \right)^4 \cdot \left( \cfrac{-1~}{7} \right)^{-3}</math> | ||
| + | |||
| |url1=https://www.youtube.com/watch?v=PQWjTEzvjG4&index=12&list=PLw7Z_p6_h3oxskBUdR5nmGa6YMySFNSY- | |url1=https://www.youtube.com/watch?v=PQWjTEzvjG4&index=12&list=PLw7Z_p6_h3oxskBUdR5nmGa6YMySFNSY- | ||
| }} | }} | ||
| Línea 95: | Línea 137: | ||
| |titulo1=Ejercicio 3 | |titulo1=Ejercicio 3 | ||
| |duracion=10'26" | |duracion=10'26" | ||
| - | |sinopsis=Ejercicios 35 al 46: Calcular la potencia de una potencia de una fracción. | + | |sinopsis=Calcula: |
| + | |||
| + | :35) <math>\left[ \left( \cfrac{-3~}{7} \right)^2 \right]^3</math> ; {{b4}}{{b4}} 36) <math>\left[ \left( \cfrac{2}{3} \right)^3 \right]^5</math> ; {{b4}}{{b4}} 37) <math>\left[ \left( \cfrac{3}{4} \right)^2 \right]^4</math> | ||
| + | |||
| + | :38) <math>\left[ \left( \cfrac{2}{5} \right)^3 \right]^2</math> ; {{b4}}{{b4}} 39) <math>\left[ \left( \cfrac{2}{3} \right)^{-2} \right]^{-3}</math>; {{b4}}{{b4}} 40) <math>\left[ \left( \cfrac{3}{5} \right)^{-2} \right]^{-7}</math> | ||
| + | |||
| + | :41) <math>\left[ \left( \cfrac{-3~}{4} \right)^{-2} \right]^{-5}</math> ; {{b4}}{{b4}} 42) <math>\left[ \left( \cfrac{-2~}{7} \right)^3 \right]^{-2}</math> ; {{b4}}{{b4}} 43) <math>\left[ \left( \cfrac{-2~}{7} \right)^{-1} \right]^3</math> | ||
| + | |||
| + | :44) <math>\left[ \left( \cfrac{2}{5} \right)^{-3} \right]^{-2}</math> ; {{b4}}{{b4}} 45) <math>\left[ \left( \cfrac{-2~}{5} \right)^{-3} \right]^{-2}</math> ; {{b4}}{{b4}} 46) <math>\left[ \left( \cfrac{-1~}{8} \right)^{-2} \right]^{-3}</math> | ||
| + | |||
| |url1=https://www.youtube.com/watch?v=49qBtEWXgYU&list=PLw7Z_p6_h3oxskBUdR5nmGa6YMySFNSY-&index=13 | |url1=https://www.youtube.com/watch?v=49qBtEWXgYU&list=PLw7Z_p6_h3oxskBUdR5nmGa6YMySFNSY-&index=13 | ||
| }} | }} | ||
| Línea 101: | Línea 152: | ||
| |titulo1=Ejercicio 4 | |titulo1=Ejercicio 4 | ||
| |duracion=5'28" | |duracion=5'28" | ||
| - | |sinopsis=Ejercicios 47 al 51: Calcular potencias de productos de fracciones. | + | |sinopsis=Escribe como varias potencias: |
| + | |||
| + | :47) <math>\left( \cfrac{2}{3} \cdot \cfrac{1}{4} \cdot \cfrac{6}{5}\right)^2</math> | ||
| + | |||
| + | :48) <math>\left( \cfrac{1}{4} \cdot \cfrac{6}{25} \cdot \cfrac{5}{9}\right)^3</math> | ||
| + | |||
| + | :49) <math>\left( \cfrac{4}{5} \cdot \cfrac{5}{7} \cdot \cfrac{8}{6}\right)^3</math> | ||
| + | |||
| + | :50) <math>\left[ \cfrac{1}{3} \cdot \left( \cfrac{-2~}{5} \right) \cdot \left( \cfrac{-2~}{2} \right) \right]^2</math> | ||
| + | |||
| + | :51) <math>\left[ \cfrac{2}{3} \cdot \left( \cfrac{-5~}{4} \right) \cdot \cfrac{8}{5} \cdot \left( \cfrac{-5~}{2} \right) \right]^{-3}</math> | ||
| |url1=https://www.youtube.com/watch?v=Be6PSqNjvds&list=PLw7Z_p6_h3oxskBUdR5nmGa6YMySFNSY-&index=14 | |url1=https://www.youtube.com/watch?v=Be6PSqNjvds&list=PLw7Z_p6_h3oxskBUdR5nmGa6YMySFNSY-&index=14 | ||
| }} | }} | ||
| Línea 107: | Línea 168: | ||
| |titulo1=Ejercicio 5 | |titulo1=Ejercicio 5 | ||
| |duracion=13'54" | |duracion=13'54" | ||
| - | |sinopsis=Ejercicios 52 al 58: Calcular productos de potencias de fracciones. | + | |sinopsis=Escribe como una sola potencia: |
| + | |||
| + | :52) <math>\left( \cfrac{2}{5} \right)^2 \cdot \left( \cfrac{3}{7} \right)^2 \cdot \left( \cfrac{9}{11} \right)^2</math> | ||
| + | |||
| + | :53) <math>\left( \cfrac{1}{3} \right)^3 \cdot \left( \cfrac{1}{4} \right)^3 \cdot \left( \cfrac{5}{6} \right)^3</math> | ||
| + | |||
| + | :55) <math>\left( \cfrac{-2~}{3} \right)^2 \cdot \left( \cfrac{5}{4} \right)^2 \cdot \left( \cfrac{-3~}{5} \right)^2</math> | ||
| + | |||
| + | :56) <math>\left( \cfrac{-2~}{5} \right)^{-3} \cdot \left( \cfrac{1}{3} \right)^{-3} \cdot \left( \cfrac{5}{4} \right)^{-3}</math> | ||
| + | |||
| + | :57) <math>\left( \cfrac{-2~}{3} \right)^{-2} \cdot \left( \cfrac{1}{5} \right)^{-2} \cdot \left( \cfrac{3}{4} \right)^{-2}</math> | ||
| + | |||
| + | :58) <math>\left( \cfrac{2}{4} \right)^{-1} \cdot \left( \cfrac{-3~}{5} \right)^{-1} \cdot \left( \cfrac{5}{7} \right)^{-1}</math> | ||
| + | |||
| + | |||
| + | |||
| |url1=https://www.youtube.com/watch?v=06M-dlh6tSw&list=PLw7Z_p6_h3oxskBUdR5nmGa6YMySFNSY-&index=15 | |url1=https://www.youtube.com/watch?v=06M-dlh6tSw&list=PLw7Z_p6_h3oxskBUdR5nmGa6YMySFNSY-&index=15 | ||
| }} | }} | ||
| Línea 138: | Línea 214: | ||
| |titulo1=Ejercicio 1 | |titulo1=Ejercicio 1 | ||
| |duracion=10'23" | |duracion=10'23" | ||
| - | |sinopsis=Ejercicios 59 al 62: Cálculos con potencias dentro de fracciones. | + | |sinopsis=Calcula: |
| + | |||
| + | :59) <math>\cfrac{3^2 \cdot 3^3 \cdot 3}{3^4}\;</math> | ||
| + | |||
| + | :60) <math>\cfrac{4^{-1} \cdot 4^{-3}}{4^{-2} \cdot 4^{-5}}\;</math> | ||
| + | |||
| + | :61) <math>\cfrac{2 \cdot 2^{-3}}{2^{-2}}\;</math> | ||
| + | |||
| + | :62) <math>\cfrac{(-2)^{-3} \cdot (-2)^5 \cdot (-2)}{(-2)^{-1} \cdot (-2)^3}\;</math> | ||
| + | |||
| |url1=https://www.youtube.com/watch?v=dhd1kiF-oPg&list=PLw7Z_p6_h3oxskBUdR5nmGa6YMySFNSY-&index=16 | |url1=https://www.youtube.com/watch?v=dhd1kiF-oPg&list=PLw7Z_p6_h3oxskBUdR5nmGa6YMySFNSY-&index=16 | ||
| }} | }} | ||
| Línea 144: | Línea 229: | ||
| |titulo1=Ejercicio 2 | |titulo1=Ejercicio 2 | ||
| |duracion=7'23" | |duracion=7'23" | ||
| - | |sinopsis=Ejercicios 63 al 66: Cálculos de potencias dentro de fracciones. | + | |sinopsis=Calcula: |
| + | |||
| + | :63) <math>\cfrac{2^2 \cdot 2^{-3} \cdot 2^5}{2^4 \cdot 2^{-1}}\;</math> | ||
| + | |||
| + | :64) <math>\cfrac{6^{-1} \cdot (6^5)^2}{6^2 \cdot 6^3}\;</math> | ||
| + | |||
| + | :65) <math>\cfrac{4^{-1} \cdot 4^{-3}}{4^{-2} \cdot 4^{-4}}\;</math> | ||
| + | |||
| + | :66) <math>\cfrac{(-3)^{-2} \cdot (-3)^{-3}}{\left[ (-3)^2 \right]^{-2}}\;</math> | ||
| + | |||
| |url1=https://www.youtube.com/watch?v=Tojm9kQ1FjU&list=PLw7Z_p6_h3oxskBUdR5nmGa6YMySFNSY-&index=17 | |url1=https://www.youtube.com/watch?v=Tojm9kQ1FjU&list=PLw7Z_p6_h3oxskBUdR5nmGa6YMySFNSY-&index=17 | ||
| }} | }} | ||
Revisión actual
Tutorial muy completo que explica las propiedades básicas de las potencias con ejemplos resueltos sencillos y alguno más complejo.
- Potencias de exponente entero de números racionales.
- Propiedades.
- Ejemplos
Producto de potencias de fracciones con la misma base. Ejemplos.
Cociente de potencias de fracciones con la misma base. Ejemplos.
Potencia de otra potencia de una fracción. Ejemplos.
Potencia de un producto de fracciones. Ejemplos.
Producto de potencias de la misma base: 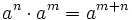 . Ejemplos.
. Ejemplos.
Cociente de potencias de la misma base: 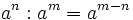 . Ejemplos.
. Ejemplos.
Potencia de otra potencia: 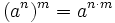 . Ejemplos.
. Ejemplos.
Potencia de un producto: 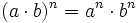 . Ejemplos.
. Ejemplos.
Potencia de un cociente: 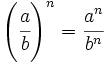 . Ejemplos.
. Ejemplos.
- Potencias de exponente 1 y 0.
- Producto y cociente de potencias de la misma base.
- Potencia de un producto y de un cociente.
- Potencia de otra potencia.
- Ejemplos.
- Potencias de base negativa.
- Potencias de exponente negativo.
- Ejemplos.
Cálculos con potencias de exponente positivo.
Cálculos con potencias de exponente negativo.
Simplificaciones de operaciones con potencias.
Cálculos con potencias de fracciones:
Calcula:
20) 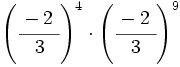 ; 21)
; 21) 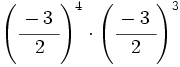 ; 22)
; 22) 
23) 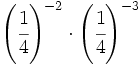 ; 24)
; 24) 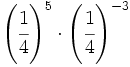 ; 25)
; 25) 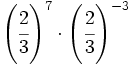
26) 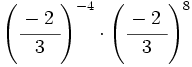 ; 27)
; 27) 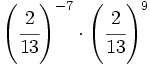 ; 28)
; 28) 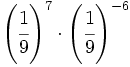
Calcula:
29) 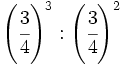 ; 30)
; 30)  ; 31)
; 31) 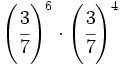
32)  ; 33)
; 33) 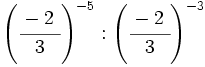 ; 34)
; 34) 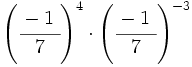
Calcula:
- 35)
![\left[ \left( \cfrac{-3~}{7} \right)^2 \right]^3](/wikipedia/images/math/0/d/5/0d5ecdfc87205ac8f368d6a70a5e1225.png) ; 36)
; 36) ![\left[ \left( \cfrac{2}{3} \right)^3 \right]^5](/wikipedia/images/math/2/9/c/29cf02b990eee91e9f5267cd7ae27ab8.png) ; 37)
; 37) ![\left[ \left( \cfrac{3}{4} \right)^2 \right]^4](/wikipedia/images/math/8/5/9/85905a10045ecdadf0d87cb496b46fd4.png)
- 38)
![\left[ \left( \cfrac{2}{5} \right)^3 \right]^2](/wikipedia/images/math/7/7/c/77ccd9469ab61459b42b5c42c31b6a03.png) ; 39)
; 39) ![\left[ \left( \cfrac{2}{3} \right)^{-2} \right]^{-3}](/wikipedia/images/math/7/1/7/717d66168670db7c17604eed1ecc5697.png) ; 40)
; 40) ![\left[ \left( \cfrac{3}{5} \right)^{-2} \right]^{-7}](/wikipedia/images/math/7/d/5/7d5523f771792c7a7444cc796d7a29e6.png)
- 41)
![\left[ \left( \cfrac{-3~}{4} \right)^{-2} \right]^{-5}](/wikipedia/images/math/d/e/2/de20c03afc7ad82625777cdcf282c409.png) ; 42)
; 42) ![\left[ \left( \cfrac{-2~}{7} \right)^3 \right]^{-2}](/wikipedia/images/math/d/8/f/d8f17a4b8bd520d1104f16e3bbe69742.png) ; 43)
; 43) ![\left[ \left( \cfrac{-2~}{7} \right)^{-1} \right]^3](/wikipedia/images/math/d/9/9/d996341203e6a65e266433c712564961.png)
- 44)
![\left[ \left( \cfrac{2}{5} \right)^{-3} \right]^{-2}](/wikipedia/images/math/e/2/b/e2b4f1793a567fb108f0a0c11c815bcb.png) ; 45)
; 45) ![\left[ \left( \cfrac{-2~}{5} \right)^{-3} \right]^{-2}](/wikipedia/images/math/1/5/4/154452bc7b4e3830e7474ee197167695.png) ; 46)
; 46) ![\left[ \left( \cfrac{-1~}{8} \right)^{-2} \right]^{-3}](/wikipedia/images/math/d/f/7/df755b98eccd3553b490053829676d47.png)
Escribe como varias potencias:
- 47)
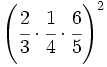
- 48)

- 49)

- 50)
![\left[ \cfrac{1}{3} \cdot \left( \cfrac{-2~}{5} \right) \cdot \left( \cfrac{-2~}{2} \right) \right]^2](/wikipedia/images/math/5/b/1/5b1a30cdfd3990559ce215699a00c2e3.png)
- 51)
![\left[ \cfrac{2}{3} \cdot \left( \cfrac{-5~}{4} \right) \cdot \cfrac{8}{5} \cdot \left( \cfrac{-5~}{2} \right) \right]^{-3}](/wikipedia/images/math/4/0/6/4066e6d3414c3e8d17a4b0e6837cad6a.png)
Escribe como una sola potencia:
- 52)
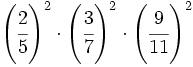
- 53)
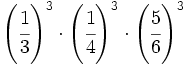
- 55)
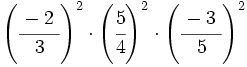
- 56)
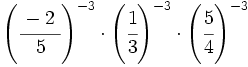
- 57)

- 58)
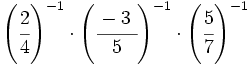
Simplifica:
- a)
![\left[\left(\cfrac{3}{5} \right)^{-1} \cdot \left(\cfrac{9}{25} \right)^2 \right]^3](/wikipedia/images/math/f/e/c/fec21991837955f9b9d945d4600cba18.png)
- b)
![\left[\cfrac{16}{9} \cdot \left(\cfrac{56}{27} \right)^{-1} \right] \cdot \left(\cfrac{14}{9} \right)^3 \cdot \left(\cfrac{7}{12} \right)^{-2}](/wikipedia/images/math/e/5/1/e5178ed20376c4ef7f07ca88d5d43975.png)
Simplifica 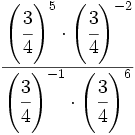
Simplifica: ![\left[ \left( \cfrac{1}{3} \right)^{10} : \left( \cfrac{1}{3} \right)^7 \right]^2](/wikipedia/images/math/6/9/1/6911b07dbbc54e802f87f7498ab77b09.png)
Cálculos con potencias dentro de fracciones:
Calcula:
- 59)

- 60)
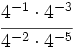
- 61)
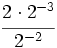
- 62)
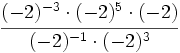
Calcula:
- 63)
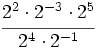
- 64)
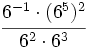
- 65)
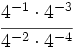
- 66)
![\cfrac{(-3)^{-2} \cdot (-3)^{-3}}{\left[ (-3)^2 \right]^{-2}}\;](/wikipedia/images/math/5/e/b/5eb0dbb75ab3ac5de8238f112e4d893f.png)
Simplifica: 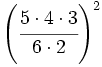
Simplifica: ![\left[ \cfrac{(8^2 \cdot 8) \cdot (6^7 \cdot 6)^2}{(8^3)^3 \cdot (6^3)^0 \cdot 6^3} \right]^3](/wikipedia/images/math/7/1/b/71b5b62cb54ed835ba335fdbca4de909.png)
Simplifica: 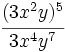
Simplifica: 
Simplifica y expresa la solución como una única potencia:
a) 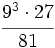
b) 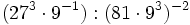
c) 
d) 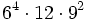
e) a) 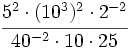
Simplifica y expresa la solución como una única potencia:
a) 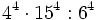
b) 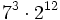
c) 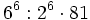
d) 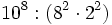
e) 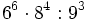
f) 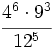
Simplifica:
- a)
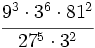
- b)
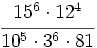
Simplifica:
- a)
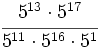
- b)
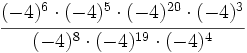
- c)
![\left[ \cfrac{( 2^3 \cdot 2^6)^{-2} \cdot (3^4)^3 \cdot 3 }{( 2^6 \cdot 2^{10})^{-1}\cdot (3^6 \cdot 3^2 \cdot 3^5)}\right]^{10}](/wikipedia/images/math/c/8/5/c85c926802bdbb7a51ef34eb65c6dda7.png)
Cálculos de diversos tipos:
Tutorial que explica la potencia de exponente entero (positivo y negativo) con fracciones y operaciones combinadas con multiplicación, división y potencias, trabajando la simplificación previa.
Exponente positivo:
- a)
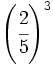 ; b)
; b)  ; c)
; c)  ; d)
; d) 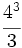 ; e)
; e) 
Exponente negativo:
- f)
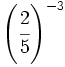 ; g)
; g) 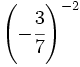 ; h)
; h)  ; i)
; i) 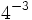 ; j)
; j) 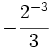
Operaciones combinadas:
- k)
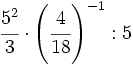 ; l)
; l)