Plantilla:Radicales (ampliación)
De Wikipedia
| Revisión de 07:38 10 sep 2017 Coordinador (Discusión | contribuciones) (→Producto y cocientes de radicales con distinto índice) ← Ir a diferencia anterior |
Revisión actual Coordinador (Discusión | contribuciones) (→Extracción de factores) |
||
| Línea 6: | Línea 6: | ||
| |duracion=19'38" | |duracion=19'38" | ||
| |sinopsis=Tutorial que explica cómo extraer factores de un radical, que se utiliza principalmente para simplificar radicales, y de cómo introducir factores dentro. | |sinopsis=Tutorial que explica cómo extraer factores de un radical, que se utiliza principalmente para simplificar radicales, y de cómo introducir factores dentro. | ||
| - | |url1=https://www.youtube.com/watch?v=b9mYVF3HOIU&index=3&list=PLZNmE9BEzVImIKACrwlnJVOz_w7oxAoRy | + | |url1=http://www.youtube.com/watch?v=b9mYVF3HOIU&index=3&list=PLZNmE9BEzVImIKACrwlnJVOz_w7oxAoRy |
| }} | }} | ||
| {{p}} | {{p}} | ||
| Línea 36: | Línea 36: | ||
| |sol= | |sol= | ||
| <math>\sqrt[3]{6000}=\sqrt[3]{2^4 \cdot 3 \cdot 5^3}=2 \cdot 5 \sqrt[3]{2 \cdot 3}=10\sqrt[3]{6}</math> | <math>\sqrt[3]{6000}=\sqrt[3]{2^4 \cdot 3 \cdot 5^3}=2 \cdot 5 \sqrt[3]{2 \cdot 3}=10\sqrt[3]{6}</math> | ||
| - | }} | ||
| - | {{p}} | ||
| - | {{AI_descartes|titulo1=Ejemplos: ''Extracción de factores de un radical'' | ||
| - | |descripcion=Pulsa el botón "Ejemplo" para ver los ejemplos. Anota algunos en tu cuaderno. | ||
| - | |||
| - | <center><iframe> | ||
| - | url=http://maralboran.org/web_ma/descartes/3_eso/Radicales/radicales3_1.html | ||
| - | width=700 | ||
| - | height=210 | ||
| - | name=myframe | ||
| - | </iframe></center> | ||
| - | |||
| - | |url1=http://maralboran.org/web_ma/descartes/3_eso/Radicales/radicales3_1.html | ||
| }} | }} | ||
| {{p}} | {{p}} | ||
| {{Videotutoriales|titulo=Extracción de factores de un radical|enunciado= | {{Videotutoriales|titulo=Extracción de factores de un radical|enunciado= | ||
| {{Video_enlace_tutomate | {{Video_enlace_tutomate | ||
| - | |titulo1=Tutorial | + | |titulo1=Tutorial 1 |
| |duracion=7'06" | |duracion=7'06" | ||
| |sinopsis=Extracción de factores de un radical. Ejemplos. | |sinopsis=Extracción de factores de un radical. Ejemplos. | ||
| |url1=https://www.youtube.com/watch?v=W-c4eVRUrVE&list=PLWRbPOo5oaTcOZhRaF3-DuT9bjIDrP8ZB&index=11 | |url1=https://www.youtube.com/watch?v=W-c4eVRUrVE&list=PLWRbPOo5oaTcOZhRaF3-DuT9bjIDrP8ZB&index=11 | ||
| + | }} | ||
| + | {{Video_enlace_pildoras | ||
| + | |titulo1=Tutorial 2 | ||
| + | |duracion=8'02" | ||
| + | |sinopsis=Extracción de factores de un radical utilizando un símil curioso. | ||
| + | |url1=https://youtu.be/dlw9p4Qu8L0?list=PLwCiNw1sXMSC9n7yjv8UoDKiokY6LF84S | ||
| + | }} | ||
| + | {{Video_enlace_escuela | ||
| + | |titulo1=Tutorial 3 | ||
| + | |duracion=5´50" | ||
| + | |sinopsis=Extracción de factores de un radical. Ejemplos | ||
| + | |url1=http://www.youtube.com/watch?v=nkEQ382ueoI&list=PLw7Z_p6_h3owuqG2cbSRKduUPNpu4q7i9&index=1&t=26m58s | ||
| + | }} | ||
| + | ---- | ||
| + | {{Video_enlace_pildoras | ||
| + | |titulo1=Ejemplos | ||
| + | |duracion=6'54" | ||
| + | |sinopsis=Extracción de factores de un radical. | ||
| + | |url1=https://youtu.be/gw5pATs-jd0?list=PLwCiNw1sXMSC9n7yjv8UoDKiokY6LF84S | ||
| }} | }} | ||
| ---- | ---- | ||
| Línea 66: | Línea 72: | ||
| a) <math>\sqrt{8}\;</math>{{b4}}{{b4}}b) <math>\sqrt{45}\;</math> | a) <math>\sqrt{8}\;</math>{{b4}}{{b4}}b) <math>\sqrt{45}\;</math> | ||
| - | |url1=https://www.youtube.com/watch?v=xXGo_UyXs14&index=12&list=PL9SnRnlzoyX3PUSesWagsxJdOfANgK_LO | + | |url1=http://www.youtube.com/watch?v=xXGo_UyXs14&index=12&list=PL9SnRnlzoyX3PUSesWagsxJdOfANgK_LO |
| }} | }} | ||
| {{Video_enlace_matefacil | {{Video_enlace_matefacil | ||
| Línea 75: | Línea 81: | ||
| a) <math>\sqrt{20}\;</math>{{b4}}{{b4}}b) <math>\sqrt{180}\;</math> | a) <math>\sqrt{20}\;</math>{{b4}}{{b4}}b) <math>\sqrt{180}\;</math> | ||
| - | |url1=https://www.youtube.com/watch?v=JNQFeq4gTog&index=13&list=PL9SnRnlzoyX3PUSesWagsxJdOfANgK_LO | + | |url1=http://www.youtube.com/watch?v=JNQFeq4gTog&index=13&list=PL9SnRnlzoyX3PUSesWagsxJdOfANgK_LO |
| }} | }} | ||
| {{Video_enlace_matefacil | {{Video_enlace_matefacil | ||
| Línea 84: | Línea 90: | ||
| a) <math>\sqrt[3]{16}\;</math>{{b4}}{{b4}}b) <math>\sqrt[3]{72}\;</math> | a) <math>\sqrt[3]{16}\;</math>{{b4}}{{b4}}b) <math>\sqrt[3]{72}\;</math> | ||
| - | |url1=https://www.youtube.com/watch?v=ndB2lsT8yew&index=14&list=PL9SnRnlzoyX3PUSesWagsxJdOfANgK_LO | + | |url1=http://www.youtube.com/watch?v=ndB2lsT8yew&index=14&list=PL9SnRnlzoyX3PUSesWagsxJdOfANgK_LO |
| }} | }} | ||
| {{Video_enlace_matefacil | {{Video_enlace_matefacil | ||
| Línea 93: | Línea 99: | ||
| a) <math>\sqrt[5]{96}\;</math>{{b4}}{{b4}}b) <math>\sqrt[4]{405}\;</math> | a) <math>\sqrt[5]{96}\;</math>{{b4}}{{b4}}b) <math>\sqrt[4]{405}\;</math> | ||
| - | |url1=https://www.youtube.com/watch?v=b1qRKXheEfE&list=PL9SnRnlzoyX3PUSesWagsxJdOfANgK_LO&index=15 | + | |url1=http://www.youtube.com/watch?v=b1qRKXheEfE&list=PL9SnRnlzoyX3PUSesWagsxJdOfANgK_LO&index=15 |
| }} | }} | ||
| {{Video_enlace_abel | {{Video_enlace_abel | ||
| Línea 102: | Línea 108: | ||
| a) <math>\sqrt{72}\;</math>{{b4}}{{b4}}b) <math>\sqrt[4]{3645}\;</math>{{b4}}{{b4}}c) <math>\sqrt{3240}\;</math>{{b4}}{{b4}}c) <math>5\,\sqrt[3]{384}\;</math> | a) <math>\sqrt{72}\;</math>{{b4}}{{b4}}b) <math>\sqrt[4]{3645}\;</math>{{b4}}{{b4}}c) <math>\sqrt{3240}\;</math>{{b4}}{{b4}}c) <math>5\,\sqrt[3]{384}\;</math> | ||
| - | |url1=https://www.youtube.com/watch?v=Rz-wClV7awM | + | |url1=http://www.youtube.com/watch?v=Rz-wClV7awM |
| }} | }} | ||
| - | |celda2= | ||
| {{Video_enlace_abel | {{Video_enlace_abel | ||
| |titulo1=Ejercicio 6 | |titulo1=Ejercicio 6 | ||
| Línea 112: | Línea 117: | ||
| a) <math>\sqrt{486}\;</math>{{b4}}{{b4}}b) <math>6\sqrt[3]{1250}\;</math>{{b4}}{{b4}}c) <math>m^3n\sqrt[5]{m^{20}n^{37}}\;</math> | a) <math>\sqrt{486}\;</math>{{b4}}{{b4}}b) <math>6\sqrt[3]{1250}\;</math>{{b4}}{{b4}}c) <math>m^3n\sqrt[5]{m^{20}n^{37}}\;</math> | ||
| - | |url1=https://www.youtube.com/watch?v=59aA7tS2-Ek | + | |url1=http://www.youtube.com/watch?v=59aA7tS2-Ek |
| }} | }} | ||
| {{Video_enlace_julioprofe | {{Video_enlace_julioprofe | ||
| Línea 118: | Línea 123: | ||
| |duracion=3'40" | |duracion=3'40" | ||
| |sinopsis=Simplifica: <math>-2\sqrt [3]{16x^5yz^9}</math> | |sinopsis=Simplifica: <math>-2\sqrt [3]{16x^5yz^9}</math> | ||
| - | |url1=https://www.youtube.com/watch?v=yQmn2rmM_t8&list=PL9B9AC3136D2D4C45&index=78 | + | |url1=http://www.youtube.com/watch?v=yQmn2rmM_t8&list=PL9B9AC3136D2D4C45&index=78 |
| }} | }} | ||
| {{Video_enlace_julioprofe | {{Video_enlace_julioprofe | ||
| Línea 124: | Línea 129: | ||
| |duracion=4'12" | |duracion=4'12" | ||
| |sinopsis=Simplifica: <math>\sqrt [4]{32x^4y^{21}z^{43}}</math> | |sinopsis=Simplifica: <math>\sqrt [4]{32x^4y^{21}z^{43}}</math> | ||
| - | |url1=https://www.youtube.com/watch?v=duwojO7exaY&list=PL9B9AC3136D2D4C45&index=79 | + | |url1=http://www.youtube.com/watch?v=duwojO7exaY&list=PL9B9AC3136D2D4C45&index=79 |
| }} | }} | ||
| {{Video_enlace_julioprofe | {{Video_enlace_julioprofe | ||
| Línea 130: | Línea 135: | ||
| |duracion=2'54" | |duracion=2'54" | ||
| |sinopsis=Simplifica: <math>\sqrt [5]{32x^5y^{-10}z^{-35}}</math> | |sinopsis=Simplifica: <math>\sqrt [5]{32x^5y^{-10}z^{-35}}</math> | ||
| - | |url1=https://www.youtube.com/watch?v=uq875zmaWxM&list=PL9B9AC3136D2D4C45&index=80}} | + | |url1=http://www.youtube.com/watch?v=uq875zmaWxM&list=PL9B9AC3136D2D4C45&index=80}} |
| + | |celda2= | ||
| {{Video_enlace_julioprofe | {{Video_enlace_julioprofe | ||
| |titulo1=Ejercicio 10 | |titulo1=Ejercicio 10 | ||
| |duracion=2'03" | |duracion=2'03" | ||
| |sinopsis=Simplifica: <math>\sqrt [5]{-243a^{20}}</math> | |sinopsis=Simplifica: <math>\sqrt [5]{-243a^{20}}</math> | ||
| - | |url1=https://www.youtube.com/watch?v=Ic979EMoC24&list=PL9B9AC3136D2D4C45&index=81 | + | |url1=http://www.youtube.com/watch?v=Ic979EMoC24&list=PL9B9AC3136D2D4C45&index=81 |
| + | }} | ||
| + | {{Video_enlace_escuela | ||
| + | |titulo1=Ejercicio 11a | ||
| + | |duracion=12´16" | ||
| + | |sinopsis= | ||
| + | |||
| + | Extrae todos los factores posibles del radicando: | ||
| + | |||
| + | :a) <math>\sqrt{12}\;</math>; {{b4}} b) <math>\sqrt{45}\;</math>; {{b4}} c) <math>\sqrt{400}\;</math>; {{b4}} | ||
| + | :d) <math>\sqrt{18}\;</math>; {{b4}} e) <math>\sqrt{128}\;</math> | ||
| + | |||
| + | |url1=http://www.youtube.com/watch?v=6DjUvSGLVOs&index=4&list=PLw7Z_p6_h3owuqG2cbSRKduUPNpu4q7i9&t=19m41s | ||
| + | }} | ||
| + | {{Video_enlace_escuela | ||
| + | |titulo1=Ejercicio 11b | ||
| + | |duracion=22´39" | ||
| + | |sinopsis= | ||
| + | |||
| + | Extrae todos los factores posibles del radicando: | ||
| + | |||
| + | :f) <math>\sqrt{216}\;</math> ; {{b4}} g) <math>\sqrt{1080}\;</math> ; {{b4}} h) <math>\sqrt[3]{625}\;</math> | ||
| + | |||
| + | :i) <math>\sqrt[3]{432}\;</math> ;{{b4}} j) <math>\sqrt[3]{-216}\;</math> ; {{b4}} k) <math>\sqrt[3]{243}\;</math> | ||
| + | |||
| + | :l) <math>\sqrt[4]{1296}\;</math> ; {{b4}} m) <math>\sqrt[4]{20736}\;</math> ; n) <math>\sqrt[5]{-480}\;</math> | ||
| + | |||
| + | |url1=http://www.youtube.com/watch?v=X5o7qfTSeB0&index=5&list=PLw7Z_p6_h3owuqG2cbSRKduUPNpu4q7i9 | ||
| + | }} | ||
| + | {{Video_enlace_khan | ||
| + | |titulo1=Ejercicio 12 | ||
| + | |duracion=6´54" | ||
| + | |sinopsis=Simplifica: <math>7\,\sqrt{117}\;</math> | ||
| + | |||
| + | |url1=http://youtu.be/43QoIMdkTx0 | ||
| + | }} | ||
| + | {{Video_enlace_khan | ||
| + | |titulo1=Ejercicio 13 | ||
| + | |duracion=4´20" | ||
| + | |sinopsis=Simplifica: <math>3\,\sqrt{500x^3}\;</math> | ||
| + | |||
| + | |url1=http://youtu.be/w7cwun6MgJ0 | ||
| + | }} | ||
| + | {{Video_enlace_khan | ||
| + | |titulo1=Ejercicio 14 | ||
| + | |duracion=7´30" | ||
| + | |sinopsis=Simplifica: | ||
| + | |||
| + | :a) <math>2\,\sqrt{7x} \cdot 3\,\sqrt{14x^2}\;</math> | ||
| + | |||
| + | :b) <math>\sqrt{2a} \cdot \sqrt{14a^3} \cdot \sqrt{5a}\;</math> | ||
| + | |||
| + | |url1=http://youtu.be/qC8wFJ7CTzo | ||
| + | }} | ||
| + | {{Video_enlace_khan | ||
| + | |titulo1=Ejercicio 15 | ||
| + | |duracion=4´27" | ||
| + | |sinopsis=Simplifica: <math>5\,\sqrt[3]{2x^2} \cdot 3\,\sqrt[3]{4x^4}\;</math> | ||
| + | |||
| + | |url1=http://youtu.be/VJbrRh8pVWI | ||
| + | }} | ||
| + | {{Video_enlace_khan | ||
| + | |titulo1=Ejercicio 16 | ||
| + | |duracion=2´17" | ||
| + | |sinopsis=Simplifica: <math>\sqrt[3]{125x^6y^3}</math> | ||
| + | |||
| + | |url1=http://youtu.be/P4K7VQ_5aHY | ||
| + | }} | ||
| + | {{Video_enlace_khan | ||
| + | |titulo1=Ejercicio 17 | ||
| + | |duracion=2´25" | ||
| + | |sinopsis=Simplifica: <math>\sqrt[4]{5a^4b^{12}}</math> | ||
| + | |||
| + | |url1=http://youtu.be/ffXymNPditI | ||
| + | }} | ||
| + | }} | ||
| + | }} | ||
| + | {{Actividades|titulo=Extracción de factores de un radical|enunciado= | ||
| + | {{AI_descartes|titulo1=Actividad 1 | ||
| + | |descripcion=Pulsa el botón "Ejemplo" para ver los ejemplos. Anota algunos en tu cuaderno. | ||
| + | |||
| + | <center><iframe> | ||
| + | url=http://maralboran.org/web_ma/descartes/3_eso/Radicales/radicales3_1.html | ||
| + | width=700 | ||
| + | height=210 | ||
| + | name=myframe | ||
| + | </iframe></center> | ||
| + | |||
| + | |url1=http://maralboran.org/web_ma/descartes/3_eso/Radicales/radicales3_1.html | ||
| + | }} | ||
| + | {{AI_Khan | ||
| + | |titulo1=Actividad 2 | ||
| + | |descripcion=Extrae factores fuera del radical. | ||
| + | |url1=http://es.khanacademy.org/math/algebra/rational-exponents-and-radicals/alg1-simplify-square-roots/a/simplifying-square-roots-review | ||
| + | }} | ||
| + | {{AI_Khan | ||
| + | |titulo1=Autoevaluación 1 | ||
| + | |descripcion=Extrae factores fuera del radical. | ||
| + | |url1=http://es.khanacademy.org/math/pre-algebra/pre-algebra-exponents-radicals/pre-algebra-square-roots/e/simplifying_radicals | ||
| + | }} | ||
| + | {{AI_Khan | ||
| + | |titulo1=Autoevaluación 1b | ||
| + | |descripcion=Extrae factores fuera del radical (con variables). | ||
| + | |url1=http://es.khanacademy.org/math/algebra/rational-exponents-and-radicals/alg1-simplify-square-roots/e/multiplying_radicals | ||
| }} | }} | ||
| + | {{AI_Khan | ||
| + | |titulo1=Autoevaluación 1c | ||
| + | |descripcion=Extrae factores fuera del radical (con variables). | ||
| + | |url1=http://es.khanacademy.org/math/algebra/rational-exponents-and-radicals/alg1-simplify-square-roots/e/adding_and_subtracting_radicals | ||
| }} | }} | ||
| }} | }} | ||
| Línea 179: | Línea 292: | ||
| Para introducir un factor dentro de un radical, éste se eleva al índice del radical y el resultado se multiplica por el radicando del radical. De esta manera, y teniendo en cuenta las propiedades de las operaciones con potencias, para introducir una potencia dentro de un radical multiplicaremos el exponente de la potencia por el índice del radical. La potencia resultante pasará dentro del radical multiplicando al radicando. | Para introducir un factor dentro de un radical, éste se eleva al índice del radical y el resultado se multiplica por el radicando del radical. De esta manera, y teniendo en cuenta las propiedades de las operaciones con potencias, para introducir una potencia dentro de un radical multiplicaremos el exponente de la potencia por el índice del radical. La potencia resultante pasará dentro del radical multiplicando al radicando. | ||
| - | |url1=https://www.youtube.com/watch?v=JgqVCsGy6wI | + | |url1=http://www.youtube.com/watch?v=JgqVCsGy6wI |
| }} | }} | ||
| {{Video_enlace_abel | {{Video_enlace_abel | ||
| Línea 187: | Línea 300: | ||
| Para introducir un factor dentro de un radical, éste se eleva al índice del radical y el resultado se multiplica por el radicando del radical. De esta manera, y teniendo en cuenta las propiedades de las operaciones con potencias, para introducir una potencia dentro de un radical multiplicaremos el exponente de la potencia por el índice del radical. La potencia resultante pasará dentro del radical multiplicando al radicando. | Para introducir un factor dentro de un radical, éste se eleva al índice del radical y el resultado se multiplica por el radicando del radical. De esta manera, y teniendo en cuenta las propiedades de las operaciones con potencias, para introducir una potencia dentro de un radical multiplicaremos el exponente de la potencia por el índice del radical. La potencia resultante pasará dentro del radical multiplicando al radicando. | ||
| - | |url1=https://www.youtube.com/watch?v=1xsJdLV2YvA | + | |url1=http://www.youtube.com/watch?v=1xsJdLV2YvA |
| }} | }} | ||
| {{Video_enlace_abel | {{Video_enlace_abel | ||
| Línea 195: | Línea 308: | ||
| Para introducir un factor dentro de un radical, éste se eleva al índice del radical y el resultado se multiplica por el radicando del radical. De esta manera, y teniendo en cuenta las propiedades de las operaciones con potencias, para introducir una potencia dentro de un radical multiplicaremos el exponente de la potencia por el índice del radical. La potencia resultante pasará dentro del radical multiplicando al radicando. Si dentro del radical tenemos otra potencia con la misma base entonces sumaremos el exponente de la potencia que entra con el de dentro del radical. | Para introducir un factor dentro de un radical, éste se eleva al índice del radical y el resultado se multiplica por el radicando del radical. De esta manera, y teniendo en cuenta las propiedades de las operaciones con potencias, para introducir una potencia dentro de un radical multiplicaremos el exponente de la potencia por el índice del radical. La potencia resultante pasará dentro del radical multiplicando al radicando. Si dentro del radical tenemos otra potencia con la misma base entonces sumaremos el exponente de la potencia que entra con el de dentro del radical. | ||
| - | |url1=https://www.youtube.com/watch?v=rEljk_OzcY0 | + | |url1=http://www.youtube.com/watch?v=rEljk_OzcY0 |
| }} | }} | ||
| {{Video_enlace_abel | {{Video_enlace_abel | ||
| Línea 208: | Línea 321: | ||
| :b) <math>a^7 \cdot b^8 \sqrt[5]{b^2}</math> | :b) <math>a^7 \cdot b^8 \sqrt[5]{b^2}</math> | ||
| - | |url1=https://www.youtube.com/watch?v=2QIY0WaX5C8 | + | |url1=http://www.youtube.com/watch?v=2QIY0WaX5C8 |
| }} | }} | ||
| }} | }} | ||
| Línea 223: | Línea 336: | ||
| |url1=http://maralboran.org/web_ma/descartes/3_eso/Radicales/radicales3_3.html | |url1=http://maralboran.org/web_ma/descartes/3_eso/Radicales/radicales3_3.html | ||
| + | }} | ||
| + | {{AI_vitutor | ||
| + | |titulo1=Autoevaluación: ''Introducción y extracción de factores de un radical'' | ||
| + | |descripcion=Ejercicios de autoevaluación sobre introducción y extracción de factores de un radical. | ||
| + | |url1=http://www.vitutor.com/di/re/r11e.html | ||
| }} | }} | ||
| {{p}} | {{p}} | ||
| Línea 255: | Línea 373: | ||
| |duracion=10'09" | |duracion=10'09" | ||
| |sinopsis=Tutorial que explica cómo sumar y restar radicales. La suma y resta son operaciones que "se llevan muy mal" con el resto de operaciones y hay que tener mucho cuidado a la hora de hacerlo con radicales. | |sinopsis=Tutorial que explica cómo sumar y restar radicales. La suma y resta son operaciones que "se llevan muy mal" con el resto de operaciones y hay que tener mucho cuidado a la hora de hacerlo con radicales. | ||
| - | |url1=https://www.youtube.com/watch?v=sKqcSF0xisc&index=5&list=PLZNmE9BEzVImIKACrwlnJVOz_w7oxAoRy | + | |url1=http://www.youtube.com/watch?v=sKqcSF0xisc&index=5&list=PLZNmE9BEzVImIKACrwlnJVOz_w7oxAoRy |
| }} | }} | ||
| {{Video_enlace_matefacil | {{Video_enlace_matefacil | ||
| Línea 261: | Línea 379: | ||
| |duracion=4'58" | |duracion=4'58" | ||
| |sinopsis=Suma y resta de radicales con el mismo índice. Ejemplos. | |sinopsis=Suma y resta de radicales con el mismo índice. Ejemplos. | ||
| - | |url1=https://www.youtube.com/watch?v=zlR_Pq5BWME&index=11&list=PL9SnRnlzoyX3PUSesWagsxJdOfANgK_LO | + | |url1=http://www.youtube.com/watch?v=zlR_Pq5BWME&index=11&list=PL9SnRnlzoyX3PUSesWagsxJdOfANgK_LO |
| }} | }} | ||
| ---- | ---- | ||
| Línea 268: | Línea 386: | ||
| |duracion=3'38" | |duracion=3'38" | ||
| |sinopsis=Simplifica: <math>\sqrt{18}+\sqrt{50}-\sqrt{2}-\sqrt{8}</math> | |sinopsis=Simplifica: <math>\sqrt{18}+\sqrt{50}-\sqrt{2}-\sqrt{8}</math> | ||
| - | |url1=https://www.youtube.com/watch?v=i_Tf9tSby2M&list=PL9B9AC3136D2D4C45&index=83 | + | |url1=http://www.youtube.com/watch?v=i_Tf9tSby2M&list=PL9B9AC3136D2D4C45&index=83 |
| }} | }} | ||
| {{Video_enlace_julioprofe | {{Video_enlace_julioprofe | ||
| Línea 274: | Línea 392: | ||
| |duracion=6'11" | |duracion=6'11" | ||
| |sinopsis=Simplifica: <math>\sqrt{32}+\sqrt{243}-\sqrt{12}-\sqrt{48}-\sqrt{27}</math> | |sinopsis=Simplifica: <math>\sqrt{32}+\sqrt{243}-\sqrt{12}-\sqrt{48}-\sqrt{27}</math> | ||
| - | |url1=https://www.youtube.com/watch?v=yRRZZu_TUGw&list=PL9B9AC3136D2D4C45&index=84 | + | |url1=http://www.youtube.com/watch?v=yRRZZu_TUGw&list=PL9B9AC3136D2D4C45&index=84 |
| + | }} | ||
| + | {{Video_enlace_escuela | ||
| + | |titulo1=Ejercicio 3 | ||
| + | |duracion=30'30" | ||
| + | |sinopsis=Calcula: | ||
| + | |||
| + | :a) <math>\sqrt{50}+2\sqrt{18}-3\sqrt{32}\;</math> | ||
| + | :b) <math>-\sqrt{12}+\sqrt{75}-\sqrt{27}\;</math> | ||
| + | :c) <math>\sqrt{20}+4\sqrt{8}-5\sqrt{50}\;</math> | ||
| + | :d) <math>\sqrt[3]{48}+\sqrt[3]{1080}-\sqrt[3]{96}\;</math> | ||
| + | :e) <math>-\sqrt[3]{-27}+2\sqrt[3]{272}-9\sqrt[3]{-81}\;</math> | ||
| + | |||
| + | |url1=http://www.youtube.com/watch?v=p1xJ0l7x0Tw&index=4&list=PLw7Z_p6_h3ozxW7jq_j3xSPsocWGGn25o | ||
| + | }} | ||
| + | {{Video_enlace_escuela | ||
| + | |titulo1=Ejercicio 4 | ||
| + | |duracion=12'36" | ||
| + | |sinopsis= | ||
| + | Calcula: | ||
| + | |||
| + | :a) <math>\sqrt[4]{32}+8\sqrt[4]{16}-\sqrt[4]{80}\;</math> | ||
| + | :b) <math>\sqrt[5]{64}-6\sqrt[5]{32}+5\sqrt[5]{96}\;</math> | ||
| + | :c) <math>-9\sqrt[6]{64}+\sqrt[6]{192}-\sqrt[6]{960}\;</math> | ||
| + | |||
| + | |url1=http://www.youtube.com/watch?v=ZQID-b3oTsc&list=PLw7Z_p6_h3ozxW7jq_j3xSPsocWGGn25o&index=5 | ||
| }} | }} | ||
| }} | }} | ||
| Línea 311: | Línea 454: | ||
| }} | }} | ||
| {{p}} | {{p}} | ||
| - | |||
| - | |||
| ===Producto y cocientes de radicales con distinto índice=== | ===Producto y cocientes de radicales con distinto índice=== | ||
| Línea 333: | Línea 474: | ||
| <math>\sqrt[12]{10^4 \cdot 5^3 : 8^6}=\sqrt[12]{2^4 \cdot 5^4 \cdot 5^3 : (2^3)^6}=\sqrt[12]{2^{-14} \cdot 5^7}</math> | <math>\sqrt[12]{10^4 \cdot 5^3 : 8^6}=\sqrt[12]{2^4 \cdot 5^4 \cdot 5^3 : (2^3)^6}=\sqrt[12]{2^{-14} \cdot 5^7}</math> | ||
| - | }} | ||
| - | {{p}} | ||
| - | {{AI_descartes|titulo1=''Producto y cocientes de radicales con distinto índice | ||
| - | |descripcion=Actividades en las que podrás aprender a multiplicar y dividir radicales de distinto índice previa reducción a índice común. | ||
| - | |url1=http://maralboran.org/web_ma/descartes/3_eso/Radicales/radicales6.htm | ||
| }} | }} | ||
| {{p}} | {{p}} | ||
| Línea 345: | Línea 481: | ||
| |duracion=7'21" | |duracion=7'21" | ||
| |sinopsis=Producto y cociente de radicales con el mismo o con distinto índice. Ejemplos. | |sinopsis=Producto y cociente de radicales con el mismo o con distinto índice. Ejemplos. | ||
| - | |url1=https://www.youtube.com/watch?v=avy7Me0Vi1I&index=13&list=PLWRbPOo5oaTcOZhRaF3-DuT9bjIDrP8ZB | + | |url1=http://www.youtube.com/watch?v=avy7Me0Vi1I&index=13&list=PLWRbPOo5oaTcOZhRaF3-DuT9bjIDrP8ZB |
| }} | }} | ||
| ---- | ---- | ||
| Línea 352: | Línea 488: | ||
| |duracion=4'43" | |duracion=4'43" | ||
| |sinopsis=Simplifica: <math>(8\sqrt[3]{a^2b}) \cdot (4\sqrt{ab^3})</math> | |sinopsis=Simplifica: <math>(8\sqrt[3]{a^2b}) \cdot (4\sqrt{ab^3})</math> | ||
| - | |url1=https://www.youtube.com/watch?v=z6WUqTVeJZU&index=86&list=PL9B9AC3136D2D4C45 | + | |url1=http://www.youtube.com/watch?v=z6WUqTVeJZU&index=86&list=PL9B9AC3136D2D4C45 |
| }} | }} | ||
| {{Video_enlace_julioprofe | {{Video_enlace_julioprofe | ||
| Línea 358: | Línea 494: | ||
| |duracion=3'50" | |duracion=3'50" | ||
| |sinopsis=Simplifica: <math>\sqrt[3]{27^{-2}} : \sqrt[4]{16}</math> | |sinopsis=Simplifica: <math>\sqrt[3]{27^{-2}} : \sqrt[4]{16}</math> | ||
| - | |url1=https://www.youtube.com/watch?v=2UQmqhWkYPc&list=PL9B9AC3136D2D4C45&index=87}} | + | |url1=http://www.youtube.com/watch?v=2UQmqhWkYPc&list=PL9B9AC3136D2D4C45&index=87}} |
| + | }} | ||
| + | {{Actividades|titulo=Producto y cocientes de radicales|enunciado= | ||
| + | {{AI_descartes|titulo1=''Producto y cociente de radicales con distinto índice | ||
| + | |descripcion=Actividades en las que podrás aprender a multiplicar y dividir radicales de distinto índice previa reducción a índice común. | ||
| + | |url1=http://maralboran.org/web_ma/descartes/3_eso/Radicales/radicales6.htm | ||
| + | }} | ||
| + | {{AI_vitutor | ||
| + | |titulo1=Autoevaluación: ''Producto de radicales'' | ||
| + | |descripcion=Ejercicios de autoevaluación sobre productos de radicales. | ||
| + | |url1=http://www.vitutor.com/di/re/r13e.html | ||
| + | }} | ||
| + | {{AI_vitutor | ||
| + | |titulo1=Autoevaluación: ''Cociente de radicales'' | ||
| + | |descripcion=Ejercicios de autoevaluación sobre cocientes de radicales. | ||
| + | |url1=http://www.vitutor.com/di/re/r14e.html | ||
| + | }} | ||
| }} | }} | ||
| {{p}} | {{p}} | ||
| Línea 367: | Línea 519: | ||
| |duracion=6'31" | |duracion=6'31" | ||
| |sinopsis=Potencias de radicales. Ejemplos. | |sinopsis=Potencias de radicales. Ejemplos. | ||
| - | |url1=https://www.youtube.com/watch?v=cslLXyMyd0E&index=14&list=PLWRbPOo5oaTcOZhRaF3-DuT9bjIDrP8ZB | + | |url1=http://www.youtube.com/watch?v=cslLXyMyd0E&index=14&list=PLWRbPOo5oaTcOZhRaF3-DuT9bjIDrP8ZB |
| + | }} | ||
| + | {{AI_vitutor | ||
| + | |titulo1=Autoevaluación: ''Potencias de radicales'' | ||
| + | |descripcion=Ejercicios de autoevaluación sobre potencias de radicales. | ||
| + | |url1=http://www.vitutor.com/di/re/r15e.html | ||
| }} | }} | ||
| {{p}} | {{p}} | ||
| - | ===Radicales dobles=== | + | ===Radicales dobles (Avanzado)=== |
| {{Videotutoriales|titulo=Radicales dobles|enunciado= | {{Videotutoriales|titulo=Radicales dobles|enunciado= | ||
| {{Video_enlace_abel | {{Video_enlace_abel | ||
| Línea 402: | Línea 559: | ||
| }} | }} | ||
| - | ===Actividades con radicales=== | + | ===Actividades=== |
| {{Videotutoriales|titulo=Operaciones con radicales|enunciado= | {{Videotutoriales|titulo=Operaciones con radicales|enunciado= | ||
| {{Video_enlace_unicoos | {{Video_enlace_unicoos | ||
| Línea 495: | Línea 652: | ||
| |url1=https://www.youtube.com/watch?v=7ORaozkauxg&list=PLWRbPOo5oaTcOZhRaF3-DuT9bjIDrP8ZB&index=12 | |url1=https://www.youtube.com/watch?v=7ORaozkauxg&list=PLWRbPOo5oaTcOZhRaF3-DuT9bjIDrP8ZB&index=12 | ||
| }} | }} | ||
| + | {{Video_enlace_escuela | ||
| + | |titulo1=Ejercicio 10 | ||
| + | |duracion=19'33" | ||
| + | |sinopsis=Calcula y simplifica: | ||
| + | :a) <math>2\sqrt{25} \cdot 3\sqrt{63}\;</math> ; {{b4}} b) <math>3\sqrt{25} \cdot 2\sqrt{18}\;</math> ; {{b4}} c) <math>3\sqrt{30} \cdot 5\sqrt{6}\;</math> | ||
| + | :d) <math>-2\sqrt{63}-5\sqrt{35}\;</math> ; {{b4}} e) <math>8\sqrt{70} \cdot (-2)\sqrt{35}\;</math> ; {{b4}} f) <math>3\sqrt{21} \cdot (-7\sqrt{7}) \cdot 4\sqrt{6}\;</math> | ||
| + | |||
| + | :g) <math>3\sqrt{55} \cdot (-3\sqrt{22}) \cdot (-4)\sqrt{5}\;</math> ; {{b4}} f) <math>-3\sqrt{15} \cdot (-7\sqrt{55}) \cdot \sqrt{3}\;</math> | ||
| + | |||
| + | |url1=https://www.youtube.com/watch?v=ZQID-b3oTsc&list=PLw7Z_p6_h3ozxW7jq_j3xSPsocWGGn25o&index=5&t=12m36s | ||
| + | }} | ||
| + | {{Video_enlace_escuela | ||
| + | |titulo1=Ejercicio 11 | ||
| + | |duracion=29'15" | ||
| + | |sinopsis=Opera y simplifica: | ||
| + | |||
| + | :a) <math>2\sqrt[3]{18} \cdot 3\sqrt[3]{12}\;</math> ; {{b4}} b) <math>3\sqrt[3]{50} \cdot 2\sqrt[3]{20}\;</math> ; {{b4}} c) <math>3\sqrt[3]{45} \cdot 5\sqrt[3]{75}\;</math> | ||
| + | |||
| + | :d) <math>-2\sqrt[3]{28} \cdot 5\sqrt[3]{98}\;</math> ; {{b4}} e) <math>8\sqrt[3]{-12} \cdot (-3\sqrt[3]{3}) \cdot \sqrt[3]{-6}\;</math> ; {{b4}} f) <math>3\sqrt[3]{8} \cdot (-7\sqrt[3]{9}) \cdot 4\sqrt[3]{3}\;</math> | ||
| + | |||
| + | :g) <math>-5\sqrt[3]{27} \cdot (-\sqrt[3]{4}) \cdot 4\sqrt[3]{2}\;</math> ; {{b4}} h) <math>3\sqrt[3]{20} \cdot (-3\sqrt[3]{10}) \cdot (-4\sqrt[3]{5})\;</math> ; {{b4}} i) <math>3\sqrt[6]{324} \cdot 5\sqrt[6]{144}\;</math> | ||
| + | |||
| + | :j) <math>-2\sqrt[4]{250} \cdot 5\sqrt[4]{40}\;</math> ; {{b4}} k) <math>3\sqrt[4]{88} \cdot (-7\sqrt[8]{216}) \cdot 4\sqrt[8]{162}\;</math> | ||
| + | |||
| + | Opera y simplifica: | ||
| + | |||
| + | :a) <math>\sqrt{72} : \sqrt{2}\;</math> ; {{b4}} b) <math>8\sqrt{200} : (-2\sqrt{2})\;</math> | ||
| + | |||
| + | |url1=https://www.youtube.com/watch?v=CBXgwtvoCko&index=6&list=PLw7Z_p6_h3ozxW7jq_j3xSPsocWGGn25o | ||
| + | }} | ||
| + | {{Video_enlace_escuela | ||
| + | |titulo1=Ejercicio 12 | ||
| + | |duracion=30'17" | ||
| + | |sinopsis=Opera y simplifica: | ||
| + | |||
| + | :a) <math>-10\sqrt{980} : 5\sqrt{5}\;</math> ; {{b4}} b) <math>6\sqrt{675} : (-\sqrt{3}) \;</math> {{b4}} c) <math>20\sqrt{3087} : 10\sqrt{7}\;</math> | ||
| + | |||
| + | :d) <math>9\sqrt[3]{432} : (-\sqrt[3]{-2})\;</math> ; {{b4}} e) <math>-10\sqrt[3]{1458} : 5\sqrt[3]{2}\;</math> ; {{b4}} f) <math>12\sqrt[3]{192} : (-6\sqrt[3]{-3})\;</math> | ||
| + | |||
| + | :g) <math>-30\sqrt[3]{3000} : 6\sqrt[3]{3}\;</math> ; {{b4}} h) <math>\sqrt[4]{512} : \sqrt[4]{2}\;</math> ; {{b4}} i) <math>9\sqrt[4]{768} : (-\sqrt[4]{3})\;</math> | ||
| + | |||
| + | :j) <math>-10\sqrt[4]{2592} : 5\sqrt[4]{2}\;</math> ; {{b4}} k) <math>12\sqrt[4]{3888} : (-6\sqrt[4]{3})\;</math> ; {{b4}} l) <math>-30\sqrt[4]{13122} : 6\sqrt[4]{2}\;</math> | ||
| + | |||
| + | Calcula: | ||
| + | |||
| + | :a) <math>(\sqrt{36})^3\;</math> ; {{b4}} b) <math>(\sqrt{5})^6\;</math> ; {{b4}} c) <math>(\sqrt{25})^4\;</math> ; {{b4}} d) <math>(\sqrt{18})^3\;</math> | ||
| + | |||
| + | |url1=https://www.youtube.com/watch?v=m6M7mBa27VY&list=PLw7Z_p6_h3ozxW7jq_j3xSPsocWGGn25o&index=7 | ||
| + | }} | ||
| + | {{Video_enlace_escuela | ||
| + | |titulo1=Ejercicio 13 | ||
| + | |duracion=19'53" | ||
| + | |sinopsis=Calcula: | ||
| + | |||
| + | :a) <math>(\sqrt[3]{-100})^4\;</math> ; {{b4}} b) <math>(\sqrt[3]{18})^4\;</math> ; {{b4}} c) <math>(\sqrt[4]{7})^5\;</math> ; {{b4}} d) <math>(\sqrt[4]{21})^7\;</math> | ||
| + | |||
| + | :e) <math>(\sqrt[4]{100})^5\;</math> ; {{b4}} f) <math>(\sqrt[5]{16})^7\;</math> ; {{b4}} g) <math>(\sqrt[6]{12})^7\;</math> | ||
| + | |||
| + | Calcula y simplifica: | ||
| + | |||
| + | :a) <math>\sqrt{\sqrt{1296}}\;</math> ; {{b4}} b) <math>\sqrt{\sqrt{256}}\;</math> ; {{b4}} c) <math>\sqrt[3]{\sqrt{46\,656}}\;</math> | ||
| + | |||
| + | :d) <math>\sqrt[3]{\sqrt[3]{4096}}\;</math> ; {{b4}} e) <math>\sqrt{\sqrt[3]{1024}}\;</math> ; {{b4}} f) <math>\sqrt[4]{\sqrt[3]{4096}}\;</math> | ||
| + | |url1=https://www.youtube.com/watch?v=xAScnfwBG-s&index=8&list=PLw7Z_p6_h3ozxW7jq_j3xSPsocWGGn25o | ||
| + | }} | ||
| }} | }} | ||
| {{p}} | {{p}} | ||
| {{Geogebra_enlace | {{Geogebra_enlace | ||
| |descripcion=En esta escena podrás practicar la suma y resta de radicales con o sin el mismo índice. | |descripcion=En esta escena podrás practicar la suma y resta de radicales con o sin el mismo índice. | ||
| - | |enlace=[https://ggbm.at/NJQsBUYx Ejercicios: Suma, resta y simplificación de radicales] | + | |enlace=[http://ggbm.at/NJQsBUYx Ejercicios: Suma, resta y simplificación de radicales] |
| + | }} | ||
| + | {{AI_vitutor | ||
| + | |titulo1=Autoevaluación: ''Suma y resta de radicales'' | ||
| + | |descripcion=Ejercicios de autoevaluación sobre sumas y restas de radicales. | ||
| + | |url1=http://www.vitutor.com/di/re/r12e.html | ||
| + | }} | ||
| + | {{AI_vitutor | ||
| + | |titulo1=Autoevaluación: ''Raíces de radicales'' | ||
| + | |descripcion=Ejercicios de autoevaluación sobre raíces de radicales. | ||
| + | |url1=http://www.vitutor.com/di/re/r16e.html | ||
| }} | }} | ||
Revisión actual
Tabla de contenidos |
Extracción e introducción de factores en un radical
El siguiente videotutorial resume lo que se va a a ver en este apartado:
Tutorial que explica cómo extraer factores de un radical, que se utiliza principalmente para simplificar radicales, y de cómo introducir factores dentro.
Extracción de factores
Procedimiento
Para extraer factores de un radical se divide el exponente (m) del factor entre el índice (n) del radical. A continuación, se saca el factor elevado al cociente (c) de la división, quedando dentro del radical el factor elevado al resto (r).
![\sqrt[n]{a^m}= a^c \cdot \sqrt[n]{a^r}](/wikipedia/images/math/5/e/c/5ecb8e4f6515ae774c6eef7fea5c576e.png)
Para la demostración transformaremos la expresión radical en potencias y aplicaremos las propiedades de las operaciones con potencias:
Fíjate que en (1) hemos usado la regla de la divsión:
Para extraer factores de un radical se divide el exponente entre el índice y se saca el factor elevado al cociente de la división quedando ese factor elevado al resto.
Ejemplo: Extracción de factores de un radical
Extrae todo lo que se pueda de este radical: ![\sqrt[3]{6000}](/wikipedia/images/math/5/c/2/5c28fba2f76ed7b33ea1b13e448c224f.png)
![\sqrt[3]{6000}=\sqrt[3]{2^4 \cdot 3 \cdot 5^3}=2 \cdot 5 \sqrt[3]{2 \cdot 3}=10\sqrt[3]{6}](/wikipedia/images/math/6/9/f/69fad43d23404b125d2e89b1f9479c74.png)
Extracción de factores de un radical. Ejemplos.
Extracción de factores de un radical utilizando un símil curioso.
Extracción de factores de un radical. Ejemplos
Extracción de factores de un radical.
Simplifica: a) Simplifica: a) Simplifica: a) Simplifica: a) Simplifica: a) Simplifica: a) Simplifica: Simplifica: Simplifica: | Simplifica: Extrae todos los factores posibles del radicando:
Extrae todos los factores posibles del radicando:
Simplifica: Simplifica: Simplifica:
Simplifica: Simplifica: Simplifica: |
Pulsa el botón "Ejemplo" para ver los ejemplos. Anota algunos en tu cuaderno.
Extrae factores fuera del radical.
Extrae factores fuera del radical.
Extrae factores fuera del radical (con variables).
Extrae factores fuera del radical (con variables).
Introducción de factores
Procedimiento
Para introducir un factor dentro de un radical, éste se eleva al índice del radical y el resultado se multiplica por el radicando del radical.
![a \sqrt[n]{b}= \sqrt[n]{a^n \cdot b}](/wikipedia/images/math/8/5/f/85f82704cfc0f9e1418d853a9e18061d.png)
Para la demostración transformaremos la expresión radical en potencias y aplicaremos las propiedades de las operaciones con potencias:
Ejemplo: Introducción de factores en un radical
Introduce los factores dentro del radical: ![10 \sqrt[3]{6}](/wikipedia/images/math/b/0/5/b059e301f3cf30968be2c858922be777.png)
![10 \sqrt[3]{6}=\sqrt[3]{6 \cdot 10^3}=\sqrt[3]{6000}](/wikipedia/images/math/f/c/a/fca24b7a66a69b8d0a52ef1d1f43ef96.png)
Pulsa el botón "Ejemplo" para ver los ejemplos. Anota algunos en tu cuaderno.
Introduce dentro del radical: 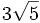
Para introducir un factor dentro de un radical, éste se eleva al índice del radical y el resultado se multiplica por el radicando del radical. De esta manera, y teniendo en cuenta las propiedades de las operaciones con potencias, para introducir una potencia dentro de un radical multiplicaremos el exponente de la potencia por el índice del radical. La potencia resultante pasará dentro del radical multiplicando al radicando.
Introduce los factores dentro del radical: ![a^3 b^2 \sqrt[4]{c}](/wikipedia/images/math/0/f/2/0f257d67a28e35c2fe66d6e47ec6170b.png)
Para introducir un factor dentro de un radical, éste se eleva al índice del radical y el resultado se multiplica por el radicando del radical. De esta manera, y teniendo en cuenta las propiedades de las operaciones con potencias, para introducir una potencia dentro de un radical multiplicaremos el exponente de la potencia por el índice del radical. La potencia resultante pasará dentro del radical multiplicando al radicando.
Introduce los factores dentro del radical: ![m^2 n^5 \sqrt[5]{m^3 p^2}](/wikipedia/images/math/2/7/6/276aa6fd087cafe6e44377e54dfae158.png)
Para introducir un factor dentro de un radical, éste se eleva al índice del radical y el resultado se multiplica por el radicando del radical. De esta manera, y teniendo en cuenta las propiedades de las operaciones con potencias, para introducir una potencia dentro de un radical multiplicaremos el exponente de la potencia por el índice del radical. La potencia resultante pasará dentro del radical multiplicando al radicando. Si dentro del radical tenemos otra potencia con la misma base entonces sumaremos el exponente de la potencia que entra con el de dentro del radical.
Introduce factores dentro del radical:
- a)
![4 \sqrt[3]{6}](/wikipedia/images/math/0/9/8/09832a2fdbea98af094bc3b99a2f71fb.png)
- b)
![2^4 \cdot 3^2 \sqrt[6]{2^2 \cdot 3^5}](/wikipedia/images/math/a/3/e/a3e7fc4c36dc72ff46d3e14c87570f8c.png)
- b)
![a^7 \cdot b^8 \sqrt[5]{b^2}](/wikipedia/images/math/7/1/c/71c6e44e4a1a1f816fefc0e45b931acc.png)
Pulsa el botón EJERCICIO y verás el enunciado; hazlo en tu cuaderno e introduce la solución con la escena, luego pulsa el botón SOLUCIÓN para ver si lo has hecho bien.
Ejercicios de autoevaluación sobre introducción y extracción de factores de un radical.
Suma y resta de radicales con el mismo índice y distinto radicando
Si tienen el mismo índice pero distinto radicando, a veces, podemos extraer factores del radical y dejarlos con el mismo radicando.
Ejemplo: Suma y resta de radicales con el mismo índice y distinto radicando
Resta los siguientes radicales: 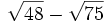
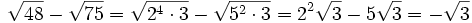
Pulsa el botón "Ejemplo" para ver los ejemplos. Anota algunos en tu cuaderno.
Tutorial que explica cómo sumar y restar radicales. La suma y resta son operaciones que "se llevan muy mal" con el resto de operaciones y hay que tener mucho cuidado a la hora de hacerlo con radicales.
Suma y resta de radicales con el mismo índice. Ejemplos.
Simplifica: 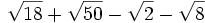
Simplifica: 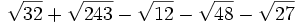
Calcula:
- a)
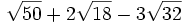
- b)
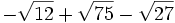
- c)

- d)
![\sqrt[3]{48}+\sqrt[3]{1080}-\sqrt[3]{96}\;](/wikipedia/images/math/2/d/6/2d67b353649768215c317a719b4f248d.png)
- e)
![-\sqrt[3]{-27}+2\sqrt[3]{272}-9\sqrt[3]{-81}\;](/wikipedia/images/math/c/0/f/c0ff906569b1db6871629962fc420418.png)
Calcula:
- a)
![\sqrt[4]{32}+8\sqrt[4]{16}-\sqrt[4]{80}\;](/wikipedia/images/math/4/c/a/4cac9ae91631e9811be06e223293e340.png)
- b)
![\sqrt[5]{64}-6\sqrt[5]{32}+5\sqrt[5]{96}\;](/wikipedia/images/math/7/a/f/7af16a27ecbd28162b9ffd8bcc06a665.png)
- c)
![-9\sqrt[6]{64}+\sqrt[6]{192}-\sqrt[6]{960}\;](/wikipedia/images/math/4/5/c/45c6fc0df3cf3b4245b56dcf055735ec.png)
Pulsa el botón EJERCICIO y verás el enunciado; hazlo en tu cuaderno e introduce la solución con la escena, luego pulsa el botón SOLUCIÓN para ver si lo has hecho bien.
Producto y cocientes de radicales con distinto índice
Para multiplicar o dividir radicales con distinto índice, primero se reducen a índice común y luego se multiplican o dividen los radicandos.
Ejemplo: Producto y cocientes de radicales con distinto índice
Reduce a un solo radical ![\sqrt[3]{10} \cdot \sqrt[4]{5}:\sqrt{8}](/wikipedia/images/math/5/9/7/597ebbc8a8a1e63faeabbecf3da740ef.png)
Para reducir los radicales a índice común calculamos el m.c.m de los índices: m.c.m.(3,4,2)=12 y elevamos cada radicando al resultado de dividir el m.c.m. por el índice de cada radical.
![\sqrt[3]{10} \cdot \sqrt[4]{5}:\sqrt{8}=\sqrt[12]{10^4} \cdot \sqrt[12]{5^3}:\sqrt[12]{8^6}](/wikipedia/images/math/e/8/1/e81ffde8d1c752d66e97b66e25149a0f.png)
Luego multiplicamos o dividimos los radicandos, ya que ahora los índices son iguales:
![\sqrt[12]{10^4} \cdot \sqrt[12]{5^3}:\sqrt[12]{8^6}=\sqrt[12]{10^4 \cdot 5^3 : 8^6}](/wikipedia/images/math/6/d/1/6d1f82fd6b85971e0e493c1e7eb2d485.png)
Finalmente simplificamos:
![\sqrt[12]{10^4 \cdot 5^3 : 8^6}=\sqrt[12]{2^4 \cdot 5^4 \cdot 5^3 : (2^3)^6}=\sqrt[12]{2^{-14} \cdot 5^7}](/wikipedia/images/math/7/0/e/70e1e7afd0d65ac459a2b76336b7fbb6.png)
Producto y cociente de radicales con el mismo o con distinto índice. Ejemplos.
Simplifica: ![(8\sqrt[3]{a^2b}) \cdot (4\sqrt{ab^3})](/wikipedia/images/math/8/e/a/8ea372bbacf499b888b5f31aeb1cae8b.png)
Simplifica: ![\sqrt[3]{27^{-2}} : \sqrt[4]{16}](/wikipedia/images/math/1/0/d/10dcb2912a1fddcdb4f0013df23cedc3.png)
Actividades en las que podrás aprender a multiplicar y dividir radicales de distinto índice previa reducción a índice común.
Ejercicios de autoevaluación sobre productos de radicales.
Ejercicios de autoevaluación sobre cocientes de radicales.
Potencias de radicales
Potencias de radicales. Ejemplos.
Ejercicios de autoevaluación sobre potencias de radicales.
Radicales dobles (Avanzado)
Convierte los siguientes radicales dobles en sencillos:
a) 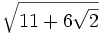
b) 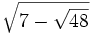
Convierte los siguientes radicales dobles en sencillos:
a) 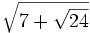
b) 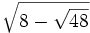
Convierte los siguientes radicales sencillos en dobles:
a) 
b) 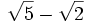
Actividades
Simplifica:
a)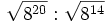
b)![\sqrt[6]{\sqrt{8}}](/wikipedia/images/math/b/d/b/bdbeee310b4a724e942f08bffbfa8d23.png)
c)![\sqrt[9]{12} \cdot \sqrt{3}](/wikipedia/images/math/7/2/8/72833303cca23937986fcffc7c483ab7.png)
Simplifica: 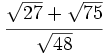
Simplifica: ![\sqrt [4]{\sqrt{x^3} \cdot 16 \sqrt{x}}](/wikipedia/images/math/c/b/1/cb16004d071779fc6b1ffc6f93945a68.png)
Simplifica: ![\left(\cfrac{\sqrt [6]{32}}{\sqrt{8}} \right)^3](/wikipedia/images/math/e/a/c/eacfafeb055cc8c9cdb0aad1d2009d5a.png)
Simplifica (Extracción e introducción de factores en un radical):
a) ![\sqrt[3]{5^{17} \cdot 4^6}](/wikipedia/images/math/0/0/5/005d7f0a77ef68096dbc57b6faf62174.png)
b) ![\sqrt[3]{16\, a^4\, b^{21}}](/wikipedia/images/math/1/0/a/10a9ce8e21e1692804f04887051c25a2.png)
c) ![7^3 \cdot 6^9 \sqrt[11]{y^3}](/wikipedia/images/math/6/d/d/6dd037766dcba2853e802af0eb172662.png)
Simplifica:
a) 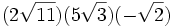
b) ![(5\sqrt[3]{4})(2\sqrt[3]{2})](/wikipedia/images/math/c/4/a/c4a86a9d45d3ab1a13edab27c030aed9.png)
c) ![(7\sqrt{2})(5\sqrt[3]{3})](/wikipedia/images/math/9/8/1/981355fbebaebcc7ed55c7fad7b969d5.png)
Simplifica:
a) 
b) ![\cfrac{24\sqrt[4]{2}}{4\sqrt[5]{3}}](/wikipedia/images/math/b/3/c/b3c493d2101ba7fdd69acb88e870ea21.png)
Simplifica:
a) 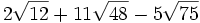
b) 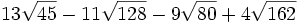
Simplifica:
a) ![3\sqrt[5]{2}+7\sqrt[5]{2}](/wikipedia/images/math/d/2/7/d27d96074bab9896a713780d59467405.png)
b) 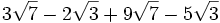
c) ![3\sqrt[3]{54}-2\sqrt[3]{2}+9\sqrt[3]{16}](/wikipedia/images/math/1/d/0/1d09e34f1f3456a7a3bee748760e4d4f.png)
d) ![4\sqrt[4]{25}-\sqrt{45}](/wikipedia/images/math/d/d/b/ddb97312176c5bcecf2ee242e8ad1131.png)
e) ![\cfrac{1}{2}\sqrt[3]{108}-\cfrac{3}{2}\sqrt[3]{4}](/wikipedia/images/math/b/d/5/bd562c177c7713390d93927f9b4bc7d4.png)
Calcula y simplifica:
- a)
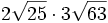 ; b)
; b) 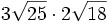 ; c)
; c) 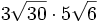
- d)
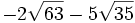 ; e)
; e) 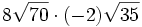 ; f)
; f) 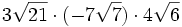
- g)
 ; f)
; f) 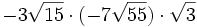
Opera y simplifica:
- a)
![2\sqrt[3]{18} \cdot 3\sqrt[3]{12}\;](/wikipedia/images/math/0/3/1/031733f0fbd54f968faa9fe5eda8d0a5.png) ; b)
; b) ![3\sqrt[3]{50} \cdot 2\sqrt[3]{20}\;](/wikipedia/images/math/5/3/2/5325b2d9d555d9f858cb45e672a5d4f1.png) ; c)
; c) ![3\sqrt[3]{45} \cdot 5\sqrt[3]{75}\;](/wikipedia/images/math/d/0/3/d03eb8f8d36666972cc949f4aa84135f.png)
- d)
![-2\sqrt[3]{28} \cdot 5\sqrt[3]{98}\;](/wikipedia/images/math/a/e/0/ae069089d23479aab015b6e60f86d52f.png) ; e)
; e) ![8\sqrt[3]{-12} \cdot (-3\sqrt[3]{3}) \cdot \sqrt[3]{-6}\;](/wikipedia/images/math/4/c/2/4c2775ca1af101fe6d39c7594ff03cf3.png) ; f)
; f) ![3\sqrt[3]{8} \cdot (-7\sqrt[3]{9}) \cdot 4\sqrt[3]{3}\;](/wikipedia/images/math/7/6/2/762033e0f8daa6c889ef1b8ff3aedd7a.png)
- g)
![-5\sqrt[3]{27} \cdot (-\sqrt[3]{4}) \cdot 4\sqrt[3]{2}\;](/wikipedia/images/math/c/7/a/c7a78297a356e0f2492f0896287bf16f.png) ; h)
; h) ![3\sqrt[3]{20} \cdot (-3\sqrt[3]{10}) \cdot (-4\sqrt[3]{5})\;](/wikipedia/images/math/6/6/6/666f233d469263babf8b6568f8816f5f.png) ; i)
; i) ![3\sqrt[6]{324} \cdot 5\sqrt[6]{144}\;](/wikipedia/images/math/d/3/e/d3ec755367d0b6a0b64cb68db1a7cd6d.png)
- j)
![-2\sqrt[4]{250} \cdot 5\sqrt[4]{40}\;](/wikipedia/images/math/4/5/9/45941ba5c1a9b2af7e5f5acfe1ab8614.png) ; k)
; k) ![3\sqrt[4]{88} \cdot (-7\sqrt[8]{216}) \cdot 4\sqrt[8]{162}\;](/wikipedia/images/math/4/8/6/48604a9cfa646b3261cb09804c4afc34.png)
Opera y simplifica:
- a)
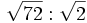 ; b)
; b) 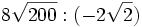
Opera y simplifica:
- a)
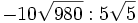 ; b)
; b) 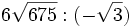 c)
c) 
- d)
![9\sqrt[3]{432} : (-\sqrt[3]{-2})\;](/wikipedia/images/math/f/b/9/fb95dd8cbe8cfd4e0c49eec43dda6ff7.png) ; e)
; e) ![-10\sqrt[3]{1458} : 5\sqrt[3]{2}\;](/wikipedia/images/math/e/e/f/eef5a8ba7329df0a841ecd829db26562.png) ; f)
; f) ![12\sqrt[3]{192} : (-6\sqrt[3]{-3})\;](/wikipedia/images/math/8/0/7/807881bc4d10def8325191dac48379b0.png)
- g)
![-30\sqrt[3]{3000} : 6\sqrt[3]{3}\;](/wikipedia/images/math/f/f/e/ffec5e6b75ac1f80c24af9caa431a158.png) ; h)
; h) ![\sqrt[4]{512} : \sqrt[4]{2}\;](/wikipedia/images/math/0/b/5/0b5ce95019fc14a1775557e6ba43ac1b.png) ; i)
; i) ![9\sqrt[4]{768} : (-\sqrt[4]{3})\;](/wikipedia/images/math/0/6/5/06566e947dc6e1be1bb70ce72612c1b0.png)
- j)
![-10\sqrt[4]{2592} : 5\sqrt[4]{2}\;](/wikipedia/images/math/5/9/c/59c73064c6c6c8edd9a75643e619e889.png) ; k)
; k) ![12\sqrt[4]{3888} : (-6\sqrt[4]{3})\;](/wikipedia/images/math/e/6/f/e6f826708d9b60ae3eec0ce03419beaa.png) ; l)
; l) ![-30\sqrt[4]{13122} : 6\sqrt[4]{2}\;](/wikipedia/images/math/f/3/8/f38325af6ab9a4b792be123712a935eb.png)
Calcula:
- a)
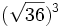 ; b)
; b) 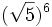 ; c)
; c) 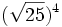 ; d)
; d) 
Calcula:
- a)
![(\sqrt[3]{-100})^4\;](/wikipedia/images/math/f/9/8/f9898c4fea7d81c6a960d595902aed45.png) ; b)
; b) ![(\sqrt[3]{18})^4\;](/wikipedia/images/math/b/8/2/b821a9571353a23729e0d70db373b576.png) ; c)
; c) ![(\sqrt[4]{7})^5\;](/wikipedia/images/math/7/e/5/7e5c3062446a9e6e968bcc8e047c05f2.png) ; d)
; d) ![(\sqrt[4]{21})^7\;](/wikipedia/images/math/d/2/2/d2219bd465cdf2cd6bd4ee043a87fa68.png)
- e)
![(\sqrt[4]{100})^5\;](/wikipedia/images/math/d/e/e/dee501759eec044d5e4b65b67661a26a.png) ; f)
; f) ![(\sqrt[5]{16})^7\;](/wikipedia/images/math/c/4/7/c477f227f0e345150bd5d4465006a35c.png) ; g)
; g) ![(\sqrt[6]{12})^7\;](/wikipedia/images/math/a/f/f/aff12187841d83e96f42fc74b7813d9e.png)
Calcula y simplifica:
- a)
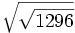 ; b)
; b) 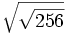 ; c)
; c) ![\sqrt[3]{\sqrt{46\,656}}\;](/wikipedia/images/math/4/4/b/44b6d41e62a3bb8b4f098b0be493b394.png)
- d)
![\sqrt[3]{\sqrt[3]{4096}}\;](/wikipedia/images/math/b/a/5/ba5c5b911a2c67423eff1042451512d8.png) ; e)
; e) ![\sqrt{\sqrt[3]{1024}}\;](/wikipedia/images/math/0/d/f/0df78f26900c5ba4320ea30e1acdadc1.png) ; f)
; f) ![\sqrt[4]{\sqrt[3]{4096}}\;](/wikipedia/images/math/4/3/8/4388e0aec792ebc9974cea4ab170a49d.png)
En esta escena podrás practicar la suma y resta de radicales con o sin el mismo índice.
Ejercicios de autoevaluación sobre sumas y restas de radicales.
Ejercicios de autoevaluación sobre raíces de radicales.
![\sqrt[n]{a^m}= a^{\frac{m}{n}} \begin{matrix} ~_{(1)}~ \\ = \\ \, \end{matrix} a^{c+\frac{r}{n}}= a^c \cdot a^{\frac{r}{n}}= a^c \cdot \sqrt[n]{a^r}](/wikipedia/images/math/d/3/9/d39083130ff7fc81f2df907b77c139e4.png)
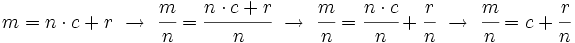
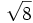 b)
b) 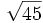
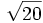 b)
b) 
![\sqrt[3]{16}\;](/wikipedia/images/math/5/3/a/53a3c4a84f10981709eb9cbe55fd96d6.png) b)
b) ![\sqrt[3]{72}\;](/wikipedia/images/math/7/3/2/73202ad33b01491941443d47216fb27f.png)
![\sqrt[5]{96}\;](/wikipedia/images/math/9/d/c/9dcae009beea43dfbe3404b5f004cc4a.png) b)
b) ![\sqrt[4]{405}\;](/wikipedia/images/math/a/8/9/a890f47fe2c93a2f06c95c8202cb8251.png)
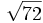 b)
b) ![\sqrt[4]{3645}\;](/wikipedia/images/math/6/4/e/64e81b0470d1d706dc6259ee3c15b821.png) c)
c)  c)
c) ![5\,\sqrt[3]{384}\;](/wikipedia/images/math/4/9/0/4905ec017f8b5e0d98f7ee1f7eec1207.png)
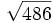 b)
b) ![6\sqrt[3]{1250}\;](/wikipedia/images/math/4/3/4/4346e96a7c5105fca5f88837843083a9.png) c)
c) ![m^3n\sqrt[5]{m^{20}n^{37}}\;](/wikipedia/images/math/4/9/6/496807e951625205ca38c068a23ebc66.png)
![-2\sqrt [3]{16x^5yz^9}](/wikipedia/images/math/5/d/a/5da6c4122805a4279103f0cf5cfb13c0.png)
![\sqrt [4]{32x^4y^{21}z^{43}}](/wikipedia/images/math/c/4/f/c4f4294a30ee9a53a181d8f3abd8d54b.png)
![\sqrt [5]{32x^5y^{-10}z^{-35}}](/wikipedia/images/math/e/f/b/efb9fab775fddc918c97f70c90e23eb4.png)
![\sqrt [5]{-243a^{20}}](/wikipedia/images/math/e/e/3/ee38623c8fe3fac34be9faf93f9e8457.png)
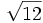 ; b)
; b) 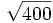 ;
;
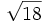 ; e)
; e) 
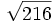 ; g)
; g) 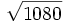 ; h)
; h) ![\sqrt[3]{625}\;](/wikipedia/images/math/e/5/d/e5d68b00dfbd79e2f3d58e0aca3d7c0f.png)
![\sqrt[3]{432}\;](/wikipedia/images/math/6/d/1/6d13f38f4d1eb2019980f043401b4212.png) ; j)
; j) ![\sqrt[3]{-216}\;](/wikipedia/images/math/f/2/e/f2e9196b5535a696cacaea492b5fca1f.png) ; k)
; k) ![\sqrt[3]{243}\;](/wikipedia/images/math/8/f/5/8f5ffd08c6adee125672bdaceac21095.png)
![\sqrt[4]{1296}\;](/wikipedia/images/math/d/f/8/df8a4653fd51d5f3a467722317d864f7.png) ; m)
; m) ![\sqrt[4]{20736}\;](/wikipedia/images/math/e/0/3/e0364ebd0e97bb3e82e855e75e9794bd.png) ; n)
; n) ![\sqrt[5]{-480}\;](/wikipedia/images/math/f/5/e/f5e99ceeb09f023ea88f8aae9d707acc.png)
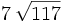
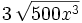

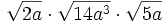
![5\,\sqrt[3]{2x^2} \cdot 3\,\sqrt[3]{4x^4}\;](/wikipedia/images/math/b/4/5/b4564f0c1cace422e46c7b4e8bef605e.png)
![\sqrt[3]{125x^6y^3}](/wikipedia/images/math/6/4/b/64b184793ed736d13012a61c0bb6a166.png)
![\sqrt[4]{5a^4b^{12}}](/wikipedia/images/math/a/b/a/abab543450564364e8ced43319182eb4.png)
![a \sqrt[n]{b}=a \cdot b^{\frac{1}{n}}=(a^n)^{\frac{1}{n}} \cdot b^{\frac{1}{n}}= (a^n \cdot b)^{\frac{1}{n}}= \sqrt[n]{a^n \cdot b}](/wikipedia/images/math/7/9/3/793321a371ba1c1c188fd5e877399640.png)
![\sqrt[4]{3} - \sqrt[4]{243}](/wikipedia/images/math/e/6/6/e66fc6d8653c96dff0e3c0b95dc93b55.png)

