Operaciones con potencias (1ºESO)
De Wikipedia
| Revisión de 10:18 11 sep 2016 Coordinador (Discusión | contribuciones) (→Propiedades de las potencias) ← Ir a diferencia anterior |
Revisión actual Coordinador (Discusión | contribuciones) (→Ejercicios propuestos) |
||
| Línea 7: | Línea 7: | ||
| {{p}} | {{p}} | ||
| (Pág. 31) | (Pág. 31) | ||
| - | ==Propiedades de las potencias== | + | ==Operaciones con potencias== |
| - | {{propiedades potencias naturales}} | + | {{Video_enlace_sensei |
| - | {{p}} | + | |titulo1=Operaciones con potencias de números naturales |
| - | {{Ejemplo_simple|titulo=Ejemplos:|contenido= | + | |duracion=12'08" |
| - | *'''Producto de potencias de la misma base:''' | + | |sinopsis=En este videotutorial haremos una introducción a las operaciones con potencias más usuales. |
| - | + | |url1=http://www.youtube.com/watch?v=7VN_1Ga66jc&index=7&list=PLA0brQx7U3OVuqlFgdMawKu5TtMwqCbH5 | |
| - | {{p}} | + | |
| - | <center><math>\begin{matrix} 5^4 \cdot 5^3 \, \\ \; \end{matrix} | + | |
| - | \begin{matrix} \ = \ \\ \; \end{matrix} | + | |
| - | \begin{matrix} \underbrace{ (5 \cdot 5 \cdot 5 \cdot 5) } \\ 4 \, \mbox{veces} \end{matrix} | + | |
| - | \begin{matrix} \ \cdot \ \\ \; \end{matrix} | + | |
| - | \begin{matrix} \underbrace{ (5 \cdot 5 \cdot 5) } \\ 3 \, \mbox{veces} \end{matrix} | + | |
| - | \begin{matrix} \ = \ \\ \; \end{matrix} | + | |
| - | \begin{matrix} 5^{4+3} = 5^7 \\ \; \end{matrix} | + | |
| - | </math> | + | |
| - | + | ||
| - | </center> | + | |
| - | <br> | + | |
| - | *'''Potencia de un producto:''' | + | |
| - | <center><math>(2 \cdot 3)^3 = 6^3 = 6 \cdot 6 \cdot 6 = 216</math></center> | + | |
| - | {{p}} | + | |
| - | <center><math>2^3 \cdot 3^3 = (2 \cdot 2 \cdot 2) \cdot (3 \cdot 3 \cdot 3) = 8 \cdot 27 = 216</math></center> | + | |
| - | {{p}} | + | |
| - | <center>Las dos formas de hacerlo son equivalentes.</center> | + | |
| - | <br> | + | |
| - | *'''Potencia de un cociente:''' | + | |
| - | <center><math>(6 : 3)^3 = 2^3 = 2 \cdot 2 \cdot 2 = 8</math></center> | + | |
| - | {{p}} | + | |
| - | <center><math>6^3 : 2^3 = 16 : 27 = 8\;</math></center> | + | |
| - | {{p}} | + | |
| - | <center>Las dos formas de hacerlo son equivalentes.</center> | + | |
| - | <br> | + | |
| - | *'''Potencia de otra potencia:''' | + | |
| - | {{p}} | + | |
| - | <center><math>(5^4)^3 = 5^4 \cdot 5^4 \cdot 5^4 = 5^{4+4+4} = 5^{4 \cdot 3} = 5^{12}</math></center> | + | |
| - | <br> | + | |
| - | *'''Potencia cero:''' Cuando se vio la definición de potencia, dijimos que {{sube|porcentaje=15%|contenido=<math>a^0 = 1\;</math>}} por convenio. Expliquemos ésto ahora un poco mejor: | + | |
| - | <center> | + | |
| - | <math>\left.\begin{matrix} | + | |
| - | 5^3:5^3 =5^{3-3}=5^0 | + | |
| - | \\ | + | |
| - | 5^3:5^3=125:125=1 | + | |
| - | + | ||
| - | \end{matrix}\right\} \ \rightarrow \ 5^0 = 1</math> | + | |
| - | </center> | + | |
| - | + | ||
| }} | }} | ||
| - | {{p}} | ||
| - | {{AI_enlace | ||
| - | |titulo1=Producto de potencias de la misma base | ||
| - | |descripcion= | ||
| - | Para ver paso a paso las transformaciones debes pulsar sobre el triángulo azul de arriba de la escena. | ||
| - | {{p}} | ||
| - | El producto de varias potencias de la misma base equivale a otra potencia cuya base es la misma y cuyo exponente es la suma de los exponentes. | ||
| - | <center><iframe> | + | ==Propiedades de las operaciones con potencias de números naturales== |
| - | url=http://maralboran.org/web_ma/descartes/1y2_eso/potencia/producto_1.html | + | {{propiedades potencias naturales}} |
| - | width=550 | + | |
| - | height=350 | + | |
| - | name=myframe | + | |
| - | </iframe></center> | + | |
| - | + | ||
| - | |url1=http://maralboran.org/web_ma/descartes/1y2_eso/potencia/producto_1.html | + | |
| - | }} | + | |
| {{p}} | {{p}} | ||
| - | {{AI_enlace | + | {{Ejemplo: propiedades potencias naturales}} |
| - | |titulo1=Cociente de potencias de la misma base | + | |
| - | |descripcion= | + | |
| - | Para ver paso a paso las transformaciones debes pulsar sobre el triángulo azul de arriba de la escena. | + | |
| - | {{p}} | + | |
| - | El cociente de dos potencias de la misma base equivale a otra potencia cuya base es la misma y cuyo exponente es la resta de los exponentes. | + | |
| - | + | ||
| - | <center><iframe> | + | |
| - | url=http://maralboran.org/web_ma/descartes/1y2_eso/potencia/cociente_1.html | + | |
| - | width=550 | + | |
| - | height=350 | + | |
| - | name=myframe | + | |
| - | </iframe></center> | + | |
| - | + | ||
| - | |url1=http://maralboran.org/web_ma/descartes/1y2_eso/potencia/cociente_1.html | + | |
| - | }} | + | |
| {{p}} | {{p}} | ||
| - | {{AI_enlace | + | {{Videos: propiedades potencias naturales}} |
| - | |titulo1=Potencia de una potencia | + | |
| - | |descripcion= | + | |
| - | Para ver paso a paso las transformaciones debes pulsar sobre el triángulo azul de arriba de la escena. | + | |
| {{p}} | {{p}} | ||
| - | La potencia de una potencia equivale a una potencia simple cuya base es la misma y cuyo exponente es el producto de los exponentes. | + | {{Actividades: Propiedades potencias con naturales}} |
| - | <center><iframe> | + | ==Actividades== |
| - | url=http://maralboran.org/web_ma/descartes/1y2_eso/potencia/potpot_1.html | + | {{actividades potencias naturales}} |
| - | width=550 | + | |
| - | height=350 | + | |
| - | name=myframe | + | |
| - | </iframe></center> | + | |
| - | + | ||
| - | |url1=http://maralboran.org/web_ma/descartes/1y2_eso/potencia/potpot_1.html | + | |
| - | }} | + | |
| - | {{p}} | + | |
| - | {{AI_enlace | + | |
| - | |titulo1=Potencia de un producto | + | |
| - | |descripcion= | + | |
| - | Para ver paso a paso las transformaciones debes pulsar sobre el triángulo azul de arriba de la escena. | + | |
| - | {{p}} | + | |
| - | La potencia de un producto equivale al producto de potencias cuyas bases son cada uno de los factores y cuyo exponente es el mismo. | + | |
| - | + | ||
| - | <center><iframe> | + | |
| - | url=http://maralboran.org/web_ma/descartes/1y2_eso/potencia/potprod_1.html | + | |
| - | width=550 | + | |
| - | height=400 | + | |
| - | name=myframe | + | |
| - | </iframe></center> | + | |
| - | + | ||
| - | |url1=http://maralboran.org/web_ma/descartes/1y2_eso/potencia/potprod_1.html | + | |
| - | }} | + | |
| {{p}} | {{p}} | ||
| (Pág. 31-32) | (Pág. 31-32) | ||
| Línea 138: | Línea 42: | ||
| :a) Aplicando la propiedad 3: | :a) Aplicando la propiedad 3: | ||
| - | <center><math>5^6 \cdot 2^6 = (5 \cdot 2)^6 = 10^6 = 1,000,000</math></center> | + | <center><math>5^6 \cdot 2^6 = (5 \cdot 2)^6 = 10^6 = 1\,000\,000</math></center> |
| :b) Aplicando la propiedad 4: | :b) Aplicando la propiedad 4: | ||
| Línea 146: | Línea 50: | ||
| :c) Aplicando la propiedad 3: | :c) Aplicando la propiedad 3: | ||
| - | <center><math>(6^4 \cdot 5^4):15^4 = (6 \cdot 5)^4 : 15^4 = 30^4 : 15^4</math></center> | + | <center><math>(6^4 \cdot 5^4):15^4 = (6 \cdot 5)^4 : 15^4 = 30^4 : 15^4=</math></center> |
| :{{b4}}y aplicando la propiedad 4: | :{{b4}}y aplicando la propiedad 4: | ||
| - | <center><math>30^4 : 15^4 = (30 : 15)^4 = 2^4 =16\;</math></center> | + | <center><math>= (30 : 15)^4 = 2^4 =16\;</math></center> |
| {{p}} | {{p}} | ||
| :d) Aplicando las propiedades 5 y 1: | :d) Aplicando las propiedades 5 y 1: | ||
| - | <center><math>(8^3)^2 :(8^3 \cdot 8^2) = 8^{3 \cdot 2} : 8^{3+2} = 8^6 : 8^5</math></center> | + | <center><math>(8^3)^2 :(8^3 \cdot 8^2) = 8^{3 \cdot 2} : 8^{3+2} = 8^6 : 8^5=</math></center> |
| :{{b4}} y aplicando la propiedad 2: | :{{b4}} y aplicando la propiedad 2: | ||
| - | <center><math>8^6 : 8^5 = 8^{6-5} = 8^1 = 8\;</math></center> | + | <center><math> = 8^{6-5} = 8^1 = 8\;</math></center> |
| :e) Por las propiedades 1 y 2: | :e) Por las propiedades 1 y 2: | ||
| - | <center><math>(a^2 \cdot a)^4 :(a^6:a^3)^3= (a^{2+1})^4 : (a^{6-3})^3 =(a^3)^4 : (a^3)^3</math></center> | + | <center><math>(a^2 \cdot a)^4 :(a^6:a^3)^3= (a^{2+1})^4 : (a^{6-3})^3 =(a^3)^4 : (a^3)^3=</math></center> |
| :{{b4}} y por las propiedades 5 y 2: | :{{b4}} y por las propiedades 5 y 2: | ||
| - | <center><math>(a^3)^4 : (a^3)^3 = a^1 : a^9 = a^{12-9} = a^3\;</math></center> | + | <center><math>= a^{12} : a^9 = a^{12-9} = a^3\;</math></center> |
| }} | }} | ||
| Línea 175: | Línea 79: | ||
| {{b4}}(Pág. 33) | {{b4}}(Pág. 33) | ||
| - | {{b4}}[[Imagen:red_star.png|12px]] 3; 6a,c,e; 7a,d; 8a,d; 9; 10 | + | {{b4}}[[Imagen:red_star.png|12px]] 2a,b,c,g,h,i; 3a,c,e; 4a; 5; 6a,c,e; 7a,b,d; 8a,b,d; 9a,c,f,g,j; 10 |
| - | + | ||
| - | {{b4}}[[Imagen:yellow_star.png|12px]] 1; 2; 4; 5; 6b,d,f; 7b,c,e,f; 8b,c,e,f | + | |
| + | {{b4}}[[Imagen:yellow_star.png|12px]] el resto | ||
| |sol= | |sol= | ||
| }} | }} | ||
| [[Categoría: Matemáticas]][[Categoría: Números]] | [[Categoría: Matemáticas]][[Categoría: Números]] | ||
Revisión actual
| Enlaces internos | Para repasar | Para ampliar | Enlaces externos |
| Indice Descartes Manual Casio | WIRIS Geogebra Calculadora |
Tabla de contenidos |
(Pág. 31)
Operaciones con potencias
En este videotutorial haremos una introducción a las operaciones con potencias más usuales.
Propiedades de las operaciones con potencias de números naturales
Propiedades de las potencias
- 1. Producto de potencias de la misma base:
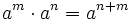
- 2. Cociente de potencias de la misma base:

- 3. Potencia de un producto:
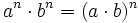
- 4. Potencia de un cociente:
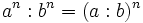
- 5. Potencia de otra potencia:
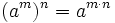
- Producto de potencias de la misma base:
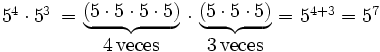
- Cociente de potencias de la misma base:
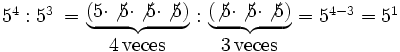
- Potencia de un producto:
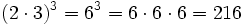

- Potencia de un cociente:
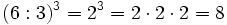
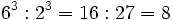
- Potencia de otra potencia:
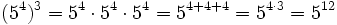
- Potencia cero: Cuando se vio la definición de potencia, dijimos que
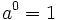 por convenio. Expliquemos ésto ahora un poco mejor:
por convenio. Expliquemos ésto ahora un poco mejor:
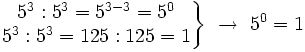
Potencias de números naturales con exponente natural.
- Producto de potencias de la misma base.
- Cociente de potencias de la misma base.
- Potencia de otra potencia.
- Ejemplos.
Aprende a multiplicar potencias con la misma base.
Aprende a dividir potencias con la misma base.
Aprende a calcular la potencia de otra potencia.
Aprende a multiplicar potencias con la misma base.
Aprende a dividir potencias con la misma base.
Aprende a calcular la potencia de un producto.
Aprende a calcular la potencia de un cociente.
Aprende a calcular la potencia de una potencia.
Enunciados de las propiedades de las potencias de números naturales.
Ejemplos de aplicación de las propiedades de las potencias de números naturales.
Ejercicio de aplicación de las propiedades de las potencias de números naturales.
Ejercicio de aplicación de las propiedades de las potencias de números naturales.
Ejercicio de aplicación de las propiedades de las potencias de números naturales.
Ejercicio de aplicación de las propiedades de las potencias de números naturales.
Ejercicio de aplicación de las propiedades de las potencias de números naturales.
Practica con las propiedades de las operaciones con potencias de números naturales.
Practica con las propiedades de las operaciones con potencias de números naturales.
Para ver paso a paso las transformaciones debes pulsar sobre el triángulo azul de arriba de la escena.
El producto de varias potencias de la misma base equivale a otra potencia cuya base es la misma y cuyo exponente es la suma de los exponentes.
Para ver paso a paso las transformaciones debes pulsar sobre el triángulo azul de arriba de la escena.
El cociente de dos potencias de la misma base equivale a otra potencia cuya base es la misma y cuyo exponente es la resta de los exponentes.
Para ver paso a paso las transformaciones debes pulsar sobre el triángulo azul de arriba de la escena.
La potencia de una potencia equivale a una potencia simple cuya base es la misma y cuyo exponente es el producto de los exponentes.
Para ver paso a paso las transformaciones debes pulsar sobre el triángulo azul de arriba de la escena.
La potencia de un producto equivale al producto de potencias cuyas bases son cada uno de los factores y cuyo exponente es el mismo.
Producto de potencias de la misma base.
Potencia de otra potencia.
Cociente de potencias de la misma base.
Potencia de un producto y de un cociente (práctica estructurada).
Potencia de un producto y de un cociente.
Rellena todas las cajas inferiores y pulsa "intro" al final. Cuando hayas marcado correctamente los tres aparecerá el mensaje CORRECTO, pero si marcas antes un número equivocado ya no aparecerá ese mensaje, por eso, no emplees los triángulos arriba y abajo para variar el número.
Ejercicios de autoevaluación sobre operaciones con potencias.
Ejercicios de autoevaluación sobre operaciones con potencias en los que puedes elegir el nivel y el tipo de operaciones.
Actividades
(Pág. 31-32)
Ejercicios resueltos: Operaciones con potencias
- a) Calcula por el camino más sencillo:
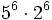
- b) Calcula por el camino más sencillo:
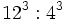
- c) Calcula con la ayuda de las propiedades:
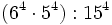
- d) Calcula con la ayuda de las propiedades:

- e) Reduce a una sola potencia:
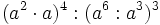
- a) Aplicando la propiedad 3:
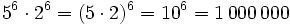
- b) Aplicando la propiedad 4:
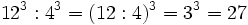
- c) Aplicando la propiedad 3:
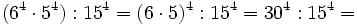
- y aplicando la propiedad 4:
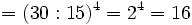
- d) Aplicando las propiedades 5 y 1:
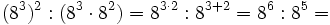
- y aplicando la propiedad 2:

- e) Por las propiedades 1 y 2:
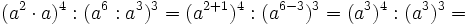
- y por las propiedades 5 y 2:
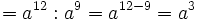
Ejercicios propuestos
|
Ejercicios propuestos: Operaciones con potencias |

