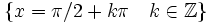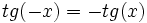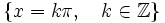Funciones trigonométricas o circulares (1ºBach)
De Wikipedia
(Diferencia entre revisiones)
| Revisión de 09:52 3 mar 2009 Coordinador (Discusión | contribuciones) ← Ir a diferencia anterior |
Revisión de 10:19 3 mar 2009 Coordinador (Discusión | contribuciones) Ir a siguiente diferencia → |
||
| Línea 26: | Línea 26: | ||
| *'''Mínimos:''' <math>\left \{ x=3 \pi /2 +2 \pi k \, , \quad k \in \mathbb{Z} \right \}</math> | *'''Mínimos:''' <math>\left \{ x=3 \pi /2 +2 \pi k \, , \quad k \in \mathbb{Z} \right \}</math> | ||
| *'''Crecimiento:''' | *'''Crecimiento:''' | ||
| - | **Crece en <math>\big( 3 \pi / 2+2 \pi k , \, \pi /2 +2 \pi k \big), \quad k \in \mathbb{Z}</math>. | + | **Crece en <math>\big( 3 \pi / 2+2 \pi (k-1) , \, \pi /2 +2 \pi k \big), \quad k \in \mathbb{Z}</math>. |
| **Decrece en <math>\big( \pi / 2+2 \pi k , \, 3 \pi /2 +2 \pi k \big), \quad k \in \mathbb{Z}</math>. | **Decrece en <math>\big( \pi / 2+2 \pi k , \, 3 \pi /2 +2 \pi k \big), \quad k \in \mathbb{Z}</math>. | ||
| }} | }} | ||
| Línea 38: | Línea 38: | ||
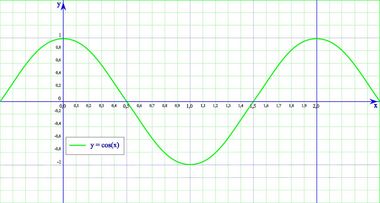
| <center><math>f(x)=cos(x) \, , \quad x \in \mathbb{R}</math></center> | <center><math>f(x)=cos(x) \, , \quad x \in \mathbb{R}</math></center> | ||
| }} | }} | ||
| - | ===Propiedades de la función seno=== | + | ===Propiedades de la función coseno=== |
| {{Caja_Amarilla|texto= | {{Caja_Amarilla|texto= | ||
| *'''Dominio:''' <math>\mathbb{R}</math> | *'''Dominio:''' <math>\mathbb{R}</math> | ||
| Línea 49: | Línea 49: | ||
| *'''Mínimos:''' <math>\left \{ x=\pi (2k+1) \, , \quad k \in \mathbb{Z} \right \}</math> | *'''Mínimos:''' <math>\left \{ x=\pi (2k+1) \, , \quad k \in \mathbb{Z} \right \}</math> | ||
| *'''Crecimiento:''' | *'''Crecimiento:''' | ||
| - | **Crece en <math>\big( 3 \pi / 2+2 \pi k , \, \pi /2 +2 \pi k \big), \quad k \in \mathbb{Z}</math>. | + | **Crece en <math>\big( \pi (2k-1) , \, 2 \pi k \big), \quad k \in \mathbb{Z}</math>. |
| - | **Decrece en <math>\big( \pi / 2+2 \pi k , \, 3 \pi /2 +2 \pi k \big), \quad k \in \mathbb{Z}</math>. | + | **Decrece en <math>\big( 2 \pi k , \, \pi (2k+1) \big), \quad k \in \mathbb{Z}</math>. |
| }} | }} | ||
| }} | }} | ||
| ==Función tangente== | ==Función tangente== | ||
| + | {{Tabla50|celda2=<center>'''Función tangente''' (cosinusoide).{{p}}[[Imagen:funcioncoseno.jpg|380px|]]<br>Los valores en el eje x están expresados en múltiplos de <math> \pi </math> rad</center> | ||
| + | |celda1= | ||
| + | {{Caja_Amarilla|texto=Se define la función '''coseno''' como | ||
| + | |||
| + | <center><math>f(x)=tg(x) \, , \quad x \in \mathbb{R}-\left \{ \pi /2 + k \pi \quad k \in \mathbb{Z} \right \}</math></center> | ||
| + | }} | ||
| + | ===Propiedades de la función tangente=== | ||
| + | {{Caja_Amarilla|texto= | ||
| + | *'''Dominio:''' <math>\mathbb{R}-\left \{ \pi /2 + k \pi \quad k \in \mathbb{Z} \right \}</math> | ||
| + | *'''Recorrido:''' <math>\mathbb{R}</math> | ||
| + | *'''Periodicidad:''' Es periódica, con período <math>\pi \,</math>. | ||
| + | *'''Continuidad:''' Es continua en su dominio. Tiene discontinuidades infinitas en <math>\left \{ x=\pi /2 + k \pi \quad k \in \mathbb{Z} \right \}</math> | ||
| + | *'''Simetrías:''' Es impar, pués <math>tg(-x)=-tg(x)\,</math> | ||
| + | *'''Cortes con eje X:''' <math>\left \{ x=k \pi , \quad k \in \mathbb{Z} \right \}</math> | ||
| + | *'''Máximos:''' No tiene | ||
| + | *'''Mínimos:''' No tiene | ||
| + | *'''Crecimiento:''' Creciente en su dominio. | ||
| + | }} | ||
| + | }} | ||
| [[Imagen:funciontg.jpg|380px|thumb|Función tangente. Los valores en el eje x están expresados en múltiplos de <math> \pi </math> rad]] | [[Imagen:funciontg.jpg|380px|thumb|Función tangente. Los valores en el eje x están expresados en múltiplos de <math> \pi </math> rad]] | ||
| ==Videos== | ==Videos== | ||
Revisión de 10:19 3 mar 2009
Menú:
| Enlaces internos | Para repasar o ampliar | Enlaces externos |
| Indice Descartes Manual Casio | WIRIS Geogebra Calculadoras |
Vamos a estudiar las funciones que se obtienen a partir de las razones trigonométricas de un ángulo x al hacer variar éste. Dicho ángulo se suele expresar en radianes.
Tabla de contenidos |
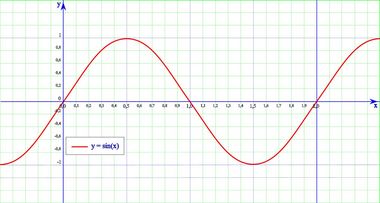
Función seno
Función coseno
Función tangente
Videos
Video tutorial de matematicasbachiller.com
Video tutorial de matematicasbachiller.com
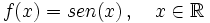
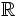
![[-1, 1]\,](/wikipedia/images/math/5/8/1/581a26dec23e9f937a02a278e20fc9c3.png)
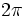 .
.
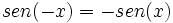
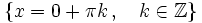
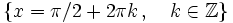
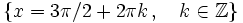
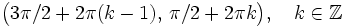 .
.
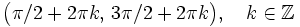 .
.
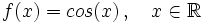

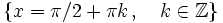
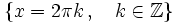
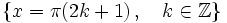
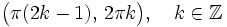 .
.
 .
.

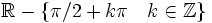
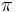 .
.