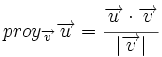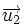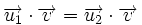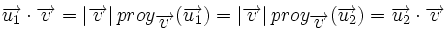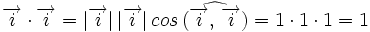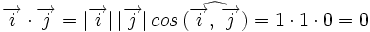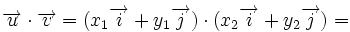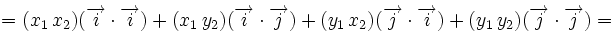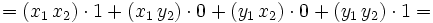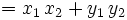Vectores: Producto escalar (1ºBach)
De Wikipedia
| Revisión de 18:12 15 mar 2009 Coordinador (Discusión | contribuciones) (→Perpendicularidad y producto escalar) ← Ir a diferencia anterior |
Revisión de 21:45 15 mar 2009 Coordinador (Discusión | contribuciones) (→Propiedad fundamental del producto escalar) Ir a siguiente diferencia → |
||
| Línea 16: | Línea 16: | ||
| ===Propiedad fundamental del producto escalar=== | ===Propiedad fundamental del producto escalar=== | ||
| {{Teorema||titulo=Propiedad fundamental|enunciado= | {{Teorema||titulo=Propiedad fundamental|enunciado= | ||
| - | *'''Nulidad:''' Si cualquiera de los dos vectores, {{sube|porcentaje=+30%|contenido=<math>\overrightarrow{u}</math>}} o {{sube|porcentaje=+30%|contenido=<math>\overrightarrow{v}</math>}}, es {{sube|porcentaje=+30%|contenido=<math>\overrightarrow{0}</math>}}, entonces {{sube|porcentaje=+30%|contenido=<math>\overrightarrow{u} \cdot \overrightarrow{v}=0</math>}}. | + | *Si cualquiera de los dos vectores, {{sube|porcentaje=+30%|contenido=<math>\overrightarrow{u}</math>}} o {{sube|porcentaje=+30%|contenido=<math>\overrightarrow{v}</math>}}, es {{sube|porcentaje=+30%|contenido=<math>\overrightarrow{0}</math>}}, entonces {{sube|porcentaje=+30%|contenido=<math>\overrightarrow{u} \cdot \overrightarrow{v}=0</math>}}. |
| - | *'''Perpendicularidad''' Dados dos vectores no nulos, {{sube|porcentaje=+30%|contenido=<math>\overrightarrow{u}</math>}} y {{sube|porcentaje=+30%|contenido=<math>\overrightarrow{v}</math>}}, se cumple que | + | *Dados dos vectores no nulos, {{sube|porcentaje=+30%|contenido=<math>\overrightarrow{u}</math>}} y {{sube|porcentaje=+30%|contenido=<math>\overrightarrow{v}</math>}}, se cumple que |
| <center><math>\overrightarrow{u} \bot \overrightarrow{v} \iff \overrightarrow{u} \cdot \overrightarrow{v}=0</math></center> | <center><math>\overrightarrow{u} \bot \overrightarrow{v} \iff \overrightarrow{u} \cdot \overrightarrow{v}=0</math></center> | ||
| |demo= | |demo= | ||
| - | *'''Nulidad:''' Esta propiedad es inmediata. | + | *La primera propiedad es inmediata. |
| - | *'''Perpendicularidad''' Si ambos vectores no son nulos, entonces, para que le productro escalar sea cero, debe ser cero el coseno del ángulo que forman esto ocurre sólo si el ángulo es de 90º. | + | *Para la segunda propiedad, si ambos vectores no son nulos, entonces, para que el producto escalar sea cero, debe ser cero el coseno del ángulo que forman, y esto ocurre sólo si el ángulo es de 90º. |
| }} | }} | ||
| {{p}} | {{p}} | ||
Revisión de 21:45 15 mar 2009
| Enlaces internos | Para repasar o ampliar | Enlaces externos |
| Indice Descartes Manual Casio | Act. Interactivas | WIRIS Geogebra Calculadoras |
Tabla de contenidos |
Producto escalar de vectores
Se llama producto escalar de dos vectores  y
y 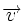 , al número real que se obtiene multiplicando los módulos de ambos vectores por el coseno del ángulo que forman:
, al número real que se obtiene multiplicando los módulos de ambos vectores por el coseno del ángulo que forman:
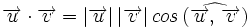
Propiedades del producto escalar
Propiedad fundamental del producto escalar
Propiedad fundamental
- Si cualquiera de los dos vectores,
 o
o 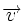 , es
, es 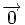 , entonces
, entonces 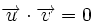 .
.
- Dados dos vectores no nulos,
 y
y 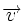 , se cumple que
, se cumple que
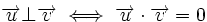
- La primera propiedad es inmediata.
- Para la segunda propiedad, si ambos vectores no son nulos, entonces, para que el producto escalar sea cero, debe ser cero el coseno del ángulo que forman, y esto ocurre sólo si el ángulo es de 90º.
Signo del producto escalar
Propiedades: signo del producto escalar
El signo del producto escalar queda determinado por el ángulo que forman los vectores:
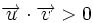 si
si  es agudo.
es agudo.
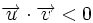 si
si 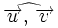 es obtuso.
es obtuso.
Esta propiedad es inmediata, ya que el coseno de un ángulo es positivo, si éste es agudo, y negativo, si es obtuso.
Operaciones con el producto escalar
Propiedades de las operaciones
- Propiedad conmutativa:
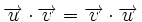 .
.
- Propiedad asociativa:
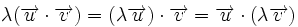 .
.
- Propiedad distributiva:
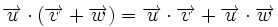 .
.
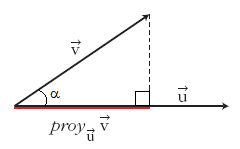
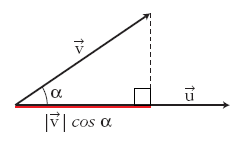
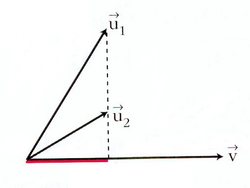
Proyección de vectores y producto escalar
Llamaremos proyección del vector 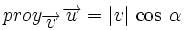 siendo Observa que la proyección es un número positivo o negativo según lo sea |
El producto escalar con bases ortonormales
Expresión analítica del producto escalar en bases ortonormales
Proposición
Sea 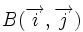 una base ortonormal, entonces
una base ortonormal, entonces
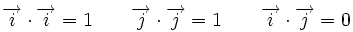
En efecto, al ser los vectores de la base unitarios y perpendiculares, tenemos:
Proposición
Si las coordenadas de los vectores  y
y 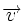 , respecto de una base otonormal
, respecto de una base otonormal 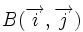 son
son  y
y 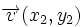 , entonces:
, entonces:
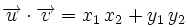
En efecto, al ser los vectores de la base unitarios y perpendiculares, tenemos:
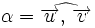
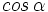 .
.

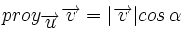
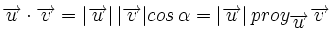
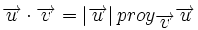 </center>
</center>