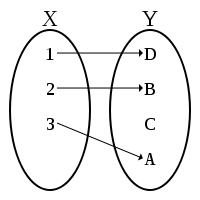
Función inyectiva
De Wikipedia
(Diferencia entre revisiones)
| Revisión de 17:20 24 ene 2009 Coordinador (Discusión | contribuciones) ← Ir a diferencia anterior |
Revisión actual Coordinador (Discusión | contribuciones) |
||
| Línea 1: | Línea 1: | ||
| - | {{Tabla75|celda2=<center>[[Image:Injection.svg|frame|right|Ejemplo de función inyectiva.]]</center> | + | {{Tabla75|celda2=<center>[[Image:Injection.png|frame|right|Ejemplo de función inyectiva.]]</center> |
| |celda1= | |celda1= | ||
| {{Caja_Amarilla | {{Caja_Amarilla | ||
| - | |texto=Una función <math>f:\mathbb{R}\to\mathbb{R}</math>, dada por <math>f \colon X \to Y \,</math> es '''inyectiva''' o '''uno a uno''' si cada valor en la imagen de <math>f\,</math> se corresponde con un único origen en el dominio. Simbólicamente: | + | |texto=Una función <math>f \colon X \to Y \,</math> es '''inyectiva''' o '''uno a uno''' si cada valor en la imagen de <math>f\,</math> se corresponde con un único valor de <math>X\;</math>. Simbólicamente: |
| {{p}} | {{p}} | ||
| <center><math>\forall x_1,x_2 \in X \ / \ f(x_1)=f(x_2) \ \Rightarrow \ x_1=x_2</math></center> | <center><math>\forall x_1,x_2 \in X \ / \ f(x_1)=f(x_2) \ \Rightarrow \ x_1=x_2</math></center> | ||
| }} | }} | ||
| {{p}} | {{p}} | ||
| - | {{Desplegable|titulo=Ejemplos:{{b}}|contenido= | + | {{Ejemplo|titulo=Ejemplo: ''Función inyectiva'' |
| - | *La función <math>f(x)=x^2\,</math> no es inyectiva, puesto que <math>f(2)=f(-2)=4\;</math>. | + | |enunciado= |
| - | *Pero si el dominio se restringe a los números positivos, obteniendo así una nueva función <math>g:\mathbb{R}^+\to\mathbb{R}^+</math> entonces sí se obtiene una función inyectiva. | + | :a) La función <math>f:\mathbb{R}\to\mathbb{R}</math>, dada por <math>f(x)=x^2\,</math> no es inyectiva |
| + | :b) Pero si el dominio se restringe a los números positivos, obteniendo así una nueva función <math>g:\mathbb{R}^+\to\mathbb{R}^+</math> entonces sí se obtiene una función inyectiva. | ||
| + | |sol= | ||
| + | a) En efecto, la función no es inyectiva puesto que <math>f(2)=f(-2)=4\;</math>. Es decir, hay dos valores del dominio, 2 y -2, cuya imagen coincide (4). | ||
| + | |||
| + | ---- | ||
| + | b) Al restringir el dominio a números positivos, dado cualquier valor de la imagen , solo existe un valor del dominio, su raíz cuadrada positiva, que se corresponde con él. | ||
| }} | }} | ||
| }} | }} | ||
| + | |||
| + | [[Categoría: Matemáticas]][[Categoría: Funciones]] | ||
Revisión actual
Una función 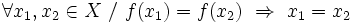  Ejemplo: Función inyectiva
Solución: a) En efecto, la función no es inyectiva puesto que b) Al restringir el dominio a números positivos, dado cualquier valor de la imagen , solo existe un valor del dominio, su raíz cuadrada positiva, que se corresponde con él. |  Ejemplo de función inyectiva. |
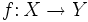es inyectiva o uno a uno si cada valor en la imagen de 
se corresponde con un único valor de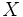 
. Simbólicamente:
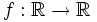, dada por 
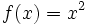no es inyectiva
entonces sí se obtiene una función inyectiva.
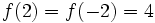. Es decir, hay dos valores del dominio, 2 y -2, cuya imagen coincide (4).
