Función inversa o recíproca (1ºBach)
De Wikipedia
| Revisión de 19:11 30 ene 2009 Coordinador (Discusión | contribuciones) (→Videos sobre funciones inversas) ← Ir a diferencia anterior |
Revisión actual Coordinador (Discusión | contribuciones) (→Inversas de las funciones trigonométricas) |
||
| Línea 6: | Línea 6: | ||
| }} | }} | ||
| {{p}} | {{p}} | ||
| - | ==Función inversa o recíproca== | + | __TOC__ |
| - | {{Tabla75|celda2= | + | |
| {{p}} | {{p}} | ||
| - | + | (Pág. 259) | |
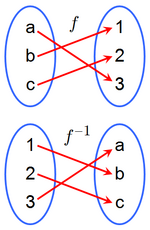
| - | <center>[[Imagen:Inverse Function.png|thumb|150px|Una función ƒ y su inversa o recíproca ƒ<sup> –1</sup>. Como ƒ aplica ''a'' en 3, la inversa ƒ<sup> –1</sup> lleva 3 de vuelta en ''a''.]]</center> | + | |
| - | |celda1= | + | |
| - | Si <math>f\;</math> es una función que lleva elementos de <math>X\;</math> en elementos de <math>Y\;</math>, en ciertas condiciones será posible definir la aplicación <math>f^{-1}\;</math> que realice el camino de vuelta de <math>Y\;</math> a <math>X\;</math>. En ese caso diremos que <math>f^{-1}\;</math> es la función '''inversa''' o '''recíproca''' de <math>f\;</math>. Formalmente: | + | |
| {{p}} | {{p}} | ||
| - | {{Caja_Amarilla | + | {{Función inversa (1ºBach)}} |
| - | |texto= | + | ==Ejercicios propuestos== |
| - | Sea <math>f\;</math> una función real [[Función inyectiva|inyectiva]], cuyo dominio sea el conjunto <math>X\;</math> y cuya imagen sea el conjunto <math>Y\;</math>. Entonces, la '''función recíproca o inversa''' de <math>f\;</math>, denotada <math>f^{-1}\;</math>, es la función de dominio <math>Y\;</math> e imagen <math>X\;</math> definida por la siguiente regla: | + | {{ejercicio |
| + | |titulo=Ejercicios propuestos: ''Función inversa o recíproca'' | ||
| + | |cuerpo= | ||
| + | (Pág. 259-260) | ||
| - | <center><math>f(x) = y\Leftrightarrow{}f^{-1}(y) = x \,\!</math></center> | + | [[Imagen:red_star.png|12px]] 1 al 6 |
| }} | }} | ||
| {{p}} | {{p}} | ||
| - | {{Teorema|titulo=Propiedades | ||
| - | |enunciado=Sea <math>f \colon X \rightarrow Y</math> una función y <math>f^{-1}\;</math> su inversa: | ||
| - | {{p}} | ||
| - | *Las gráficas de <math>f\;</math> y <math>f^{-1}\;</math> son simétricas respecto de la recta <math>y=x\;</math>. | ||
| - | *La función <math>f^{-1}\;</math>, al igual que <math>f\;</math>, es una función [[Función biyectiva|biyectiva]], que queda determinada de modo único por <math>f\;</math> y que cumple: | ||
| - | :a) <math>f^{-1} \circ f = I_X</math> | ||
| - | :b) <math>f \circ f^{-1}=I_Y</math> | ||
| - | |||
| - | donde <math>I_X\;</math> e <math>I_Y\;</math> son las [[Función identidad|funciones identidad]] en <math>X\;</math> e <math>Y\;</math> respectivamente. | ||
| - | |demo= | ||
| - | }} | ||
| - | }} | ||
| - | {{p}} | ||
| - | {{AI2|titulo=Actividad Interactiva: ''Función inversa''|cuerpo= | ||
| - | {{ai_cuerpo | ||
| - | |enunciado='''Actividad 1.''' Representación gráfica de una función <math>f(x)\;</math> y de su inversa <math>f^{-1}(x)\;</math>. | ||
| - | |actividad= | ||
| - | En esta escena tienes la gráfica de la función <math>f(x) = x^3\;</math> (en verde) y la de <math>f^{-1}(x)=\sqrt[3]{x}</math> (en amarillo). Observa que son simétricas respecto de la bisectriz del primer cuadrante, la recta <math>y=x\;</math> (en rojo). | ||
| - | |||
| - | {{p}} | ||
| - | <center><iframe> | ||
| - | url=http://maralboran.org/web_ma/descartes/Analisis/El_pinta_graficas/grafic_4f.html | ||
| - | width=450 | ||
| - | height=380 | ||
| - | name=myframe | ||
| - | </iframe></center> | ||
| - | <center>[http://maralboran.org/web_ma/descartes/Analisis/El_pinta_graficas/grafic_4f.html '''Click''' aquí si no se ve bien la escena]</center> | ||
| - | |||
| - | Prueba a cambiar también la función <math>f(x)=x^3\;</math> por otras funciones, por ejemplo, <math>f(x)=x^2\;</math>. ¿Quien sería su función inversa?. ¿Que ocurre?. Recuerda que para que una función tenga inversa debe ser [[Función inyectiva |inyectiva]]. | ||
| - | |||
| - | No olvides pulsar "Intro" al cambiar cada función. | ||
| - | }} | ||
| - | }} | ||
| - | {{p}} | ||
| - | {{Ejemplo|titulo=Ejemplo: ''Función inversa'' | ||
| - | |enunciado=Halla la función inversa de la función <math>f:\mathbb{R}\to\mathbb{R}</math> definida por <math>f(x)=x^2\;</math>: | ||
| - | |sol= | ||
| - | Como la función <math>f(x)=x^2\;</math> no es inyectiva, no podemos calcular su inversa. No obstante, podemos descomponerla en dos trozos que si sean funciones inyectivas por separado y alos que si podamos calcular su inversa: | ||
| - | |||
| - | <center><math>f(x)=x^2= | ||
| - | \begin{cases} | ||
| - | f_1(x)=x^2 \ , & si \ x \ge 0 \rightarrow f_1^{-1}(x)=\sqrt{x} | ||
| - | \\ | ||
| - | f_2(x)=x^2 \ , & si \ x < 0 \rightarrow f_2^{-1}(x)=-\sqrt{x} | ||
| - | \end{cases} | ||
| - | </math></center> | ||
| - | |||
| - | |||
| - | En la siguiente escena puedes ver <math>f(x)=x^2\;</math> (en verde), <math>f_1^{-1}(x)=\sqrt{x}</math> (en amarillo), y <math>f_2^{-1}(x)=-\sqrt{x}</math> (en turquesa): | ||
| - | |||
| - | |||
| - | <center><iframe> | ||
| - | url=http://maralboran.org/web_ma/descartes/Analisis/El_pinta_graficas/grafic_4g.html | ||
| - | width=450 | ||
| - | height=380 | ||
| - | name=myframe | ||
| - | </iframe></center> | ||
| - | <center>[http://maralboran.org/web_ma/descartes/Analisis/El_pinta_graficas/grafic_4g.html '''Click''' aquí si no se ve bien la escena]</center> | ||
| - | }} | ||
| - | {{p}} | ||
| - | |||
| - | ==Videos sobre funciones inversas== | ||
| - | {{p}} | ||
| - | {{Video_enlace2 | ||
| - | |titulo1=Función inversa o recíproca | ||
| - | |duracion= | ||
| - | |sinopsis=Video tutorial de matematicasbachiller.com | ||
| - | |url1=http://www.matematicasbachiller.com/videos/cdiferencial/01/di01_42.html | ||
| - | }} | ||
| - | {{p}} | ||
| - | {{ejemplo2 | ||
| - | |titulo=[[Imagen:Video.gif|30px]] Video-Ejemplos: ''Función inversa o recíproca'' | ||
| - | |enunciado= | ||
| - | {{Video_enlace2 | ||
| - | |titulo1=1. Ejemplos | ||
| - | |duracion='" | ||
| - | |sinopsis=Video tutorial de matematicasbachiller.com | ||
| - | |url1=http://www.matematicasbachiller.com/videos/cdiferencial/01/di01_42_01.html | ||
| - | }} | ||
| - | {{Video_enlace2 | ||
| - | |titulo1=1. Ejemplos | ||
| - | |duracion='" | ||
| - | |sinopsis=Video tutorial de matematicasbachiller.com | ||
| - | |url1=http://www.matematicasbachiller.com/videos/cdiferencial/01/di01_42_01.html | ||
| - | }} | ||
| - | {{Video_enlace2 | ||
| - | |titulo1=2. Ejemplos | ||
| - | |duracion='" | ||
| - | |sinopsis=Video tutorial de matematicasbachiller.com | ||
| - | |url1=http://www.matematicasbachiller.com/videos/cdiferencial/01/di01_42_02.html | ||
| - | }} | ||
| - | {{Video_enlace2 | ||
| - | |titulo1=3. Ejemplos | ||
| - | |duracion='" | ||
| - | |sinopsis=Video tutorial de matematicasbachiller.com | ||
| - | |url1=http://www.matematicasbachiller.com/videos/cdiferencial/01/di01_42_03.html | ||
| - | }} | ||
| - | {{Video_enlace2 | ||
| - | |titulo1=4. Ejemplos | ||
| - | |duracion='" | ||
| - | |sinopsis=Video tutorial de matematicasbachiller.com | ||
| - | |url1=http://www.matematicasbachiller.com/videos/cdiferencial/01/di01_42_04.html | ||
| - | }} | ||
| - | {{Video_enlace2 | ||
| - | |titulo1=5. Ejemplos | ||
| - | |duracion='" | ||
| - | |sinopsis=Video tutorial de matematicasbachiller.com | ||
| - | |url1=http://www.matematicasbachiller.com/videos/cdiferencial/01/di01_42_05.html | ||
| - | }} | ||
| - | }} | ||
| - | {{p}} | ||
| - | {{Video_enlace2 | ||
| - | |titulo1=Ojo con la notación de las funciones inversas | ||
| - | |duracion= | ||
| - | |sinopsis=Video tutorial de matematicasbachiller.com | ||
| - | |url1=http://www.matematicasbachiller.com/videos/cdiferencial/01/di01_43.html | ||
| - | }} | ||
| - | |||
| [[Categoría: Matemáticas]][[Categoría: Funciones]] | [[Categoría: Matemáticas]][[Categoría: Funciones]] | ||
Revisión actual
| Enlaces internos | Para repasar o ampliar | Enlaces externos |
| Indice Descartes Manual Casio | WIRIS Geogebra Calculadoras |
Tabla de contenidos |
(Pág. 259)
Función inversa o recíproca
Si  es una función que lleva elementos de es una función que lleva elementos de 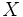 en elementos de en elementos de  , en ciertas condiciones será posible definir la aplicación , en ciertas condiciones será posible definir la aplicación 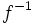 que realice el camino de vuelta de que realice el camino de vuelta de  a a 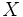 . En ese caso diremos que . En ese caso diremos que 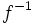 es la función inversa o recíproca de es la función inversa o recíproca de  . Formalmente: . Formalmente:
Introducción a las funciones inversas. Propiedades Sea
donde |
Obtención de la expresión analítica de la función inversa
Procedimiento
Para intentar hallar la expresión analítica de la inversa de y=f(x):
- Se despeja (si se puede) la variable "x" para ponerla en función de la variable "y".
- Se intercambian las dos incógnitas (donde aparece "x" se pone "y" y viceversa).
- La expresión resultante es la de la función inversa de f.
1 ejemplo sobre el cáculo de la función inversa y su interpretación gráfica.
2 ejemplos sobre el cáculo de la función inversa y su interpretación gráfica.
Algunos ejemplos sobre el cálculo de la función inversa y sobre la composición de funciones.
Obtención de la función inversa de 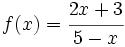 previa demostración de su inyectividad.
previa demostración de su inyectividad.
1 ejemplo sobre el cálculo de la función inversa de una función trigonométrica.
Ejemplo: Función inversa
Halla la función inversa de la función 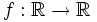 definida por
definida por 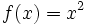 :
:
Como la función 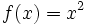 no es inyectiva, no podemos calcular su inversa. No obstante, podemos descomponerla en dos trozos que si sean funciones inyectivas por separado y a los que si podamos calcular su inversa:
no es inyectiva, no podemos calcular su inversa. No obstante, podemos descomponerla en dos trozos que si sean funciones inyectivas por separado y a los que si podamos calcular su inversa:
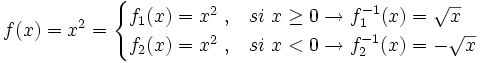
En la siguiente escena puedes ver 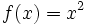 (en verde),
(en verde), 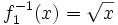 (en amarillo), y
(en amarillo), y 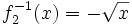 (en turquesa):
(en turquesa):
En esta escena podrás introducir la expresión analítica de una función y obtener la expresión analítica de su inversa, así como ver sus respectivas representaciones gráficas. También se te propondrán algunas actividades.
Ejemplo sobre el cálculo del rango o recorrido de una función mediante el cálculo del dominio de su función inversa.
Ejercicios propuestos
|
Ejercicios propuestos: Función inversa o recíproca |
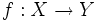 es
es 
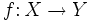 una función y
una función y 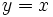 .
.


 e
e 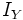 son las
son las